Understanding the catalytic role of oxalic acid in SO₃ hydration to form H₂SO₄ in the atmosphere

The hydration of SO₃ plays an important role in atmospheric sulfuric acid formation. Some atmospheric species can be involved in and facilitate the reaction. In this work, using quantum chemical calculations, we show that oxalic acid, the most common dicarboxylic acid in the atmosphere, can effectively catalyze the hydration of SO₃. The energy barrier of the SO₃ hydration reaction catalyzed by oxalic acid (cTt, tTt, tCt and cCt conformers) is a little higher or less than 1 kcal mol⁻¹, which is lower than the energy barrier of 5.17 kcal mol⁻¹ for water-catalyzed SO₃ hydration. Compared with the rates of the SO₃ hydration reaction catalyzed by oxalic acid and water, it can be found that in the upper troposphere the OA-catalyzed SO₃ hydration can play an important role in promoting SO₃ hydration. It leads us to conclude that the involvement of oxalic acid in SO₃ hydration to form H₂SO₄ is significant in the atmosphere.

1 Introduction

In the atmosphere, hydrogen atom transfer (HAT) reactions play a significant role in many processes. The radical reaction ($\mathrm{R}-\mathrm{H}+\mathrm{OH}\to \mathrm{R}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}$), as the most traditional HAT reaction, can be widely found in the atmosphere (Alvarez-Idaboy et al., 2001; Cameron et al., 2002; Steckler et al., 1997; Atkinson et al., 2006). The HAT process can also be found in some addition (Steudel, 1995; Williams et al., 1983; Courmier et al., 2005; Zhang and Zhang, 2002), decomposition (Rayez et al., 2002; Kumar and Francisco, 2015; Gutbrod et al., 1996), isomerization (Zheng and Truhlar, 2010; Atkinson, 2007) and abstraction reactions (Ji et al., 2013, 2017). These atmospheric HAT reactions display a main feature that the two-point hydrogen bond can occur and thus facilitates HAT (Kumar et al., 2016). Water molecules, acids and other catalysts, acting as hydrogen donors and acceptors, can contribute to the formation of two-point hydrogen bond (Vöhringer-Martinez et al., 2007; da Silva, 2010; Gonzalez et al., 2010; Bandyopadhyay et al., 2017). Thus, the effect of catalysts on promoting atmospheric HAT reactions has attracted more attention from atmospheric scientists.

The hydration of SO₃ to form sulfuric acid (H₂SO₄) is a typical addition reaction involving the HAT. In the atmosphere, this hydration reaction is regarded as the main source of gas-phase sulfuric acid. For the reaction SO₃ + H₂O → H₂SO₄, the pre-reactive SO₃… H₂O complex is firstly formed, and the complex is then rearranged to produce H₂SO₄ (Holland and Castleman, 1978; Hofmann-Sievert and Castleman, 1984). But subsequent research found that this hydration reaction involving a single water molecule cannot take place in the atmosphere due to its high energy barrier (Hofmann and Schleyer, 1994; Morokuma and Muguruma, 1994; Steudel, 1995). The inclusion of a second water molecule in the above reaction has been proven to significantly reduce the hydration energy barrier (Morokuma and Muguruma, 1994; Loerting and Liedl, 2000; Larson et al., 2000). The promoting effect can be mainly attributed to the formation of the two-point hydrogen bond, which reduces the ring strain occurring in the pre-reactive complex, and facilitates the rearrangement of the pre-reactive complex via double HAT. It has also been shown that some other atmospheric molecules can serve as a catalyst to promote the hydration of SO₃. To the best of our knowledge, the hydroperoxy radical (Gonzalez et al., 2010), formic acid (Hazra and Sinha, 2011; Long et al., 2012), sulfuric acid (Torrent-Sucarrat et al., 2012), nitric acid (Long et al., 2013) and ammonia (Bandyopadhyay et al., 2017) have been reported to replace the second water molecule to catalyze the hydration reaction of SO₃.

Oxalic acid (OA), the most prevalent dicarboxylic acid in the atmosphere (Ho et al., 2015; Kawamura and Ikushima, 1993), is a water-soluble organic acid, so it has a high concentration in aerosols (Kawamura et al., 2013; van Pinxteren et al., 2014; Deshmukh et al., 2016; Wang et al., 2016). In addition to its accumulation in aerosols, OA, as an organic acid in the gas phase, has been found to enhance new particle formation (NPF; Xu et al., 2010, 2017; Weber et al., 2012, 2014; Xu and Zhang, 2012; Peng et al., 2015; Miao et al., 2015; Zhao et al., 2016; Chen et al., 2017; Arquero et al., 2017; Zhang, 2010). Theoretical studies about the effect of OA on atmospheric particle nucleation and growth have shown that it can generate stable complexes with water (Weber et al., 2012), sulfuric acid (Xu et al., 2010; Xu and Zhang, 2012; Miao et al., 2015; Zhao et al., 2016), ammonia (Weber et al., 2014; Peng et al., 2015) and amines (Chen et al., 2017; Xu et al., 2017; Arquero et al., 2017) via an intermolecular hydrogen bond. For OA, its potential to promote NPF is mainly attributed to its capability of forming hydrogen bonds with hydroxyl and/or carbonyl-type functional groups. Conversely to monocarboxylic acids, dicarboxylic acids such as OA have been proved to enhance nucleation in two directions because of its two acid moieties (Xu and Zhang, 2012). Thus, it can be believed that OA is a good candidate for catalyzing the HAT reaction in the atmosphere.

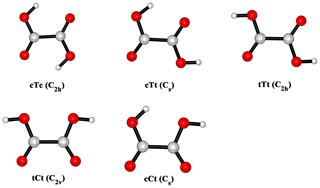
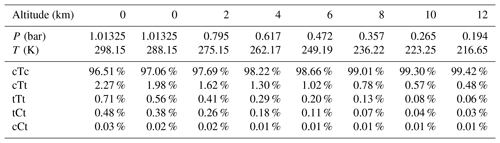
In this paper, we report the hydration reaction of SO₃ in the presence of OA, aiming to study the catalytic effect and importance of OA in the hydration of SO₃. It is known that OA can exist in several conformational forms (Buemi, 2009), which can be identified through the nomenclature used by Nieminen et al. (1992). Thus, five stable conformers of OA were considered in this work. The rate constants of OA-catalyzed SO₃ hydration were calculated using kinetic analysis, and compared with that of the water-catalyzed hydration reaction. Finally, combining concentrations of reactants with the rate constants, we evaluated the importance of the hydration process involving the OA relative to the hydration of SO₃ with the second water molecule as a catalyst to form sulfuric acid.

2 Computational details

The Gaussian 09 software suite (Frisch et al., 2010) was used in this work to perform all electronic structure calculations. The geometric structures – including all reactants, complexes, transition states and products – were optimized using the M06-2X method (Zhao and Truhlar, 2008) with 6-311++G(3df,3pd) basis set. For the M06-2X method, the ultrafine integration grid was chosen to enhance calculation accuracy at reasonable additional cost. The frequency calculations were carried out at the same level after geometric optimization to verify the local minimum points and transition states through the criterion that there are no imaginary frequencies for the local minimum point and one imaginary frequency for transition states. According to frequency calculations, the zero-point energies (ZPEs) and thermal corrections can also be obtained. The intrinsic reaction coordinate (IRC) calculation (Fukui, 1981; Hratchian and Schlegel, 2004; Hratchian and Schlegel, 2005) was performed to ensure that the transition states are connected with the corresponding reactants and products. A high level ab initio method, CCSD(T) method (Purvis and Bartlett, 1982; Pople et al., 1987), with the cc-pV(T+d)Z basis set was used to refine the single-point energies of these optimized species. To obtain a more accurate conformational population of OA at different temperatures, the quantum chemistry composite method, Gaussian 4 (G4) theory (Curtiss et al., 2007), was also performed for OA conformers.

In the kinetic analysis, the electronic energies were based on the CCSD(T)/cc-pV(T+d)Z level of theory, while the partition functions were obtained from the M06-2X/6-311++G(3df,3pd) level of theory. The rate constants for the rearrangement process of the SO₃ hydration reaction was estimated using conventional transition-state theory (TST; Truhlar et al., 1996) with Wigner tunneling correction. All kinetic analyses were executed in the KiSThelP program (Canneaux et al., 2014). The kinetic analyses are summarized as follows.

Based on the discussion in this paper, it can be concluded that SO₃ hydration reactions begin with the formation of a pre-reactive complex, and then pass by a transition state to form a post-reactive complex. This process can be characterized by the following reaction:

$$\begin{array}{}\text{(R1)}& {\displaystyle }& {\displaystyle }A+B\underset{k_{-\mathrm{1}}}{\stackrel{k_{\mathrm{1}}}{\mathit{\leftrightarrows }}}C,\text{(R2)}& {\displaystyle }& {\displaystyle }C+D\underset{k_{-\mathrm{2}}}{\stackrel{k_{\mathrm{2}}}{\mathit{\leftrightarrows }}}\mathrm{pre}\text{-}\mathrm{reactive}\mathrm{complex}\stackrel{k_{\mathrm{uni}}}{⟶}\mathrm{post}\text{-}\mathrm{reactive}\mathrm{complex}.\end{array}$$

Assuming that the complexes are in equilibrium with their reactants and that the steady-state approximation is applied to the pre-reactive complex, the reaction rate can be formulated as

$$\begin{array}{}\text{(1)}& {\displaystyle }v={\displaystyle \frac{k_{\mathrm{1}}}{k_{-\mathrm{1}}}}{\displaystyle \frac{k_{\mathrm{2}}}{k_{-\mathrm{2}}}}{k}_{\mathrm{uni}}\left[A\right]\left[B\right]\left[D\right]=K_{\mathrm{eq}\mathrm{1}}{K}_{\mathrm{eq}\mathrm{2}}{k}_{\mathrm{uni}}\left[A\right]\left[B\right]\left[D\right],\end{array}$$

where K_eq1 and K_eq2 are the equilibrium constants, and k_uni is the rate constant for a unimolecular reaction from the pre-reactive complex to post-reactive complex.

In the water-catalyzed hydration process, two channels can be written as

$$\begin{array}{ll}\text{(R3)}& {\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\underset{k_{-\mathrm{1}}}{\stackrel{k_{\mathrm{1}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}{\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\underset{k_{-\mathrm{2}}^{w\mathrm{1}}}{\stackrel{k_{\mathrm{2}}^{w\mathrm{1}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\\ {\displaystyle }& {\displaystyle }\stackrel{k_{\mathrm{uni}\mathit{\_}w}}{⟶}{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O},\\ \text{(R4)}& {\displaystyle }& {\displaystyle }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\underset{k_{-\mathrm{1}}^{w\mathrm{2}}}{\stackrel{k_{\mathrm{1}}^{w\mathrm{2}}}{\mathit{\leftrightarrows }}}{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}{\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\underset{k_{-\mathrm{2}}^{w\mathrm{2}}}{\stackrel{k_{\mathrm{2}}^{w\mathrm{2}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\\ {\displaystyle }& {\displaystyle }\stackrel{k_{\mathrm{uni}\mathit{\_}w}}{⟶}{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}.\end{array}$$

The corresponding rates are

$$\begin{array}{ll}\text{(2)}& {\displaystyle }{v}_{w\mathrm{1}}& {\displaystyle }={\displaystyle \frac{k_{\mathrm{1}}}{k_{-\mathrm{1}}}}{\displaystyle \frac{k_{\mathrm{2}}^{w\mathrm{1}}}{k_{-\mathrm{2}}^{w\mathrm{1}}}}{k}_{\mathrm{uni}\mathit{\_}w}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]{\displaystyle }& {\displaystyle }=K_{\mathrm{eq}\mathrm{1}}{K}_{\mathrm{eq}\mathrm{2}}^{w\mathrm{1}}{k}_{\mathrm{uni}\mathit{\_}w}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\\ {\displaystyle }& {\displaystyle }=k_{w\mathrm{1}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right],\\ \text{(3)}& {\displaystyle }{v}_{w\mathrm{2}}& {\displaystyle }={\displaystyle \frac{k_{\mathrm{1}}^{w\mathrm{2}}}{k_{-\mathrm{1}}^{w\mathrm{2}}}}{\displaystyle \frac{k_{\mathrm{2}}^{w\mathrm{2}}}{k_{-\mathrm{2}}^{w\mathrm{2}}}}{k}_{\mathrm{uni}\mathit{\_}w}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]{\displaystyle }& {\displaystyle }=K_{\mathrm{eq}\mathrm{1}}^{w\mathrm{2}}{K}_{\mathrm{eq}\mathrm{2}}^{w\mathrm{2}}{k}_{\mathrm{uni}\mathit{\_}w}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\\ {\displaystyle }& {\displaystyle }=k_{w\mathrm{2}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right].\end{array}$$

For the OA-catalyzed hydration reaction of SO₃, there are two reaction channels and the same features as in the water-assisted hydration process. The two channels can be shown as follows:

$$\begin{array}{ll}\text{(R5)}& {\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\underset{k_{-\mathrm{1}}}{\stackrel{k_{\mathrm{1}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}{\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}+X\underset{k_{-\mathrm{2}}^{X\mathrm{1}}}{\stackrel{k_{\mathrm{2}}^{X\mathrm{1}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }X\stackrel{k_{\mathrm{uni}\mathit{\_}X}}{⟶}{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\mathrm{\cdots }{X}^{\prime },\\ \text{(R6)}& {\displaystyle }& {\displaystyle }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}+X\underset{k_{-\mathrm{1}}^{X\mathrm{2}}}{\stackrel{k_{\mathrm{1}}^{X\mathrm{2}}}{\mathit{\leftrightarrows }}}{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }X{\displaystyle }& {\displaystyle }{\mathrm{SO}}_{\mathrm{3}}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }X\underset{k_{-\mathrm{2}}^{X\mathrm{2}}}{\stackrel{k_{\mathrm{2}}^{X\mathrm{2}}}{\mathit{\leftrightarrows }}}{\mathrm{SO}}_{\mathrm{3}}\mathrm{\cdots }{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\mathrm{\cdots }X\stackrel{k_{\mathrm{uni}\mathit{\_}X}}{⟶}{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\mathrm{\cdots }{X}^{\prime }.\end{array}$$

Here, the symbol X in these two equations represents the different conformers of OA (namely, cTt, tTt, tCt and cCt conformer).

The corresponding rates of SO₃ hydration involving OA can be obtained from the following equations:

$$\begin{array}{ll}\text{(4)}& {\displaystyle }& {\displaystyle }{v}_{X\mathrm{1}}={\displaystyle \frac{k_{\mathrm{1}}}{k_{-\mathrm{1}}}}{\displaystyle \frac{k_{\mathrm{2}}^{X\mathrm{1}}}{k_{-\mathrm{2}}^{X\mathrm{1}}}}{k}_{\mathrm{uni}\mathit{\_}X}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]{\displaystyle }& {\displaystyle }=K_{\mathrm{eq}\mathrm{1}}{K}_{\mathrm{eq}\mathrm{2}}^{X\mathrm{1}}{k}_{\mathrm{uni}\mathit{\_}X}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]=k_{X\mathrm{1}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right],\\ \text{(5)}& {\displaystyle }& {\displaystyle }{v}_{X\mathrm{2}}={\displaystyle \frac{k_{\mathrm{1}}^{X\mathrm{2}}}{k_{-\mathrm{1}}^{X\mathrm{2}}}}{\displaystyle \frac{k_{\mathrm{2}}^{X\mathrm{2}}}{k_{-\mathrm{2}}^{X\mathrm{2}}}}{k}_{\mathrm{uni}\mathit{\_}X}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]{\displaystyle }& {\displaystyle }=K_{\mathrm{eq}\mathrm{1}}^{X\mathrm{2}}{K}_{\mathrm{eq}\mathrm{2}}^{X\mathrm{2}}{k}_{\mathrm{uni}\mathit{\_}X}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]=k_{X\mathrm{2}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right].\end{array}$$

To assess the importance of OA in SO₃ hydration to H₂SO₄ in the atmosphere, the relative rate can be used as

$$\begin{array}{ll}\text{(6)}& {\displaystyle \frac{v_{X\mathrm{1}}}{v_{w\mathrm{1}}}}& {\displaystyle }={\displaystyle \frac{k_{X\mathrm{1}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]}{k_{w\mathrm{1}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]}}{\displaystyle }& {\displaystyle }={\displaystyle \frac{k_{X\mathrm{1}}\left[X\right]}{k_{w\mathrm{1}}\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]}},\\ \text{(7)}& {\displaystyle \frac{v_{X\mathrm{2}}}{v_{w\mathrm{2}}}}& {\displaystyle }={\displaystyle \frac{k_{X\mathrm{2}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[X\right]}{k_{w\mathrm{2}}\left[{\mathrm{SO}}_{\mathrm{3}}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]}}{\displaystyle }& {\displaystyle }={\displaystyle \frac{k_{X\mathrm{2}}\left[X\right]}{k_{w\mathrm{2}}\left[{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right]}}.\end{array}$$

It can be easily inferred from these equations that rate constants are the same for Eqs. (2, k_w1) and (3, k_w2), as well as for Eqs. (4, k_X1) and (5, k_X2) (for proof, see Supplement S1). Thus, the relative rate values in Eqs. (6) and (7) are the same. On account of these reasons, we will only compare the relative rate ν_X1∕ν_w1 in this paper.

3 Results and discussion

3.1 Water-catalyzed hydration reaction of SO₃

Although the hydration of SO₃ involving two water molecules has been discussed many times, we still include it in our paper so as to compare it with the following SO₃ hydration reactions catalyzed by OA at the same theoretical level, i.e., at the CCSD(T)/cc-pV(T+d)Z//M06-2X/6-311++G(3df,3pd) level. For the reactants SO₃+2H₂O, the existence of two pathways has become a consensus. One is that the water dimer reacts with SO₃ to obtain the pre-reactive complex, then this complex is rearranged to form the H₂SO₄⋯H₂O complex (channel 1); the other begins with the reaction of the SO₃⋯H₂O complex with water, and the following reaction process is the same as channel 1 (channel 2). In the hydration process, the additional water molecule serves as a catalyst that can promote the reaction by making a bridge in the HAT from water to SO₃. The potential energy profile and geometric structures can be found in Fig. 1. Other results about the reaction in the Supplement in Table S1.

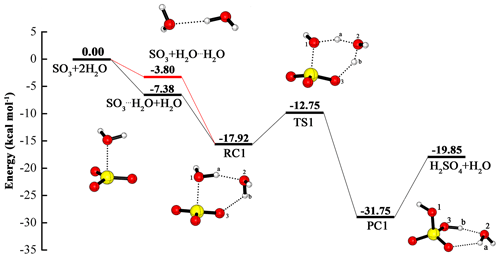
Figure 1 Calculated potential energy profile for the hydration of SO₃ with the second water molecule as a catalyst at the CCSD(T)/cc-pV(T+d)Z//M06-2X/6-311++G(3df,3pd) level.

From the two channels, it is clear that the binding energy of the water dimer is 3.80 kcal mol⁻¹, and that of the SO₃⋯H₂O complex is 7.38 kcal mol⁻¹. Moreover, the binding energy of the water dimer is consistent with its experimental value of 3.15±0.03 kcal mol⁻¹ (Rocher-Casterline et al., 2011), and with theoretical results of 2.90 (Torrent-Sucarrat et al., 2012), 2.97 (Long et al., 2013), 3.14 (Hazra and Sinha, 2011) and 3.30 kcal mol⁻¹ (Klopper et al., 2000). In the SO₃⋯H₂O complex, the binding energy of 7.38 kcal mol⁻¹ in our paper agrees with that from theoretical estimates in the literature: 7.60 (Torrent-Sucarrat et al., 2012), 7.42 (Long et al., 2013), 7.25 (Hazra and Sinha, 2011) and 7.77 kcal mol⁻¹ (Long et al., 2012). As shown in the Fig. 1, the two pathways involve the formation of the same pre-reactive complex (RC1) and the same subsequent processes. RC1 has a binding energy of 14.12 kcal mol⁻¹ relative to SO₃ + H₂O⋯H₂O, which is in accord with 13.60 (Torrent-Sucarrat et al., 2012) and 13.76 kcal mol⁻¹ (Long et al., 2013). The RC1 consists of a six-membered ring structure, in which two hydrogen bonds (also called a two-point hydrogen bond) between H_a and O₂, H_b and O₃, can be found. The formation of sulfuric acid from the RC1 needs to go through the rearrangement process with a transition state, which is a rate-limiting step with the energy barrier of 5.17 kcal mol⁻¹ with respect to RC1.

3.2 OA-catalyzed hydration reaction of SO₃

OA conformers, as shown in Fig. 2, are named according to the configurations of $\mathrm{H}-\mathrm{O}-\mathrm{C}-\mathrm{C}$ and $\mathrm{O}=\mathrm{C}-\mathrm{C}=\mathrm{O}$ dihedral angle: the lower-case letters refer to cis (c) or trans (t) configuration of $\mathrm{H}-\mathrm{O}-\mathrm{C}-\mathrm{C}$; the upper-case letters relate to cis (C) or tans (T) configuration of $\mathrm{O}=\mathrm{C}-\mathrm{C}=\mathrm{O}$. The nomenclature about conformers of OA is proposed by Nieminen et al. (1992).

Figure 2 Structures of oxalic acid (OA) conformers optimized at the M06-2X/6-311++G(3df,3pd) level.

The calculated potential energy profile for the OA-catalyzed hydration reaction of SO₃ is depicted in Fig. 3, and the corresponding geometry structures are shown in Fig. 4. Energies, enthalpies and free energies in all relevant species for the OA-catalyzed hydration of SO₃ are summarized in the Supplement in Tables S2–S5. From the two figures, it is obvious that the cTc conformer cannot act as a catalyst in the hydration reaction because the HAT cannot occur. The transfer failure is attributed to the fact that the hydrogen and oxygen atom involved in the two-point hydrogen bond do not come from the same carboxyl group (see Fig. 4). The hydration reactions catalyzed by the remaining four conformers exhibit the same feature (Fig. 3), i.e., the pre-reactive complex formed from the SO₃⋯H₂O complex with OA or the OA⋯H₂O complex with water can evolve into a product complex (H₂SO₄⋯OA complex) via the transition state of HAT. In this process, OA serves as a catalyst to promote the SO₃ hydration reaction by making a bridge when the hydrogen atom transfers from H₂O to SO₃. In the reaction catalyzed by the cTt conformer, the binding energy of the cTt⋯H₂O complex is 10.25 kcal mol⁻¹. The binding energy of the pre-reactive complex is 10.95 kcal mol⁻¹ compared to the cTt⋯H₂O complex with SO₃, while that relative to the SO₃⋯H₂O complex is 13.82 kcal mol⁻¹ with cTt. The transformation from RC_cTt to PC_cTt (PC refers to post-reactive complex) corresponds to the HAT process, and has a transition state (TS_cTt) with an energy barrier of 1.19 kcal mol⁻¹ with respect to RC_cTt. PC_cTt lies below the RC_cTt by 13.32 kcal mol⁻¹. It should be noted that the cTt conformer from RC_cTt has transformed to the cCt conformer in PC_cTt. The binding energy of PC_cTt is 17.78 kcal mol⁻¹ compared to H₂SO₄ and the cCt conformer.

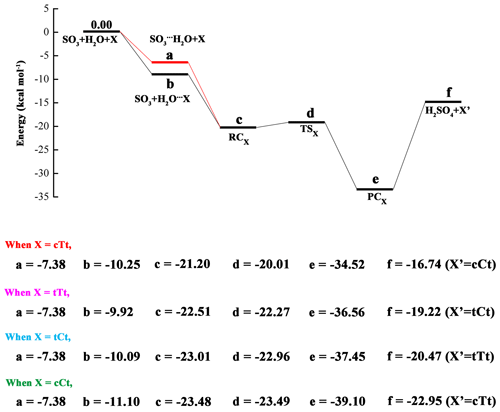
Figure 3 Calculated potential energy profile for the hydration of SO₃ with oxalic acid (OA) conformers (cTt, tTt, tCt and cCt) as catalysts at the CCSD(T)/cc-pV(T+d)Z//M06-2X/6-311++G(3df,3pd) level.

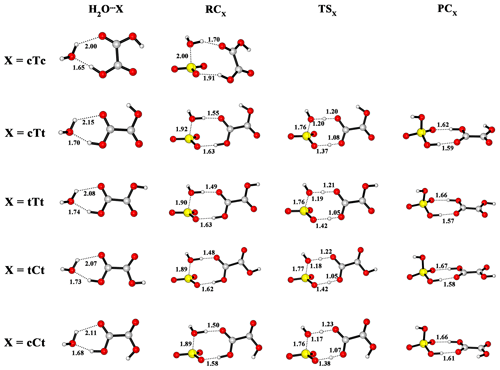
Figure 4 Optimized M06-2X/6-311++G(3df,3pd) structures of reactant complexes, pre-reactive complexes, transition states and post-reactive complexes for the OA-catalyzed SO₃ hydration reaction (distance unit: angstrom.

In hydration reactions involving the tTt conformer, the tTt⋯H₂O complex is stabilized by 9.92 kcal mol⁻¹, relative to tTt + H₂O. Starting with the tTt⋯H₂O + SO₃ channel, the pre-reactive complex (RC_tTt) can be formed with a binding energy of 12.59 kcal mol⁻¹, whereas the binding energy of RC_tTt is 15.13 kcal mol⁻¹ when it comes from the SO₃⋯H₂O + tTt channel. The TS_tTt lies above the RC_tTt by 0.24 kcal mol⁻¹. And the reaction proceeds with the formation of PC_tTt, which is more stable than RC_tTt (14.05 kcal mol⁻¹). The formed PC_tTt needs to obtain an energy of 17.34 kcal mol⁻¹ to disaggregate into H₂SO₄ and the tCt conformer.

For the tCt conformer, the binding energy of the tCt⋯H₂O complex is 10.09 kcal mol⁻¹. In the two channels, the same pre-reactive complex (RC_tCt) can be formed with a binding energy of 12.92 kcal mol⁻¹ relative to tCt⋯H₂O + SO₃, and 15.63 kcal mol⁻¹ with respect to SO₃⋯H₂O + tCt. The RC_tCt proceeds via the transition state (TS_tCt, with energy barrier of 0.05 kcal mol⁻¹) into the post-reactive complex (PC_tCt). The PC_tCt complex can also be generated from the H₂SO₄ and tTt conformer releasing 16.98 kcal mol⁻¹ of energy.

For the cCt conformer, the binding energy of the cCt⋯H₂O complex is 11.10 kcal mol⁻¹. Beginning with the cCt⋯H₂O + SO₃ and SO₃⋯H₂O + cCt channels, the hydration reactions undergo the same RC_cCt complex, which is more stable than the two reactants (by 12.38 kcal mol⁻¹, 16.10 kcal mol⁻¹, respectively), and a transition state (TS_cCt), and then produces the H₂SO₄⋯cTt complex (PC_cCt), which lies below the RC_cCt complex by 15.62 kcal mol⁻¹ and below H₂SO₄ + cTt by 16.15 kcal mol⁻¹. The pre-reactive complex (RC_cCt) is nearly iso-energetic with the transition state (TS_cCt).

In the light of the analysis above, it is obvious that the energy barrier of the hydration reaction catalyzed by OA is around 1.00 kcal mol⁻¹ for the cTt conformer, and approximates to 0.00 kcal mol⁻¹ for another three conformers. The result shows that OA is more effective than water in catalyzing the SO₃ hydration because the hydration reaction catalyzed by water has an energy barrier of 5.17 kcal mol⁻¹. Another point worth mentioning is that one OA conformer involved in SO₃ hydration transfers to another conformer type after the completion of hydration reaction (cTt → cCt, tTt → tCt, tCt → tTt, cCt → cTt).

In addition, compared to formic acid (Millet et al., 2015; Bannan et al., 2017), the SO₃ hydration reaction catalyzed by OA displays some specific characteristics. Both acids can obviously decrease the energy barrier of the hydration reaction. But because OA is a dicarboxylic acid, only one in the carboxylic groups participate in the hydration reaction and the other is free. This characteristic indicates that post-reactive complexes (PC_tTt and PC_tCt) can serve as a catalyst to continue to promote the SO₃ hydration. For these post-reactive complexes (PC_cTt, PC_tTt, PC_tCt and PC_cCt), the above result has shown that these complexes are stable compared to the isolated H₂SO₄ and OA conformers. The free carboxylic group in these complexes can also provide the interaction site when these complexes interact with other species. The free carboxylic group of these stable post-reactive complexes indicates that these complexes have the potential to participate in nucleation.

3.3 Atmospheric implications

To evaluate the importance of OA in enhancing H₂SO₄ formation in the atmosphere, we calculated the rate constants of SO₃ hydration catalyzed by OA and water, and compared the rate of OA-catalyzed hydration reactions with that of water-catalyzed hydration at different altitudes in the troposphere. The temperature, pressure, density of air and water vapor content at the corresponding altitude are taken from US Standard Atmosphere (1976, NASA and NOAA), and are shown in Table S6 in the Supplement.

According to these calculation methods, described in “Computational details” Sect. 2, it can be confirmed that both the ratio of rate constants and relative concentrations are important elements to estimate the effect of OA-catalyzed SO₃ hydration. Thus, the rate constants for hydration reactions of SO₃ catalyzed by OA and water at different altitudes are firstly analyzed and summarized in Table 1. The corresponding K_eq and k_uni are included in the Supplement (Table S7).

Table 1 Rate constants (in cm⁶ molecule⁻² s⁻¹) of the SO₃ hydration reaction catalyzed by water and by OA at different altitudes.

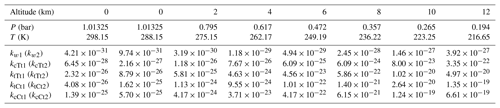
As shown in Table 1, the rate constant in Eq. (2) changes from $\mathrm{4.21}\times {\mathrm{10}}^{-\mathrm{31}}$ cm⁶ molecule⁻² s⁻¹ (298.15 K at 0 km altitude) to $\mathrm{3.92}\times {\mathrm{10}}^{-\mathrm{27}}$ cm⁶ molecule⁻² s⁻¹ (216.15 K at 12 km altitude). For Eq. (4), the transformation of rate constants within the range of altitudes can also be found (k_cTt1: from $\mathrm{6.45}\times {\mathrm{10}}^{-\mathrm{28}}$ to $\mathrm{3.35}\times {\mathrm{10}}^{-\mathrm{22}}$; k_tTt1: from $\mathrm{2.32}\times {\mathrm{10}}^{-\mathrm{26}}$ to $\mathrm{4.97}\times {\mathrm{10}}^{-\mathrm{20}}$; k_tCt1: from $\mathrm{4.08}\times {\mathrm{10}}^{-\mathrm{26}}$ to $\mathrm{1.35}\times {\mathrm{10}}^{-\mathrm{19}}$; k_cCt1: from $\mathrm{1.39}\times {\mathrm{10}}^{-\mathrm{25}}$ to $\mathrm{6.61}\times {\mathrm{10}}^{-\mathrm{19}}$ cm⁶ molecule⁻² s⁻¹). All corresponding rate constant ratios at various altitudes are described in Table 2. Obviously, the rate constant in the OA-catalyzed SO₃ hydration reaction is about 10³–10⁸ times larger than that for the water-catalyzed SO₃ hydration reaction within the range of altitudes. It can be seen from Table 2 that rate constant ratios increase with the temperature decreasing (i.e., with altitude increasing).

Table 2 Relative rate constants of OA-catalyzed SO₃ hydration with respect to water-catalyzed SO₃ hydration at different altitudes.

Table 3 Conformational population for OA conformers at different altitudes.

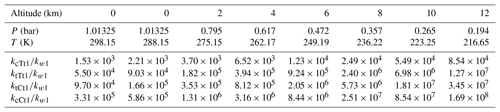
As seen from Table 2, at 298.15 K, the OA-catalyzed SO₃ hydration reaction is 10³–10⁵ faster than the corresponding water-catalyzed reaction (k_cTt1∕k_w1: 1.53×10³; k_tTt1∕k_w1: 5.50×10⁴; k_tCt1∕k_w1: 9.70×10⁴; k_cCt1∕k_w1: 3.31×10⁵). In order to evaluate the catalytic effect of OA, we also list the rate constant ratio between other species and water-catalyzed SO₃ hydration reactions. At 298 K, the nitric-acid-catalyzed rate constant is just 1.19 times larger than the water-catalyzed rate constant (Long et al., 2013). When sulfuric acid functions as an autocatalyst, the value of the rate constant ratio is around 10² (Torrent-Sucarrat et al., 2012). When formic acid acts as a catalyst, its rate constant is 10⁴ greater than that of the water-catalyzed SO₃ hydration at 300 K (Hazra and Sinha, 2011). The rate constant for ammonia-catalyzed SO₃ hydration is 7 orders of magnitude larger than that for the water-catalyzed reaction at 298 K (Bandyopadhyay et al., 2017). These results indicate that the catalytic effect of OA in the SO₃ hydration reaction is better than nitric acid and sulfuric acid, and similar to formic acid, but weaker than ammonia.

Table 4 Concentrations (in molecules cm⁻³) of OA conformers involved in SO₃ hydration and water at different altitudes.

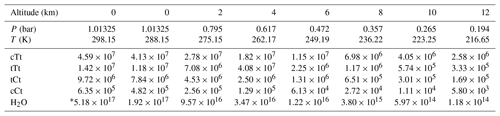
^* The water vapor concentration at 0 km and 298.15 K are obtained from Torrent-Sucarrat et al. (2012). Other water vapor concentrations are taken from US Standard Atmosphere, 1976.

Table 5 Relative rate of SO₃ hydration reaction catalyzed by OA and by water at different altitudes.

^* The rate ν_OA1 represents the sum of reaction rate for Eq. (4; X = cTt, tTt, tCt, cCt). The rate (ν_cTt1∕ν_w1, ν_tTt1∕ν_w1, ν_tCt1∕ν_w1, ν_cCt1∕ν_w1) are shown in Table S10 in the Supplement.

Based on the calculated Gibbs free energy at G4 level (see Table S8 in the Supplement) and the assumption of a Boltzmann distribution, mole fractions for OA conformers can be obtained (Table 3). The calculation method for the conformational population is shown in Supplement S2. The most stable conformer, cTc conformer, accounts for more than 95 % of OA at different altitudes ranging from 0 to 12 km. But this conformer cannot participate in catalyzing the hydration reaction of SO₃. In other words, the conformers involved in catalyzing the SO₃ hydration reaction do not exceed 5 % of OA. In some studies (Martinelango et al., 2007; Bao et al., 2012), observed concentrations of OA in the gas phase range from approximately 10 ng m⁻³ to close to 1 µg m⁻³. To obtain actual concentrations of OA conformers, we assumed 302 ng m⁻³ (2.02×10⁹ molecules cm⁻³) for total OA at 298.15 K and an altitude of 0 km, which are measured by Bao et al. (2012). Combining mole fractions for conformers (cTt, tTt, tCt and cCt) and the total concentration of OA and considering the temperature and pressure effect using the idea gas equation (see Table S9 in the Supplement), the concentration of OA conformers involved in the hydration reaction at various altitudes can be calculated, which is tabulated in Table 4. The water vapor concentration is also included in Table 4.

As shown in Table 5, reaction rate ratios between the SO₃ hydration reactions catalyzed by OA conformers (cTt, tTt, tCt and cCt) and the SO₃ hydration reaction catalyzed by H₂O are described. At an altitude of 0 km, the rate ratio for these two reactions is within the range of 10⁻⁵–10⁻⁶ at two temperatures (298.15 and 288.15 K), which indicates that the OA-catalyzed SO₃ hydration is of minor importance at 0 km at different temperatures. However, as the altitude increases, the OA has an increasing impact on the SO₃ hydration because of an obvious increase in the ratio. When the altitude increases to 10 km, the OA-catalyzed reaction is just 2 orders of magnitude slower than the water-catalyzed reaction. At 12 km altitude, the water-catalyzed hydration reaction is approximately 10 times faster than the OA-catalyzed SO₃ hydration. To sum up, the comparison of relative rates shows that, in the upper troposphere, the OA can play a significant role in enhancing SO₃ hydration to H₂SO₄.

It has been shown that some species including nitric acid, sulfuric acid, formic acid, ammonia, the hydroperoxy radical and OA in our study can catalyze the SO₃ hydration reaction. By forming a two-point hydrogen bond, these species can make a bridge to promote the hydration reaction. It may be concluded that, as long as the species can form a two-point hydrogen bond with a water molecule and SO₃, it has the potential to promote the SO₃ hydration reaction. However, the real atmospheric importance of the species-catalyzed SO₃ hydration reaction needs to be evaluated. That is, compared to the water-catalyzed SO₃ hydration reaction, the species must have sufficient catalytic effect, leading to an increase in rate constants, so as to compensate for the high concentration difference between water vapor and the species.

4 Conclusion

The main conclusion of this work is that oxalic acid (OA), the most abundant dicarboxylic acid in the atmosphere, has the remarkable ability to catalyze SO₃ hydration to H₂SO₄, and has a potential impact on H₂SO₄ formation in the atmosphere.

We have shown that the water-catalyzed hydration reaction of SO₃ has an energy barrier of 5.17 kcal mol⁻¹. For OA, four conformers (cTt, tTt, tCt and cCt) can be involved in the hydration reaction, whereas the most stable conformer (cTc) cannot catalyze the hydration reaction because the formed hydrogen bond structure rejects HAT. Other conformers can catalyze the hydration reaction and the corresponding energy barrier is a little higher or less than 1 kcal mol⁻¹. The results demonstrate that OA has the stronger catalytic ability than water for SO₃ hydration to form H₂SO₄.

According to the kinetic analysis, the rate constant of the OA-catalyzed hydration reaction is greater than that of the water-catalyzed reaction by orders of magnitude, which also reflects the obvious catalytic ability of OA. In addition to the rate constant, the reactant concentration is also an important factor to assess the effect of OA on SO₃ hydration. Based on these two factors, our calculation shows that, in the upper troposphere, OA can play an important role in SO₃ hydration to form H₂SO₄.

This work not only provides insight into the new mechanism of SO₃ hydration in the atmosphere, but also has potential importance for investigating the catalytic effect of OA on other atmospheric reactions.