Molecular-level study on the role of methanesulfonic acid in iodine oxoacid nucleation

Iodic acid (HIO₃) and iodous acid (HIO₂) have been identified as nucleating effectively by the Cosmics Leaving Outdoor Droplets (CLOUD) experiment at CERN, yet it may be hard to explain all HIO₃-induced nucleation. Given the complexity of marine atmosphere, other precursors may be involved. Methanesulfonic acid (MSA), as a widespread precursor over oceans, has been proven to play a vital role in facilitating nucleation. However, its kinetic impacts on the synergistic nucleation of iodine oxoacids remain unclear. Hence, we investigated the MSA-involved HIO₃–HIO₂ nucleation process at the molecular level using density functional theory (DFT) and the Atmospheric Cluster Dynamics Code (ACDC). Our results show that MSA can form stable molecular clusters with HIO₃ and HIO₂ jointed via hydrogen bonds, halogen bonds, and electrostatic attraction after proton transfer to HIO₂. Thermodynamically, the MSA-involved clustering can occur nearly without a free-energy barrier, following the HIO₂–MSA binary and HIO₃–HIO₂–MSA ternary pathway. Furthermore, our results show that considering MSA will significantly enhance the calculated rate of HIO₃–HIO₂-based cluster formation, by up to 10⁴-fold in cold marine regions containing rich MSA and scarce iodine, such as the polar regions Ny-Ålesund and Marambio. Thus, the proposed more efficient HIO₃–HIO₂–MSA nucleation mechanism may provide theoretical evidence for explaining the frequent and intensive bursts of marine iodine particles.

1 Introduction

Marine aerosol, which is the primary natural aerosol (O'Dowd and de Leeuw, 2007), has a significant impact on global climate, radiation balance, and even human health (Wang et al., 2010; Pöschl, 2005). New particle formation (NPF) is a primary source of marine aerosols, which proceeds via nucleation and subsequent growth (Lee et al., 2019; Zhang, 2010; Kulmala et al., 2013; Zhang et al., 2012). Moreover, nucleation, forming critical clusters at 1–2 nm from gaseous precursors, is the pivotal step affecting NPF (Zhang, 2010; Kulmala et al., 2013). However, the chemicals involved in nucleation and the underlying mechanisms remain poorly understood due to technological limitations in molecular-level analysis. Additionally, a lack of comprehensive and long-term ocean observations further hinders our knowledge of marine NPF.

Recent field studies have suggested that marine NPF events are closely related to atmospheric iodine-bearing molecules emitted by algae (Yu et al., 2019; Baccarini et al., 2020; Beck et al., 2021). During NPF events in coastal areas (e.g., Mace Head and Zhejiang; Sipilä et al., 2016; Yu et al., 2019) and polar oceans (e.g., Arctic Ocean) (Baccarini et al., 2020), the nucleation processes are mainly driven by iodic acids (HIO₃). However, the self-nucleation of HIO₃ alone cannot fully explain the observed NPF rates (Rong et al., 2020). More recently, the Cosmics Leaving Outdoor Droplets (CLOUD) experiment at CERN has found that iodous acid (HIO₂) plays a key role in stabilizing HIO₃, enabling effective nucleation by the sequential addition of HIO₃ followed by HIO₂ (He et al., 2021). Further theoretical studies have uncovered the fact that the stabilizing effect of HIO₂ on HIO₃ stems from its role as a base in clustering (S. Zhang et al., 2022; Liu et al., 2023). Although the efficient nucleation of HIO₃ and HIO₂ is overall consistent with the CLOUD measurement (R. Zhang et al., 2022), this mechanism does not account for all HIO₃-induced nucleation in the authentic atmosphere (Ma et al., 2023). Thus, other essential precursors in the marine atmosphere might potentially affect HIO₃–HIO₂ nucleation, but which ones have an effect and how remain largely unexamined.

Methanesulfonic acid (MSA), as a typical marine sulfur precursor, is widespread over oceans (Saltzman et al., 1983; Read et al., 2008; Chen et al., 2012; Yan et al., 2019) with considerable atmospheric concentrations (10⁵–10⁸ molec. cm⁻³) (Eisele and Tanner, 1993; Dal Maso et al., 2002; Chen et al., 2018; Yan et al., 2019). Moreover, MSA has been shown to initiate nucleation with vital atmospheric precursors, such as ammonia and amines, enhancing cluster formation (O'Dowd et al., 2002; Bork et al., 2014; Shen et al., 2019, 2020; Brean et al., 2021; Liu et al., 2022). Importantly, current evidence suggests that MSA can also form stable clusters with HIO₃ or HIO₂ individually, but none of the resulting binary nucleation can explain field measurements well (Ning et al., 2022; Wu et al., 2023). Despite the stabilizing effect of MSA on iodine oxoacids, whether MSA can synergistically nucleate with HIO₃ and HIO₂, as well as the induced kinetic impacts on clustering, remains unknown. Furthermore, given the coexistence of MSA and HIO₃ in different marine regions (Quéléver et al., 2022; Beck et al., 2021), along with the consistent presence of HIO₃ and HIO₂ as homologous substances (Sipilä et al., 2016), the importance of the HIO₃–HIO₂–MSA nucleation mechanism may differ under distinct ambient conditions, but this remains unclear.

Herein, we have systematically investigated the HIO₃–HIO₂-based nucleation involved in MSA, including (HIO₃)_x(HIO₂)_y(MSA)_z ($\mathrm{1}\le x+y+z\le \mathrm{5}$, $\mathrm{0}\le z\le \mathrm{3}$) clusters, by combining a quantum chemical (QC) approach with the Atmospheric Cluster Dynamics Code (ACDC) (McGrath et al., 2012). To probe the nature of cluster formation and investigate the intermolecular interactions, wave-function analysis was performed. Additionally, the Gibbs free energies of cluster formation were calculated to evaluate cluster stability. Moreover, a series of ACDC simulations were executed to delve into the influence of MSA on nucleation rates and mechanisms under varying atmospheric conditions, such as regarding precursor concentration and temperature.

2 Methods

2.1 Quantum chemistry calculations

To locate the low-lying isomers of (HIO₃)_x(HIO₂)_y(MSA)_z ($\mathrm{1}\le x+y+z\le \mathrm{5}$, $\mathrm{0}\le z\le \mathrm{3}$) clusters, the multi-step conformer search was adopted here (details in the Supplement). The resulting stable clusters with the lowest energies were identified at the ωB97X-D/6-311$++$G(3df,3pd) (for C, H, O, and S atoms) + aug-cc-pVTZ-PP with ECP28MDF (for the I atom) level of theory (Francl et al., 1982; Peterson et al., 2003), and the corresponding Cartesian coordinates are collected in Table S9 in the Supplement. In addition, the structures of pure HIO₃, pure HIO₂, pure MSA, HIO₃–HIO₂, HIO₃–MSA, and HIO₂–MSA clusters in the present study have been adopted from previous studies (Rong et al., 2020; S. Zhang et al., 2022; Liu et al., 2023; Ning et al., 2022; Wu et al., 2023). All density functional theory (DFT) calculations were carried out using the Gaussian 09 package (Frisch et al., 2009), where FineGrid and tight convergence were employed. The single-point energy was calculated at the RI-CC2/aug-cc-pVTZ (for C, H, and O atoms) + aug-cc-pV(T+d)Z (for the S atom) + aug-cc-pVTZ-PP with ECP28MDF (for the I atom) level of theory (Hättig and Weigend, 2000) by the TURBOMOLE program (Ahlrichs et al., 1989) because of its success in fitting with the experiments (Lu et al., 2020; Kürten et al., 2018; Rong et al., 2020; Almeida et al., 2013). In the present study, the Gibbs formation free energy (ΔG_ref, kcal mol⁻¹) of the HIO₃–HIO₂–MSA clusters at the reference pressure (1 atm) was calculated as

$$\begin{array}{}\text{(1)}& \mathrm{\Delta }{\mathrm{G}}_{\mathrm{ref}}=\mathrm{\Delta }{E}_{\mathrm{RI}-\mathrm{CC}\mathrm{2}}+\mathrm{\Delta }{G}_{\mathrm{thermal}}^{\mathit{\omega }\mathrm{B}\mathrm{97}\mathrm{X}-\mathrm{D}},\end{array}$$

where ΔE_RI−CC2 is the electronic contribution and $\mathrm{\Delta }{\mathrm{G}}_{\mathrm{thermal}}^{\mathit{\omega }\mathrm{B}\mathrm{97}\mathrm{X}-\mathrm{D}}$ is the thermal contribution to free energy. The ΔG_ref values at different temperatures (T= 258–298 K) were calculated using the Shermo 2.0 code (Lu and Chen, 2021) and are collected in Table S1. Further, given the effect of the precursor's vapor pressures, the ΔG_ref was converted into $\mathrm{\Delta }G\left(P_{\mathrm{1}},P_{\mathrm{2}},\mathrm{\dots },P_n\right)$ (Vehkamäki, 2006) by Eq. (2):

$$\begin{array}{}\text{(2)}& \mathrm{\Delta }G\left(P_{\mathrm{1}},P_{\mathrm{2}},\mathrm{\dots },P_n\right)=\mathrm{\Delta }{G}_{\mathrm{ref}}-k_{\mathrm{B}}T\sum _{i=\mathrm{1}}^n{N}_i\ln \left({\displaystyle \frac{P_i}{P_{\mathrm{ref}}}}\right),\end{array}$$

where n is the number of components within the cluster, k_B denotes the Boltzmann constant, T signifies the temperature, N_i refers to the number of molecules of type i in the number of components in the cluster, and P_i is the partial pressure of component i in the vapor phase.

2.2 Wave-function analysis

Wave-function analysis was carried out using Multiwfn 3.7 (Lu and Chen, 2012) to investigate the binding nature within molecular clusters. The electrostatic potential (ESP) on the van der Waals (vdW) surface was calculated to identify active interaction sites. Specifically, the negative ESP region is electron-rich, while the positive ESP region is electron-deficient, potentially leading to mutual non-covalent interactions, such as hydrogen bonds (HBs) and halogen bonds (XBs). To further quantify the bond strength, the electron density ρ(r), Laplacian electron density ∇²ρ(r), and energy density H(r) at bond critical points (BCPs) were calculated based on the atoms-in-molecules (AIM) theory (Lane et al., 2013).

2.3 Atmospheric cluster dynamic simulations

To explore nucleation kinetics, the Atmospheric Cluster Dynamics Code (ACDC) (McGrath et al., 2012) was adopted here to compute the cluster formation rates, steady-state concentrations, and formation pathways by explicit solution of the birth–death equations (Eq. 3).

$$\begin{array}{}\text{(3)}& \begin{array}{rl}{\displaystyle \frac{\mathrm{d}{C}_i}{\mathrm{d}t}}& ={\displaystyle \frac{\mathrm{1}}{\mathrm{2}}}\sum _{j<i}{\mathit{\beta }}_{j,(i-j)}{C}_j{C}_{(i-j)}+\sum _j{\mathit{\gamma }}_{(i+j)\to i}{C}_{i+j}\\ & -\sum _j{\mathit{\beta }}_{i,j}{C}_i{C}_j-{\displaystyle \frac{\mathrm{1}}{\mathrm{2}}}\sum _{j<i}{\mathit{\gamma }}_{i\to j}{C}_i+Q_i-S_i,\end{array}\end{array}$$

where the subscripts i, j, i−j, and i+j denote different clusters or monomers; C_i is the concentration of cluster i; and β_i,j and ${\mathit{\gamma }}_{(i+j)\to i}$ represent the cluster collision and evaporation rate coefficient, respectively. Q_i and S_i denote the external source and sink terms, respectively. β_i,j is calculated as follows:

$$\begin{array}{}\text{(4)}& {\mathit{\beta }}_{i,j}={\left({\displaystyle \frac{\mathrm{3}}{\mathrm{4}\mathit{\pi }}}\right)}^{\mathrm{1}/\mathrm{6}}{\left({\displaystyle \frac{\mathrm{6}{k}_{\mathrm{B}}T}{m_i}}+{\displaystyle \frac{\mathrm{6}{k}_{\mathrm{B}}T}{m_j}}\right)}^{\mathrm{1}/\mathrm{2}}{\left(V_i^{\mathrm{1}/\mathrm{3}}+V_j^{\mathrm{1}/\mathrm{3}}\right)}^{\mathrm{2}},\end{array}$$

where m_i and V_i represent the mass and volume of cluster i, respectively. $V_i=\mathrm{3}/\mathrm{4}\times \mathit{\pi }\times {\left(d_i/\mathrm{2}\right)}^{\mathrm{3}}$, where the diameter d_i of cluster i is derived from the cluster volume V_i calculated by Multiwfn 3.7 (Lu and Chen, 2012). ${\mathit{\gamma }}_{(i+j)\to i}$ is calculated by Eq. (5):

$$\begin{array}{}\text{(5)}& {\mathit{\gamma }}_{(i+j)\to i}={\mathit{\beta }}_{i,j}{\displaystyle \frac{P_{\mathrm{ref}}}{k_{\mathrm{B}}T}}\exp \left({\displaystyle \frac{\mathrm{\Delta }{G}_{i+j}-\mathrm{\Delta }{G}_i-\mathrm{\Delta }{G}_j}{k_{\mathrm{B}}T}}\right),\end{array}$$

where P_ref is the reference pressure at 1 atm and ΔG is the formation free energy of the cluster.

In the ACDC simulations performed, we considered all possible collision and evaporation processes, including monomer–monomer, monomer–cluster, and cluster–cluster collisions, as well as decomposition of parent clusters into monomers and clusters or into two smaller clusters. Additionally, whether the clusters in the simulated system are stable depends on whether the rate of collision frequencies exceeds the total evaporation rate coefficients ($\mathit{\beta }C/\mathrm{\Sigma }\mathit{\gamma }>\mathrm{1}$) (Table S4). The setting of the boundary conditions of ACDC simulations is summarized in Table S3. An uncertainty analysis was performed in this study (Table S8 and Figs. S15, S16, and S17), with details provided in the Supplement.

3 Results and discussion

Here, conformational analysis was first carried out to study how MSA affects intermolecular interactions in the HIO₃–HIO₂–MSA clusters. The thermodynamic analysis was employed to assess stability of the formed clusters. To gain insights into nucleation mechanisms, a series of ACDC simulations were executed under varying atmospheric conditions.

3.1 Cluster conformational analysis

Strong interactions among nucleation precursors are pivotal for forming stable clusters. To evaluate the binding potential of MSA with HIO₃ and HIO₂, we calculated the ESP-mapped molecular vdW surface to identify interaction sites. As illustrated in Fig. 1, MSA has a positive ESP maximum (+63.95 kcal mol⁻¹) at the H atom of its −OH group, serving as an HB donor. The iodine atoms of HIO₃ and HIO₂ with positive ESP maximums (+51.90 and +45.26 kcal mol⁻¹) can act as XB donors. Additionally, the oxygen atoms in the S=O group (from MSA) and I=O group (from HIO₃ and HIO₂) with strong electronegativity can act as HB or XB acceptor sites due to the lone-pair electrons. Therefore, as shown in Fig. 1d, MSA, HIO₃, and HIO₂ have the potential to form clusters via intermolecular HBs and XBs.

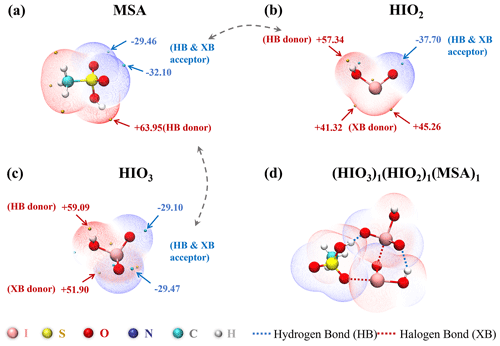
Figure 1 The ESP-mapped molecular vdW surface of (a) MSA, (b) HIO₂, (c) HIO₃, and (d) (HIO₃)₁(HIO₂)₁(MSA)₁. The golden and cyan dots represent the positions of maximums and minimums of ESP (units: kcal mol⁻¹), respectively. The dashed gray arrows signify the site-to-site interaction tendencies.

As presented in Fig. 2, all the identified HIO₃–HIO₂–MSA clusters are structurally stabilized by the network of HBs (dashed blue lines) and XBs (dashed red lines). Within these clusters, the inward-facing oxygen atom and hydroxyl (−OH) group in MSA facilitates it being involved in forming more HBs and XBs compared to the HIO₃–HIO₂ clusters (Fig. S1 in the Supplement). Statistically, within HIO₃–HIO₂–MSA clusters, the percentage of XBs (61 %) is higher than that of HBs (39 %). Notably, during the HIO₃–HIO₂–MSA cluster formation, HIO₂ behaves like a base and is protonated by MSA instead of HIO₃, likely due to stronger acidity of MSA than HIO₃. After the MSA-driven proton transfer to HIO₂, the resulting electrostatic interactions between the formed ion pairs (CH₃SO${}_{\mathrm{3}}^{-}$–H₂IO${}_{\mathrm{2}}^{+}$) further stabilize the clusters. Taken together, MSA can form clusters with HIO₃ and HIO₂ via HBs, XBs, and electrostatic attraction between ion pairs after proton transfer. Additionally, taking the (HIO₃)₁(HIO₂)₃(MSA)₁ cluster for example, there are still some potential remaining unoccupied binding sites as shown in Fig. S2. This suggests that the large-size clusters studied still have unoccupied HB and XB sites that can potentially facilitate the condensation of precursors in the atmosphere, enhancing further growth of marine aerosols.

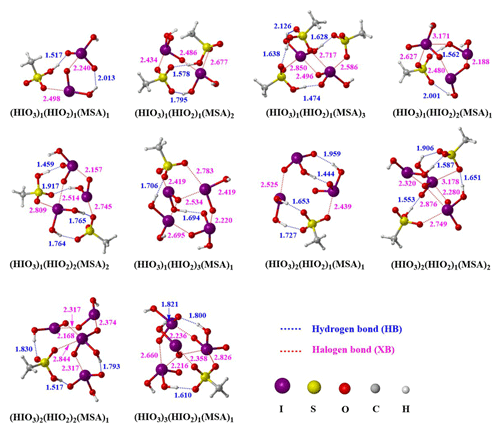
Figure 2 The most stable configurations of the HIO₃–HIO₂–MSA ternary clusters identified at the ωB97XD/6-311$++$G(3df, 3pd) (for C, H, O, and S atoms) + aug-cc-pVTZ-PP with ECP28MDF (for I atom) level of theory. The lengths of bonds are given in ångströms (Å).

To further quantify bond strength within HIO₃–HIO₂–MSA clusters, topological analysis was performed based on the atoms-in-molecules (AIM) theory. The electron density ρ(r), Laplacian electron density ∇²ρ(r), and energy density H(r) at the corresponding bond critical points (BCPs) in the studied HIO₃–HIO₂–MSA clusters were calculated and are collected in Table S2. ρ(r) is generally positively associated with the bond strength. For the HIO₃–HIO₂–MSA clusters, ρ(r) values at the BCPs of the HBs range from 0.0090 to 0.0869 a.u. (atomic units), exceeding the reported threshold of HB (0.002–0.040 a.u.) (Koch and Popelier, 1995; Grabowski, 2004). Moreover, the associated values of ∇²ρ(r) at these BCPs range from 0.0310 to 0.1180 a.u., falling within the suggested range for HB (0.014–0.139 a.u.) (Koch and Popelier, 1995; Grabowski, 2004). Meanwhile, for O–I⋯O XBs, the ρ(r) and ∇²ρ(r) values within HIO₃–HIO₂–MSA clusters range from 0.0068 to 0.1999 and 0.0288 to 0.1744 a.u., respectively. Collectively, MSA can stabilize HIO₃–HIO₂ clusters via more relatively strong HBs and XBs while also protonating HIO₂ to form ion pairs.

3.2 Cluster formation pathways and free-energy surface

To explore how MSA affects HIO₃–HIO₂-based nucleation kinetics, the ACDC simulations were employed to reveal the nucleation mechanism under varying atmospheric conditions. Based on field measurements (Berresheim et al., 2002; Chen et al., 2018; Sipilä et al., 2016; Beck et al., 2021), the ranges of [MSA], [HIO₃], and [HIO₂] are set to be 10⁶–10⁸, 10⁶–10⁸, and 2.0×10⁴–2.0×10⁶ molec. cm⁻³, respectively, where $\left[{\mathrm{HIO}}_{\mathrm{3}}\right]/\left[{\mathrm{HIO}}_{\mathrm{2}}\right]$ is a constant. Here, the condensation sink (CS) coefficient is set to be $\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹ (Dal Maso et al., 2002) and the temperature (T) is 278 K. Under such conditions, the molecular-level nucleation pathways and the corresponding branching ratios are depicted in Fig. 3a. The detailed branch ratio is also shown at 278 K (Fig. S3) and 268 K (Fig. S4). Furthermore, to comprehend how the growth occurs thermodynamically, we herein calculated the Gibbs free energies (ΔG, Eq. 2) along the main clustering pathway at the conditions of T= 268–278 K, [HIO₃] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{6}}$, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{4}}$, and [MSA] $=\mathrm{5.0}\times {\mathrm{10}}^{\mathrm{6}}$ molec. cm⁻³ (Figs. 3b and S5).

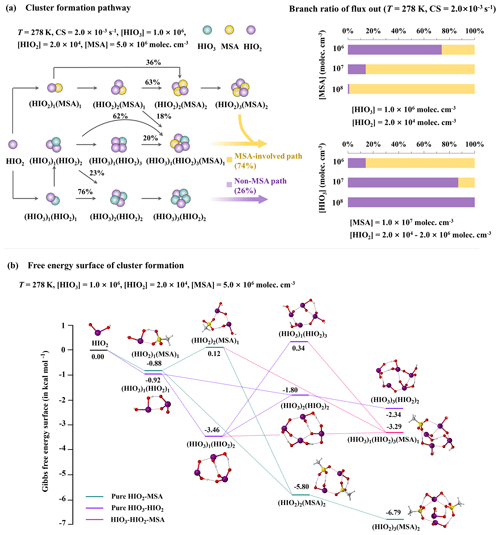
Figure 3 (a) Left: the main cluster growth pathway of the HIO₃–HIO₂–MSA nucleation system at T= 278 K, CS $=\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹, [HIO₃] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{6}}$, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{4}}$, and [MSA] $=\mathrm{5.0}\times {\mathrm{10}}^{\mathrm{6}}$ molec. cm⁻³. Right: the branch ratio of outward flux under varying [MSA] (10⁶–10⁸ molec. cm⁻³) and [HIO₃] (10⁶–10⁸ molec. cm⁻³) values. The yellow and purple arrows (or bars) denote MSA-involved and non-MSA outward flux (or branch ratio), respectively. (b) The Gibbs free energies of cluster formation (ΔG, kcal mol⁻¹) based on the main clustering pathway in the HIO₃–HIO₂–MSA nucleation system. $\left[{\mathrm{HIO}}_{\mathrm{3}}\right]/\left[{\mathrm{HIO}}_{\mathrm{2}}\right]$ is a constant.

As shown in Fig. 3a, the clustering pathways, at T=278 K, CS $=\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹, [HIO₃] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{6}}$, [HIO₃] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{4}}$, and [MSA] $=\mathrm{5.0}\times {\mathrm{10}}^{\mathrm{6}}$ molec. cm⁻³, can be categorized into two main types: (i) MSA-involved pathways, including HIO₂–MSA and HIO₃–HIO₂–MSA nucleation, and (ii) non-MSA pathways, primarily involving HIO₃–HIO₂ nucleation. For the HIO₂–MSA pathway, the initial formation of (HIO₂)₁(MSA)₁ heterodimers occurs without any energy barrier (Fig. 3b). The subsequent cluster growth mainly proceeds via sequential addition of HIO₂ or MSA monomers, partly coupled with cluster collisions. Specifically, 63 % of (HIO₂)₂(MSA)₂ results from (HIO₂)₂(MSA)₁ colliding with MSA monomers with an energy barrier of 1.00 kcal mol⁻¹, while 36 % results from a barrierless combination of two (HIO₂)₁(MSA)₁ clusters. At this point, kinetics drives growth by colliding (HIO₂)₂(MSA)₁ with MSA monomers, instead of following the lowest-energy pathway. This is because the collision frequency of (HIO₂)₁(MSA)₁ and a HIO₂ monomer is higher, stemming from the higher [HIO₂]. Then, the formed (HIO₂)₂(MSA)₂ further collides with a HIO₂ monomer, yielding the stable (HIO₂)₃(MSA)₂ cluster against evaporation. As for HIO₃–HIO₂–MSA nucleation, the formation of an (HIO₃)₁(HIO₂)₃(MSA)₁ cluster arises from the collision of (i) (HIO₂)₂(MSA)₁ with (HIO₃)₁(HIO₂)₁ (18 %, energy barrier: 1.00 kcal mol⁻¹) and (ii) (HIO₃)₁(HIO₂)₂ with (HIO₂)₁(MSA)₁ (62 %, energy barrier: 0.17 kcal mol⁻¹), as well as (iii) (HIO₃)₁(HIO₂)₃ binding with a MSA monomer (20 %, energy barrier: 3.80 kcal mol⁻¹). In addition, for the non-MSA pathway marked by purple arrows, 76 % of (HIO₃)₂(HIO₂)₂ cluster formation arises from the collision between two (HIO₃)₁(HIO₂)₁ clusters, which accords closely with the barrierless pathway shown in Fig. 3b.

Overall, the MSA-involved pathways contribute to 74 % of cluster formation, while the non-MSA path accounts for only 26 %. Although the HIO₃–HIO₂–MSA growth pathway is less favorable than the HIO₃–HIO₂ and HIO₂–MSA pathways at T= 278 K, it can become barrierless at a lower temperature of 268 K (Fig. S5). This result may be explained by the fact that the lower temperature results in a decrease in the evaporation rates of the HIO₃–HIO₂–MSA clusters. The detailed cluster evaporation paths and corresponding γ at 268 and 278 K are collected in Tables S5 and S6. Generally, stable clusters have lower evaporation rates. According to the calculated cluster evaporation rates (Σγ, s⁻¹) at 278 K (Table S7), more than 40 % of the clusters have Σγ values less than 10⁻³ s⁻¹, indicating relatively high stability ($\mathit{\beta }C/\mathrm{\Sigma }\mathit{\gamma }>\mathrm{1}$). Among these resulting stable clusters (see Fig. S6), the majority (85 %) contain HIO₂. Moreover, the concentration of these stable clusters increases gradually with time, even reaching a maximum of 10⁴ molec. cm⁻³ (Fig. S6). Of these stable clusters, initial (HIO₃)₁(HIO₂)₁, (HIO₂)₂, and (MSA)₁(HIO₂)₁ dimers form rapidly, and at ​​​​​​​t≈1 s, heterotrimers (HIO₃)₁(HIO₂)₂ and (MSA)₁(HIO₂)₂ begin to form, after which the larger-sized clusters also form. This time-dependent evidence suggests that MSA is involved in the whole clustering process, from the initial formation of smaller clusters to the large-sized nucleated clusters that potentially further grow. Taken together, these findings highlight the direct and significant involvement of MSA in HIO₃–HIO₂ nucleation, facilitating cluster formation.

As presented on the right of Fig. 3a, the contribution of different clustering pathways to the outward flux varies with precursor concentrations. With increasing [MSA] from 10⁶ to 10⁸ molec. cm⁻³, the contribution of MSA-involved pathways rises from 1 % to 99 % during nucleation. At the median [MSA] of 10⁷ molec. cm⁻³, the MSA-involved pathway contributes 86 %, whereas the non-MSA pathway accounts for just 14 %. In contrast, the ratio of MSA-involved pathways decreases (from 86 % to 0 %) with increasing concentrations of iodine oxoacids. At higher [HIO₃] values of 10⁸ molec. cm⁻³, the HIO₃–HIO₂ pathway dominates nucleation. Predictably, the kinetic impact of MSA on HIO₃–HIO₂ nucleation is more pronounced in marine regions with richer MSA away from iodine sources.

3.3 Enhancement of cluster formation rates

Guided by the clustering pathway analysis, MSA has shown its potential to participate in the HIO₃–HIO₂-based nucleation, but its detailed impacts on cluster formation rates (J, cm⁻³ s⁻¹) remain uncertain. The influence of MSA on J under different atmospheric conditions is systematically analyzed below.

Figure 4 presents the simulated J of the HIO₃–HIO₂–MSA (red bar) and HIO₃–HIO₂ system (gray bar) against the varying temperatures (T= 258–298 K) at CS $=\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹, [HIO₃] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{7}}$, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{5}}$, and [MSA] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{7}}$ molec. cm⁻³. Clearly, J(HIO₃–HIO₂–MSA) is consistently higher than J(HIO₃–HIO₂), highlighting the enhancement effect of MSA on HIO₃–HIO₂-based clustering under common atmospheric temperatures. Specifically, both J(HIO₃–HIO₂–MSA) and J(HIO₃–HIO₂) are negatively dependent on T due to reduced cluster evaporation caused by low T. As a result, MSA could promote nucleation with higher J, especially in the colder regions, such as polar oceans.

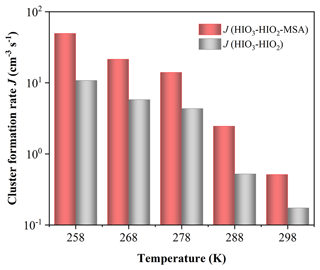
Figure 4 Simulated cluster formation rates J (cm⁻³ s⁻¹) against varying atmospheric temperatures: T= 258–298 K, CS $=\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹, [HIO₃] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{7}}$, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{5}}$, and [MSA] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{7}}$ molec. cm⁻³.

It is worth noting that apart from atmospheric temperature, precursor concentrations might also vary regionally or seasonally, which can further affect nucleation. So, to comprehensively reveal the effect of MSA, here we defined and calculated MSA-driven enhancement factor R (Eq. 6) under varying concentrations of MSA, HIO₃, and HIO₂ (i.e., [MSA], [HIO₃], and [HIO₂], units: molec. cm⁻³).

$$\begin{array}{}\text{(6)}& \begin{array}{rl}R& ={\displaystyle \frac{J({\mathrm{HIO}}_{\mathrm{3}}-{\mathrm{HIO}}_{\mathrm{2}}-\mathrm{MSA})}{J({\mathrm{HIO}}_{\mathrm{3}}-{\mathrm{HIO}}_{\mathrm{2}})}}\\ & ={\displaystyle \frac{J\left(\left[{\mathrm{HIO}}_{\mathrm{3}}\right]=x,\left[{\mathrm{HIO}}_{\mathrm{2}}\right]=y,\left[\mathrm{MSA}\right]=z\right)}{J\left(\left[{\mathrm{HIO}}_{\mathrm{3}}\right]=x,\left[{\mathrm{HIO}}_{\mathrm{2}}\right]=y\right)}},\end{array}\end{array}$$

where J(HIO₃–HIO₂–MSA) and J(HIO₃–HIO₂) represent the cluster formation rate of the HIO₃–HIO₂–MSA and HIO₃–HIO₂ system, respectively. x, y, and z are the adopted [HIO₃], [HIO₂], and [MSA], respectively.

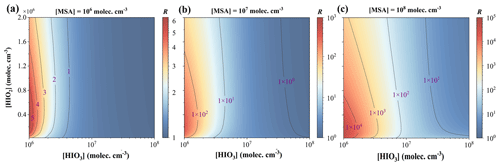
Figure 5 Enhancement strength R of MSA acting on cluster formation rates at varying precursor concentrations: [HIO₃] =10⁶–10⁸ and [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{4}}$–2.0×10⁶ molec. cm⁻³ for (a) [MSA] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{6}}$ molec. cm⁻³, (b) [MSA] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{7}}$ molec. cm⁻³, and (c) [MSA] $=\mathrm{1.0}\times {\mathrm{10}}^{\mathrm{8}}$ molec. cm⁻³; T= 278 K and CS $=\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹.

As seen from Fig. 5, with [MSA] ranging from 10⁶ to 10⁸ molec. cm⁻³ (Fig. 5a–c), the maximum R increases 5- to 10⁴-fold, which is primarily due to MSA-mediated synergistic nucleation with HIO₃ and HIO₂ (recalling Sect. 3.2). Even at a median [MSA] of 1.0×10⁷ molec. cm⁻³, the resulting R can increase approximately 10²-fold. In contrast, R is decayed under conditions of higher [HIO₃] and [HIO₂]. Furthermore, under the conditions with lower $\left[{\mathrm{HIO}}_{\mathrm{3}}\right]/\left[{\mathrm{HIO}}_{\mathrm{2}}\right]$, where R is higher, the contribution of MSA nucleating with HIO₂ increases due to the relative scarcity of HIO₃. Conversely, R decreases at higher $\left[{\mathrm{HIO}}_{\mathrm{3}}\right]/\left[{\mathrm{HIO}}_{\mathrm{2}}\right]$; i.e., the impacts of MSA decrease. That is, the enhancing effect of MSA on J is limited in near-iodine source regions. Naturally, in regions with sparser iodine, the promoting effect of MSA is significant. However, the atmospheric [HIO₃] ranges widely from 10⁶ to 10⁸ molec. cm⁻³. When [HIO₃] is comparable to or higher than [MSA], the HIO₃–HIO₂ pathway contributes more, and the R of MSA decreases with the rising [HIO₃]. It is worth noting that when [HIO₃] is comparable to [MSA], the R of MSA is greater than 2, as the contribution of MSA to clustering includes not only the direct formation of HIO₃–HIO₂–MSA clusters (∼ 20 %), but also its “catalysis” role in facilitating the formation of initial HIO₃–HIO₂ clusters (Fig. S7). To sum up, MSA can promote nucleation, particularly in marine regions characterized by lower T and lower [HIO₃] and [HIO₂]. In addition, we also considered the conditions in relatively polluted (CS $=\mathrm{1.0}\times {\mathrm{10}}^{-\mathrm{2}}$ s⁻¹) and clean (CS $=\mathrm{1.0}\times {\mathrm{10}}^{-\mathrm{4}}$ s⁻¹) environments and found that, similarly to the environment with a CS value of $\mathrm{2.0}\times {\mathrm{10}}^{-\mathrm{3}}$ s⁻¹, MSA exhibits a significant promoting effect on iodine particle formation (Figs. S8–S11). Furthermore, the effect of HIO₂ addition on the whole nucleation system was considered, as it is not only a rate-limiting step for cluster formation, leading to a significant increase in J(HIO₃–HIO₂–MSA) compared to J(HIO₃–MSA) (Fig. S12), but also thermodynamically favorable due to the HIO₃–HIO₂–MSA path being almost barrierless (1.24 kcal mol⁻¹) compared to the HIO₃–MSA pathway (Fig. S13).

3.4 Comparison with field observations

To further assess the atmospheric implications of the proposed HIO₃–HIO₂–MSA nucleation, we simulated J, shown in Fig. 6, based on the ambient conditions of the typical polar regions (e.g., Ny-Ålesund and Marambio) and the mid-latitude marine regions (e.g., Mace Head and Réunion). Subsequently, we compared these simulation results with observed nucleation rates, and the definition of the cluster formation rate is detailed in the Supplement. As shown in Fig. 6a, the J(HIO₃–HIO₂–MSA) simulated at T= 268 K, CS $=\mathrm{4.0}\times {\mathrm{10}}^{-\mathrm{4}}$ s⁻¹, [HIO₃] =10⁵–10⁶, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{3}}$–2.0×10⁴, and [MSA] =10⁶–10⁸ molec. cm⁻³ was compared with field observations in coastal Ny-Ålesund (Beck et al., 2021; He et al., 2021). Both J(HIO₃–HIO₂–MSA) and J(HIO₃–HIO₂) increase with the rising [HIO₃] and [HIO₂]. Importantly, the addition of MSA effectively promotes J to a higher level (orange area), aligning with most field measurements ($\mathrm{1.2}\times {\mathrm{10}}^{-\mathrm{3}}$–$\mathrm{1.2}\times {\mathrm{10}}^{-\mathrm{1}}$ cm⁻³ s⁻¹, gray lines) (Beck et al., 2021). Even when [MSA] is as low as 1.0×10⁶ molec. cm⁻³ (the orange line below), the J(HIO₃–HIO₂–MSA) can be 1 order of magnitude higher than the observed J of $\mathrm{1.2}\times {\mathrm{10}}^{-\mathrm{3}}$ cm⁻³ s⁻¹ (the gray line below). Moreover, the simulated J in Fig. 6b was obtained under the conditions of coastal Marambio, Antarctica: T= 273 K, CS $=\mathrm{1.0}\times {\mathrm{10}}^{-\mathrm{4}}$ s⁻¹, [HIO₃] =10⁵–10⁶, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{3}}$–2.0×10⁴, and [MSA] =10⁶–10⁷ molec. cm⁻³ (Quéléver et al., 2022; He et al., 2021). Compared to J(HIO₃–HIO₂), the MSA-enhanced J(HIO₃–HIO₂–MSA) is overall higher, better fitting with the field observations ($\mathrm{1.2}\times {\mathrm{10}}^{-\mathrm{1}}$–2.4×10¹ cm⁻³ s⁻¹, gray line) (Quéléver et al., 2022). These findings imply that MSA potentially plays a vital role in cold polar oceanic regions, particularly with higher [MSA] during NPF events.

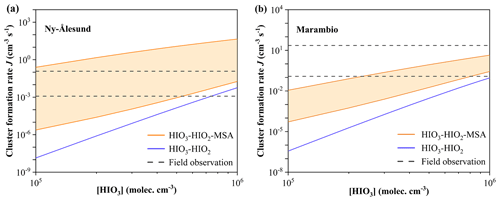
Figure 6 Comparison with the simulated cluster formation rates (J, cm⁻³ s⁻¹) and field observations under the ambient conditions of (a) Ny-Ålesund (T= 268 K; CS $=\mathrm{4.0}\times {\mathrm{10}}^{-\mathrm{4}}$ s⁻¹; and [HIO₃] =10⁵–10⁶, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{3}}$–2.0×10⁴, and [MSA] =10⁶–10⁸ molec. cm⁻³) and (b) Marambio (T= 273 K; CS $=\mathrm{1.0}\times {\mathrm{10}}^{-\mathrm{4}}$ s⁻¹; and [HIO₃] =10⁵–10⁶, [HIO₂] $=\mathrm{2.0}\times {\mathrm{10}}^{\mathrm{3}}$–2.0×10⁴, and [MSA] =10⁶–10⁷ molec. cm⁻³). The orange area, blue line, and gray line represent J(HIO₃–HIO₂–MSA), J(HIO₃–HIO₂), and J(field observation), respectively. $\left[{\mathrm{HIO}}_{\mathrm{3}}\right]/\left[{\mathrm{HIO}}_{\mathrm{2}}\right]$ is a constant.

In addition, the influence of HIO₃–HIO₂–MSA nucleation over the relatively warmer mid-latitude marine areas, such as Mace Head and Réunion, was investigated here (Fig. S14). We found that J(HIO₃–HIO₂–MSA) is slightly higher than J(HIO₃–HIO₂), especially in regions with high concentrations of iodine oxoacids (e.g., Mace Head), showing a relatively limited enhancement effect of MSA on nucleation. Based on the simulated J(HIO₃–HIO₂) (∼ 1.2×10⁴ cm⁻³ s⁻¹), iodine nucleation can almost completely explain the NPF events of Mace Head (Fig. S14a), which provides potential theoretical evidence for explaining the previous findings (Sipilä et al., 2016).

Overall, in the mid-latitude oceans, especially near iodine sources like Mace Head, MSA may have a limited enhancement effect on nucleation. In this case, the clustering process is dominated by abundant iodine oxoacids, while in the colder polar regions, particularly those with higher [MSA] like Marambio, MSA indeed significantly facilitates HIO₃–HIO₂ nucleation, suggesting a vital role in polar NPF.

4 Conclusion

The present study systematically investigates the HIO₃–HIO₂-based nucleation process enhanced by MSA at the molecular level by QC calculations and ACDC simulations. The results indicate that MSA can stabilize HIO₃–HIO₂-based clusters by building the intricate networks with more HBs and XBs. During clustering, MSA replaces HIO₃ in protonating HIO₂ to form ion pairs, resulting in relatively strong electrostatic attractions. In addition, thermodynamic analyses suggest that MSA-involved clustering is nearly barrierless. Compared to the HIO₃–HIO₂ system reported previously, the MSA-involved synergistic nucleation with HIO₃ and HIO₂ proceeds more efficiently, through two additional clustering pathways: (i) HIO₂–MSA binary and (ii) HIO₃–HIO₂–MSA ternary pathways. Moreover, the resulting enhancement effect of MSA on nucleation is more substantial in colder regions, especially with richer MSA, but weaker in the environments near iodine sources. Further comparison with field observations indicates that HIO₃–HIO₂–MSA synergistic nucleation plays a limited role in mid-latitude ocean regions, particularly in regions with abundant iodine (e.g., Mace Head), but a potential role in colder polar regions (e.g., Ny-Ålesund and Marambio).

This study highlights the essential enhancing role of MSA in iodine oxoacids nucleation, and the HIO₃–HIO₂–MSA synergistic nucleation may help to explain the observation of abundant iodine particles during marine NPF events. Given the complex oceanic atmosphere, other potential nucleation precursors beyond MSA, such as sulfuric acid and amines, may also affect the HIO₃–HIO₂ nucleation process and further contribute to the formation of marine iodine particles, deserving future investigations.