Kinetics of OH + SO₂ + M: temperature-dependent rate coefficients in the fall-off regime and the influence of water vapour

The OH-initiated oxidation of SO₂ is the dominant, first step in the transformation of this atmospherically important trace gas to particulate sulfate, and accurate rate coefficients for the title reaction under all atmospheric conditions (pressures, temperatures, and humidity) are required to assess its role in, for example, new particle formation. Prior to this study, no temperature-dependent data were available in the fall-off regime for atmospherically relevant bath gases. We thus address an important omission in the kinetic database for this reaction and highlight significant discrepancies in recommended parameterizations. In this work, generation of OH via pulsed laser photolysis at 248 and 351 nm was coupled to its detection by laser-induced fluorescence to obtain rate coefficients (k₁) for the title reaction at pressures of 14–742 Torr (1 Torr = 1.333 hPa) and temperatures of 220–333 K in N₂ bath gas. In situ SO₂ concentrations, central to accurate kinetic measurements under pseudo-first-order conditions, were measured by optical absorption. Under the conditions of the present study, the termolecular reaction between OH and SO₂ is in the fall-off regime, and we parameterized the rate coefficients in N₂ in terms of low- (k_1,0) and high-pressure (k_1,∞) limiting rate coefficients and a broadening factor (F_C) to obtain $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}=\mathrm{3.03}\times {\mathrm{10}}^{-\mathrm{31}}$ ($T/\mathrm{300}$ K)^−4.10 cm⁶ molecule⁻² s⁻¹, $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.00}\times {\mathrm{10}}^{-\mathrm{12}}$ cm³ molecule⁻¹ s⁻¹, and F_C=0.58. The effects of water vapour on the title reaction were explored through measurements in N₂–H₂O mixtures at 273, 298, and 333 K using the same experimental methods. The rate coefficients are significantly enhanced by the presence of water vapour with $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{1.65}\times {\mathrm{10}}^{-\mathrm{30}}$ ($T/\mathrm{300}$ K)^−4.90 cm⁶ molecule⁻² s⁻¹, which indicates that H₂O is a factor >5 more efficient in quenching the HOSO₂* association complex than N₂. A model-based comparison of our rate coefficients and parameterization with previous literature measurements and recommendations of evaluation panels are presented and discussed. The use of the new parameterization instead of the IUPAC or NASA evaluations, particularly after including H₂O as a third-body quencher, leads to a significant (10 %–30 %) reduction in the lifetime of SO₂ in some parts of the atmosphere and increases the ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{SO}}_{\mathrm{2}}$ ratio concomitantly.

1 Introduction

Sulfur enters the atmosphere predominantly in the form of gaseous sulfur dioxide (SO₂), which results from both natural and anthropogenic sources (Stevenson et al., 2003). The amount of SO₂ produced from human activities, particularly via fossil fuel combustion, is similar to that resulting from natural emissions (e.g. volcanic eruptions) and can be dominant on a regional scale (Brown, 1982; Brimblecombe, 2013; Lelieveld et al., 1997). SO₂ is a key intermediate during the complex chemical and photochemical reactions that ultimately transform reduced sulfur compounds to sulfates. The oxidation of SO₂ in the atmosphere occurs in the gas phase as well as in droplets and aerosol particles (Liu et al., 2020; Cox, 1979; Beilke and Gravenhorst, 1978). The gas-phase oxidation of SO₂ is initiated mainly by the OH radical (Reaction R1), with a small contribution in forested regions by stabilized Criegee intermediates (Mauldin Iii et al., 2012; Huang and Chao, 2015). Based on a seasonal, global average boundary layer OH concentration of 1 × 10⁶ molecule cm⁻³ and the present recommendation for the rate coefficient (Atkinson et al., 2004; IUPAC, 2021), the lifetime of SO₂ with respect to reaction with OH (to form HOSO₂) is a few days.

$$\begin{array}{}\text{(R1)}& {\mathrm{SO}}_{\mathrm{2}}+\mathrm{OH}+\mathrm{M}\to {\mathrm{HOSO}}_{\mathrm{2}}+\mathrm{M}\end{array}$$

Once collisionally stabilized HOSO₂ reacts with O₂ to form SO₃ (Reaction R2), which is hydrolysed to sulfuric acid (H₂SO₄) (Reaction R3),

$$\begin{array}{}\text{(R2)}& {\displaystyle }{\mathrm{HOSO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\to {\mathrm{HO}}_{\mathrm{2}}+{\mathrm{SO}}_{\mathrm{3}}\text{(R3)}& {\displaystyle }{{\mathrm{SO}}_{\mathrm{3}}+\left({\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right)}_n\to {{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}+\left({\mathrm{H}}_{\mathrm{2}}\mathrm{O}\right)}_{n-\mathrm{1}},\end{array}$$

H₂SO₄ can initiate particle formation (e.g. via reactions with basic trace gases such as NH₃) or condense on existing particles, thus contributing to aerosol formation, growth, and cloud droplet nucleation (Kulmala et al., 1998; Vehkamäki et al., 2002; Sipilä et al., 2010; Saltzman et al., 1983). The aforementioned processes occur throughout the atmosphere, affecting ecosystems as well as the earth troposphere radiation budget and thus climate (Badr and Probert, 1994; Lelieveld and Heintzenberg, 1992; Stevenson et al., 2003; Feichter et al., 1996). In addition, the oxidation of SO₂ to sulfate is a major sink of stratospheric OH and water (Bekki, 1995) and provides surface area for heterogeneous processes that, for example, contribute to stratospheric ozone depletion (Weisenstein et al., 1996; Heckendorn et al., 2009).

As a result of its central importance to atmospheric chemistry, the kinetics of the title reaction have been investigated in numerous experimental studies. The results of laboratory investigations of k₁, which serve as the basis for the IUPAC (IUPAC, 2021) and NASA (Burkholder et al., 2020) evaluation panels, are summarized in Table 1. A few early measurements of k₁ at around 298 K and 1 atm N₂ (or air) (Izumi et al., 1984; Barnes et al., 1986; Davis et al., 1979; Castleman Jr. and Tang, 1976; Cox and Sheppard, 1980) are not included in Table 1, as they do not contain information on the pressure or temperature dependence of the title reaction and display relatively large differences in k₁ at 298 K and 1 atm, with values ranging from 5.99 × 10⁻¹³ to 1.22 × 10⁻¹² cm³ molecule⁻¹ s⁻¹. Surprisingly, for such an important reaction in atmospheric chemistry, no temperature-dependent measurements of k₁ in the fall-off regime have been carried out in atmospherically relevant bath gases (e.g. air or N₂) but in He, for which the collision efficiency is much lower than for N₂ and O₂, the dominant components of the atmosphere. In addition, the latest measurements of k₁, (Blitz et al., 2017a) using modern, laser-based photolysis methods, suggest that previous measurements were strongly biased (to larger values) by the photoexcitation/dissociation of SO₂ (see later).

Table 1 Temperature- and pressure-dependent measurements of k₁.

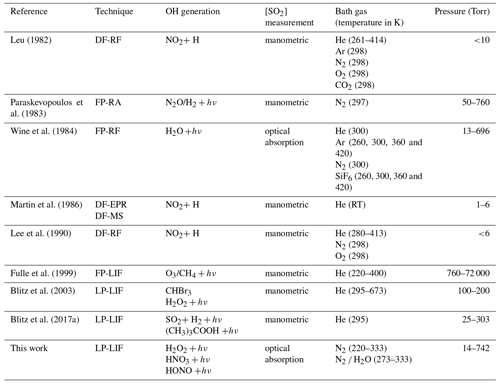
Notes: DF-RF is discharge flow–resonance fluorescence, FP-RA is flash photolysis–resonance absorption, FP-RF is flash photolysis–resonance fluorescence, DF-EPR-MS is discharge flow–electric paramagnetic resonance/mass spectrometry, FP-LIF is flash photolysis–laser-induced fluorescence, LP-LIF is laser flash photolysis–laser-induced fluorescence. RT is room temperature.

The title reaction is in the fall-off regime across the temperature and pressure ranges in the atmosphere. To parameterize the rate coefficients for such reactions, the Troe-type formulation (Troe, 1983) is widely used, which requires experimentally determined high-pressure (k_1,∞) and low-pressure (k_1,0) limiting rate coefficients as well as a broadening factor describing the transition at intermediate pressures. To date, the rate coefficient at the high-pressure limit has not been measured directly, and the value of k₁ at 298 K of ∼ 2.4 ×  10⁻¹² cm³ molecule⁻¹ s⁻¹ at 96 bar (72 000 Torr) of He, the highest pressure explored to date, is still below the extrapolated k_1,∞ value of 3.6 × 10⁻¹² cm³ molecule⁻¹ s⁻¹ (Fulle et al., 1999). Blitz et al. (2017a) derived values of k_1,∞ indirectly via measurements of the vibrational relaxation of OH in collision with SO₂ but obtained a much lower value of $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{7.2}\times {\mathrm{10}}^{-\mathrm{13}}$ cm³ molecule⁻¹ s⁻¹. The values of k_1,∞, presently recommended by IUPAC (last updated in November 2017) and NASA (last updated in May 2020), are 2.0 × 10⁻¹² and 1.7 × 10⁻¹² cm³ molecule⁻¹ s⁻¹, respectively, falling between those of Fulle et al. (1999) and Blitz et al. (2017a).

From the studies reporting low-pressure limiting rate coefficients, largely obtained in flow-tube experiments, it is unclear whether k_1,0 could be accurately derived through linear fitting to measured values of k₁ over a small pressure range, as measurements at the experimental pressures (a few Torr) were already impacted by fall-off. As discussed by Amedro et al. (2019), other factors such as wall losses of OH add to the difficulty of deriving accurate low-pressure limiting rate coefficients in flow-tube experiments, especially at low temperatures.

Apart from N₂ and O₂, water vapour (H₂O) is a major atmospheric component. In particular, in the relatively warm boundary layer, for example, in the tropics, the mixing ratio of water vapour can exceed 5 %. For termolecular reactions involving OH, H₂O may be expected to be a more efficient third-body quenching agent than N₂ and O₂ (Troe, 2003; Amedro et al., 2020), and the presence of water vapour can significantly enhance rate coefficients of third-body reactions in the low-pressure and fall-off regimes. A recent study (Amedro et al., 2020) demonstrated that neglecting to consider the influence of water vapour would lead to an underestimation in the rate coefficients of the OH + NO₂ reaction by ∼ 10 % in some parts of the lower atmosphere. Given the similarities between the title reaction and the OH + NO₂ reaction, the participation of H₂O as a third-body quencher may also significantly enhance k₁, and such effects need to be examined experimentally.

The goals of this study were to address some of the shortcomings in the present dataset on the reaction of OH with SO₂ by accurately measuring k₁ in N₂ bath gas over a wide temperature/pressure range relevant for the atmosphere and to elucidate the role of water vapour as a third-body quencher in the title reaction. Such datasets are expected to yield a new parameterization for k₁ with reduced uncertainties.

2 Experimental methods

Rate coefficients for the title reaction were derived using the pulsed-laser-photolysis (PLP) laser-induced fluorescence (LIF) technique under pseudo-first-order conditions ([SO₂] ≫ [OH]; see Sect. 3.2). The concentrations of SO₂, as well as H₂O in the experiments exploring the effect of water vapour, were obtained online via optical absorption measurements.

2.1 PLP-LIF technique

Detailed descriptions of the PLP-LIF setup are given in previous publications (Wollenhaupt et al., 2000; Amedro et al., 2019). Briefly, the reaction took place in a jacketed, cylindrical quartz reactor with a volume of ∼ 500 cm³, and the temperature inside the reactor was regulated by circulating ethanol (at 220 K) or 60 : 40 ethylene glycol mixture (250, 273, and 333 K) through an outer jacket. The temperature at the centre of the reaction volume was monitored by a J-type thermocouple before and after each experiment; the pressure was measured using 100 and 1000 Torr capacitance manometers. The average linear velocity of gas flowing through the tubular reactor was kept at ∼ 8–9 cm s⁻¹ by adjusting the total flow rates. As the flow direction and laser beams (0.8 cm diameter) are perpendicular to each other, a linear velocity of over 8 cm⁻¹ ensures that a fresh gas sample was photolysed at each laser pulse (10 Hz), and the volume of gas imaged onto the photomultiplier tube (PMT) is replenished between pulses. For the vast majority of experiments, a KrF excimer laser (COMPex 205F, Coherent) provided pulses of 248 nm light (∼ 20 ns) to photolyse H₂O₂ or HNO₃ for the generation of OH radicals in the vibrational ground state (Schiffman et al., 1993).

$$\begin{array}{}\text{(R4)}& {\displaystyle }{\mathrm{H}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{2}}+h\mathit{\nu }\left(\mathrm{248}\mathrm{nm}\right)\to \mathrm{2}\mathrm{OH}\text{(R5)}& {\displaystyle }{\mathrm{HNO}}_{\mathrm{3}}+h\mathit{\nu }\left(\mathrm{248}\mathrm{nm}\right)\to \mathrm{OH}+{\mathrm{NO}}_{\mathrm{2}}\end{array}$$

In addition, a limited set of experiments were carried out in which HONO was photolysed at 351 nm (XeF excimer laser):

$$\begin{array}{}\text{(R6)}& \mathrm{HONO}+h\mathit{\nu }\left(\mathrm{351}\mathrm{nm}\right)\to \mathrm{OH}+\mathrm{NO}.\end{array}$$

OH radicals thus generated were excited at 282 nm $\left(A^{\mathrm{2}}\mathrm{\Sigma }\right(\mathit{\nu }=\mathrm{1})\leftarrow X^{\mathrm{2}}\mathrm{\Pi }(\mathit{\nu }=\mathrm{0}\left)\right)$ by a YAG-pumped dye laser, and the subsequent OH fluorescence was detected by a photomultiplier placed behind a 309 nm interference filter and a BG 26 glass cut-off filter. The photolysis laser fluence was measured by a Joule meter placed behind the exit window of the reactor, and the shot-to-shot variation in the intensity of the dye laser was monitored by a photodiode. The timing between the triggers of the photolysis and probe lasers was scanned using a digital delay generator; time-dependent OH profiles were obtained by accumulating the fluorescence signals using a boxcar integrator. Fluorescence resulting from the excitation of SO₂ at 282 nm was also observed using this setup, which results in a constant background signal during each experiment. Typically, 20 points were recorded before triggering the excimer laser to measure the background signal, which also includes a component from electronic noise. The background signal was subtracted from the measured OH profile before further kinetic analyses.

2.2 Online optical absorption measurements

The accurate determination of the rate coefficients under pseudo-first-order conditions requires reliable quantification of SO₂ concentrations ([SO₂]). In this work, online optical absorption cells were located both upstream and downstream of the reactor to monitor the SO₂ concentration at room temperature (298 K). Upstream of the reactor, light from a deuterium lamp was directed through a multi-pass absorption cell (l=110 cm $\times \mathrm{8}=\mathrm{880}$ cm) and detected by a low-resolution (Δλ=2 nm) spectrograph (Ocean-Optics USB 2000). The measured optical density over the wavelength range of 240–325 nm was fit to a reference spectrum (Manatt and Lane, 1993) to derive SO₂ concentrations. The second (downstream) optical absorption cell (l=34.8 cm) was equipped with a low-pressure zinc lamp and monitored optical density at 213.86 nm to measure the concentration of SO₂ (see Sect. 3.1) and the OH precursors H₂O₂ and HNO₃. SO₂ concentrations were calculated using a 213.86 nm cross-section derived in separate experiments (see later). Concentrations of H₂O₂ and HNO₃ were calculated using σ_213.86 nm (H₂O₂) $=\mathrm{3.30}\times {\mathrm{10}}^{-\mathrm{19}}$ (Vaghjiani and Ravishankara, 1989) and σ_213.86 nm (HNO₃) $=\mathrm{4.52}\times {\mathrm{10}}^{-\mathrm{19}}$ cm² molecule⁻¹ (Dulitz et al., 2018).

Approximate, initial concentrations of OH radicals were calculated from the precursor concentrations and the excimer laser fluence as recorded by the Joule-meter. A third absorption cell (l=40 cm, located downstream of the 213.86 nm cell) equipped with a low-pressure 184.95 nm Hg lamp as light source was additionally used in the experiments exploring the impact of water vapour. In this case, a cross-section of σ_184.95 nm (H₂O) = 7.14 × 10⁻²⁰ cm² molecule⁻¹ (Cantrell et al., 1997) was used.

2.3 Chemicals

Nitrogen (N₂; 99.999 %) was supplied by Westfalen and used without further purification. SO₂ (Merck, 99.8 %) was diluted in N₂ and stored in a stainless-steel cylinder. Hydrogen peroxide (H₂O₂, AppliChem, 35 %) was vacuum-distilled to >90 wt % purity. Anhydrous HNO₃ was synthesized by mixing potassium nitrate (KNO₃; Sigma-Aldrich, 99 %) and sulfuric acid (H₂SO₄; Roth, 98 %) and condensing the HNO₃ vapour in a trap cooled with liquid nitrogen. Distilled water (Merck, liquid-chromatography-grade) was degassed before use.

3 Results and discussion

3.1 Quantification of SO₂ concentrations

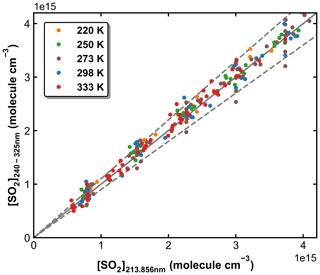
SO₂ absorption spectra over 170–330 nm at room temperature are presented in Fig. 1, including a low-resolution (Δλ=0.1 nm) dataset reviewed and compiled by Manatt and Lane (1993) and a set of higher-resolution data ($\mathrm{\Delta }\mathit{\lambda }=\mathrm{2.5}\times {\mathrm{10}}^{-\mathrm{4}}$ nm) spanning 198–325 nm reported by Stark et al. (Stark et al., 1999; Rufus et al., 2003). The two absorption bands (240–330 and 170–230 nm) correspond to the $\stackrel{\mathrm{̃}}{B}{(}^{\mathrm{1}}{B}_{\mathrm{1}})\leftarrow \stackrel{\mathrm{̃}}{X}{(}^{\mathrm{1}}{A}_{\mathrm{1}})$ and the $\stackrel{\mathrm{̃}}{C}{(}^{\mathrm{1}}{B}_{\mathrm{2}})\leftarrow \stackrel{\mathrm{̃}}{X}{(}^{\mathrm{1}}{A}_{\mathrm{1}})$ transitions, respectively (Rollins et al., 2016). The high-resolution measurements by Stark et al. (1999) reveal very narrow rotational–vibrational lines in the 200–220 nm region and cross-sections that are factors of 2–3 larger than those of Manatt and Lane (1993). Clearly, the use of an inappropriate cross-section at the wavelength of our very narrow Zn atomic emission line (∼ 213.86 nm) could introduce large uncertainty in SO₂ concentration. For this reason, we derived an effective cross-section for our Zn-lamp emission by comparing absorption measurements in the multi-pass cell and the 213.86 nm cell for a flowing gas mixture of SO₂ in N₂. We refer to this cross-section as an “effective” cross-section as nearby, weaker atomic lines will also pass through the interference filter (214 ± 5 nm) used to isolate the 213.86 nm line. In Fig. 1, broadband (240–325 nm, resolution ∼ 2 nm) absorption measurements recorded using the multi-pass cell are compared to the reference spectrum of Manatt and Lane (1993). SO₂ concentrations were determined through least-squares fitting the measured optical density to the reference spectrum degraded to the same resolution as our spectrometer. The effective cross-section at 213.86 nm was then derived according to the Beer–Lambert law, taking into account pressure differences in the two absorption cells, as shown in Fig. 2. The slope of a linear regression through the data points is the effective cross-section of SO₂ at the emission wavelength of our low-pressure Zn lamp. The value obtained (4.00 × 10⁻¹⁸ cm² molecule⁻¹) is very close (within ∼ 3 %) to the value of 4.07 × 10⁻¹⁸ cm² molecule⁻¹ listed at 213.86 nm by Stark et al. (1999) and the value of 3.87 × 10⁻¹⁸ cm² molecule⁻¹ derived by Wine et al. (1984) using a similar experimental setup. The high coefficient of determination (R²=0.9984) for the linear regression of all data points obtained at different pressures (41 to 494 Torr) indicates that the effective value of σ_213.86(SO₂) is independent of pressure. SO₂ concentration measurements using the two absorption cells in the experiments at different temperatures are plotted in Fig. 3. The good agreement (slope very close to 1) between the measured (at room temperature) SO₂ concentrations upstream and downstream of the reactor (held at temperatures between 220 and 333 K) shows that no SO₂ is lost in transit (e.g. via adsorption to surfaces or condensation) through the cold/hot reactor. The scatter in this plot is caused by small baseline shifts in the long path measurements upstream of the reactor.

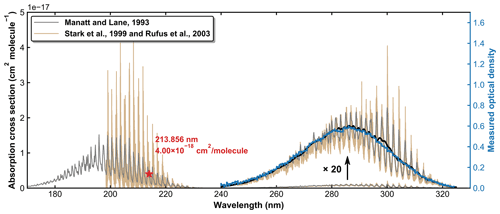
Figure 1 SO₂ absorption spectra reported by Manatt and Lane (1993) and Stark et al. (1999; Rufus et al., 2003). The black line is the result of degrading the resolution in the 240–325 nm region to that of our optical density measurements (blue line).

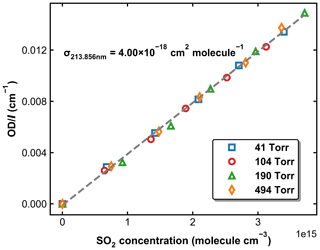
Figure 2 Normalized optical density in the 213.86 nm absorption cell as a function of SO₂ concentration measured using the multi-pass absorption cell. The experiments were performed at 298 K and pressures of 41, 104, 190, and 494 Torr. The dashed grey line is a linear regression, for which the slope is the effective cross-section of SO₂ at 213.86 nm.

Figure 3 SO₂ concentrations measured in experiments at different temperatures using the multi-pass absorption cell upstream of the reactor (y axis) and the 213.86 nm absorption cell downstream of the reactor (x axis). The solid line represents y=x, and the dashed lines are y=1.1x and y=0.9x.

3.2 Rate coefficients for the title reaction in N₂

Rate coefficients for the title reaction in N₂ bath were measured at pressures between 14 and 742 Torr at five different temperatures (220, 250, 273, 298, and 333 K). In deriving a parameterization for the rate coefficient in air (see later), we assume that, as is the case for the vast majority of termolecular reactions, N₂ and O₂ (the major components of air) have the same collisional quenching efficiency. In all experiments, the initial OH concentration was kept sufficiently low (10¹¹–10¹² molecule cm⁻³) relative to that of SO₂ (6 × 10¹⁴ to 6 × 10¹⁵ molecule cm⁻³), so that pseudo-first-order conditions applied and the decay of OH may be described by

$$\begin{array}{}\text{(1)}& {\left[\mathrm{OH}\right]}_t=[\mathrm{OH}{]}_{\mathrm{0}}(-k^{\prime }t),\end{array}$$

where [OH]_t is the OH concentration at time t after the photolysis laser pulse, and k^′ is the pseudo-first-order rate coefficient defined as

$$\begin{array}{}\text{(2)}& k^{\prime }=k_{\mathrm{1}}\left[{\mathrm{SO}}_{\mathrm{2}}\right]+k_d,\end{array}$$

where k₁ (in cm³ molecule⁻¹ s⁻¹) is the bimolecular rate coefficient for the title reaction, and k_d (in s⁻¹) accounts for the OH removal through reactions with H₂O₂ or HNO₃ (Reaction R7 or R8) as well as OH loss due to diffusion out of the reaction zone.

$$\begin{array}{}\text{(R7)}& {\displaystyle }\mathrm{OH}+{\mathrm{H}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{2}}\to {\mathrm{H}}_{\mathrm{2}}\mathrm{O}+{\mathrm{HO}}_{\mathrm{2}}\text{(R8)}& {\displaystyle }\mathrm{OH}+{\mathrm{HNO}}_{\mathrm{3}}\to {\mathrm{H}}_{\mathrm{2}}\mathrm{O}+{\mathrm{NO}}_{\mathrm{3}}\end{array}$$

Figure 4 displays a set of OH decay profiles at six different SO₂ concentrations ranging from 0 to 5.34 × 10¹⁵ molecule cm⁻³ at 298 K in 59.9 Torr N₂. H₂O₂ was used as OH precursor in this dataset, and the initial OH concentration was ∼ 4 × 10¹¹ molecule cm⁻³. Each OH decay is the average of 20 measurements taken over a period of ∼ 5 min. For each profile, the decay constant k^′ was obtained through least-squares fitting to Eq. (1). From each set of OH decays at a given temperature, pressure, and bath gas, the associated bimolecular rate coefficient k₁ was derived using Eq. (2) as shown in Fig. 5, which plots k^′ against [SO₂] at 298 K at four different pressures.

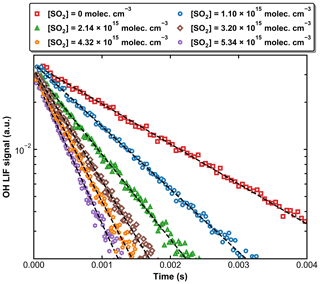
Figure 4 Exponential decay of OH at 298 K and 59.9 Torr (N₂ bath gas) in the presence of six different SO₂ concentrations. OH was generated by the photolysis of H₂O₂ at 248 nm. The dashed black lines are fits using Eq. (1).

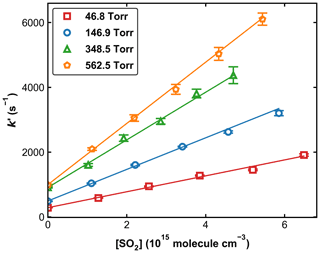
Figure 5 Pseudo-first-order rate coefficients (k^′) as a function of the SO₂ concentration ([SO₂]) at four different pressures at 298 K. Error bars represent 2σ statistical uncertainty. The lines are weighted, linear regressions.

Previous experimental studies have reported that the photoexcitation/dissociation of SO₂ can have a large impact on the kinetics of OH loss. To circumvent this, optical filters containing SO₂ have been used to reduce the absorption of, for example, light from flash lamps (160–220 nm) (Paraskevopoulos et al., 1983; Wine et al., 1984). The 248 nm laser light used in the current setup is beyond the SO₂ photodissociation threshold of 219 nm, so single-photon SO₂ photodissociation cannot affect the measurements for k₁. However, using a similar setup at 248 nm, Blitz et al. (2017b, a) found evidence for two-photon dissociation of SO₂ in their experiments in He and reported a high-pressure limiting rate coefficient that is lower (by a factor of 2–5) than all other measurements or recommendations by evaluation panels. Blitz et al. (2017b) suggested that previous measurements were biased by additional OH removal by radical–radical reactions initiated by the two-photon dissociation of SO₂. In order to evaluate whether SO₂ photoexcitation could have impacted on our measurements of k₁ in N₂, we conducted measurements at 298 K and 14 Torr in N₂ with the excimer laser power varied by a factor of ∼ 14. Over this energy range, the impact of any two-photon processes would scale by a factor of ∼ 200. k₁ was thus measured in a total of 10 experiments, for which SO₂ concentrations ranged from 1.2 × 10¹⁵ to 6.0 × 10¹⁵ molecule cm⁻³, the concentration of H₂O₂ was kept at around 3 × 10¹⁴ molecule cm⁻³, and the laser fluence was varied from ∼ 0.5 to ∼ 9.5 mJ cm⁻². Values of k₁ as a function of the 248 nm laser fluence are displayed in Fig. 6. Our data clearly show that k₁ is independent of laser fluence (the average value is 1.29 ± 0.05 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹), suggesting that, under our experimental conditions in N₂ bath, secondary reactions between OH radicals resulting from the photoexcitation of SO₂ are insignificant. This observation helps to rule out that single- or two-photon processes involving SO₂ excitation or dissociation do not bias the OH decay and that reactions of OH with, for example, products of Reaction (R1) (i.e. HOSO₂) or reaction with HO₂ and NO₃ (formed in Reactions R7 and R8), are unimportant. To examine the potential for two-photon photolysis of SO₂ in He bath gas, we added H₂O to a reaction mixture of H₂O₂, SO₂, and He and observed non-exponential OH kinetics, suggesting the intermediacy of O(¹D):

$$\begin{array}{}\text{(R9)}& {\displaystyle }{\mathrm{SO}}_{\mathrm{2}}+\mathrm{2}h\mathit{\nu }\to \mathrm{O}{(}^{\mathrm{1}}\mathrm{D})+\mathrm{SO}\text{(R10)}& {\displaystyle }\mathrm{O}{(}^{\mathrm{1}}\mathrm{D})+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\to \mathrm{2}\mathrm{OH}.\end{array}$$

We emphasize that such effects were not seen in N₂ bath gas in which O(¹D) is rapidly quenched to less reactive O(³P).

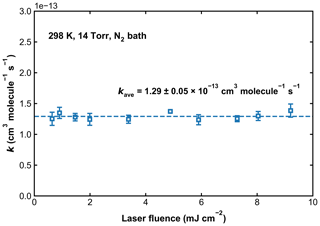
Figure 6 Values of k₁ measured at 298 K and in 14 Torr of N₂ bath gas. The 248 nm laser fluence was varied by a factor of ∼ 14. The dashed line represents the average rate coefficient.

To confirm beyond doubt that our measurements using 248 nm PLP are not biased by SO₂ excitation, an additional experiment was performed (193.2 Torr and 298 K) using HONO photolysis at 351 nm as OH precursor. At 351 nm, the SO₂ absorption cross-section is ∼ 3 orders of magnitude lower than at 248 nm, and SO₂ excitation is negligible. HONO was generated in situ by the dropwise addition of a 0.1 M NaNO₂ solution to a 20 wt % H₂SO₄ solution, and the characteristic bands at around 342, 354, and 368 nm (Stutz et al., 2000) were monitored by the multi-pass optical absorption cell. This setup provided sufficient amounts of HONO (∼ 10¹⁴ molecule cm⁻³) for kinetic measurements for about 1–1.5 h after adding a few drops of the NaNO₂ solution. Note that the concentration of H₂O above the H₂SO₄ solution is very low, so that these can be considered to be dry experiments (i.e. in N₂ bath gas).

Unlike the H₂O₂ and HNO₃ sources of OH described above, the concentration of HONO was not stable over the time required to measure a series of values of k^′ in the presence of various amounts of SO₂. Therefore, measurements of k^′ with and without SO₂ were conducted intermittently. Figure 7 displays the measured first-order OH decay rate constants (k^′) with different amounts of SO₂ present in the system and over a period of ∼ 2.5 h. In the absence of SO₂ (blue symbols), the k^′ decreased from an initial value of ∼ 2800 s⁻¹ to one of ∼ 800 s⁻¹ at 5200 s. This is mainly due to the reaction of OH with HONO and impurities such as NO₂ and NO. After ∼ 5400 s a few drops of NaNO₂ were again added to the H₂SO₄ solution, and the increase in [HONO] was accompanied by an increase in k^′. The decay in k^′ over time was fit with a second-order polynomial function, which, via interpolation, was used to calculate the contribution of OH loss in the absence of SO₂ (i.e. k_d) from the individual values obtained with SO₂ present. Based on the loss rate constants in the absence of SO₂ and the rate coefficient for reaction of OH with HONO (k₁₁ (298 K) = 6.0 × 10⁻¹² cm³ molecule⁻¹ s⁻¹) (Atkinson et al., 2004), we estimate the HONO concentrations to be ∼ 1–5 × 10¹⁴ molecule cm⁻³.

$$\begin{array}{}\text{(R11)}& \mathrm{HONO}+\mathrm{OH}\to {\mathrm{NO}}_{\mathrm{2}}+{\mathrm{H}}_{\mathrm{2}}\mathrm{O}\end{array}$$

Combined with the photolysis laser fluence of around 1 mJ cm⁻², this results in an initial OH concentration of 0.3–1.5 × 10¹¹ molecule cm⁻³. A total of five different SO₂ concentrations were used in these experiments, and at each SO₂ concentration, the measurement of k^′ was repeated four times. The averaged values of k^′, after correction for the contribution from reactions of OH with HONO and other impurities, as well as diffusion loss of OH, are plotted against SO₂ concentration in Fig. 8. A linear regression yields a value of k₁= 4.91 ± 0.13 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹. A similar set of experiments at 273 K and 295 Torr yielded k₁= 8.44 ± 0.19 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹. Owing to the more convoluted experimental procedure and data analysis and also the larger OH losses in the absence of SO₂ (up to ∼ 3000 s⁻¹), the overall uncertainty of the rate coefficient obtained in this manner is larger than that obtained using H₂O₂ and HNO₃ as OH precursors and the difference (∼ 10 %) between the rate constant obtained using the 248 nm photolysis of H₂O₂ or HNO₃ or HONO as OH source is not considered significant. In any case, this level of agreement (combined with the laser fluence variation described above) rules out a bias to k₁ of the magnitude reported by Blitz et al. (2017b) when working at 248 nm in N₂ bath gas.

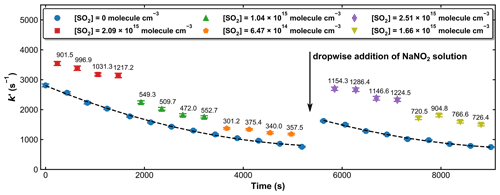
Figure 7 Pseudo-first-order rate coefficients (k^′) at 193.2 Torr and 298 K, whereby OH was generated by the 351 nm photolysis of HONO. The time-dependent OH decay constants in the absence of SO₂ (blue symbols) were fit to a second-degree polynomial (dashed black lines). The number on the top of each data point when SO₂ is present (red-, green-, orange-, purple-, and olive-coloured symbols) represents the difference in k^′ to that obtained (by interpolation) in the absence of SO₂. A few drops of the NaNO₂ solution were re-added after about 90 min to maintain OH levels and thus a good signal-to-noise ratio.

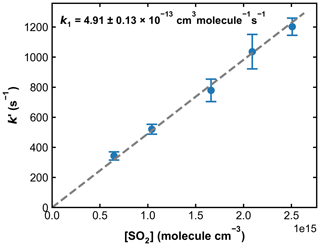
Figure 8 Pseudo-first-order rate coefficients (k^′) as a function of the SO₂ concentration ([SO₂]) at 193.2 Torr N₂ and 298 K. OH was generated through HONO photolysis at 351 nm. Each data point is an average over four individual measurements, and the error bars represent 2σ statistical uncertainties. k₁ was obtained from the slope of the linear regression.

Our measurements of k₁ obtained in N₂ bath gas (in total >100) are plotted against the N₂ concentration, at five different temperatures in Fig. 9. The rate coefficients and associated experimental conditions are listed in Table S1 in the Supplement. The rate coefficients obtained using H₂O₂ and HNO₃ as OH precursors (at 273 and 298 K) are indistinguishable from each other, indicating that the values obtained are not influenced by secondary chemistry. The overall uncertainty in k₁ (using H₂O₂ and HNO₃ precursors for OH) is estimated to be ∼ 7 %, which takes into account estimated systematic bias in the SO₂ cross-section at 213.86 nm.

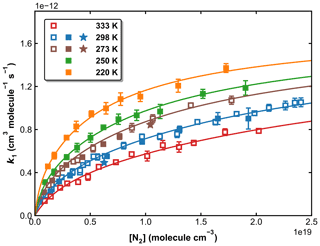
Figure 9 k₁ as a function of N₂ concentration in the fall-off regime at five different temperatures. Open squares, closed squares, and stars represent data obtained using H₂O₂, HNO₃, and HONO as OH precursors, respectively. The error bars represent 2σ statistical uncertainties. The solid lines are the fits of the experimental data to Eq. (3) with $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}=\mathrm{3.03}$ × 10⁻³¹ cm⁶ molecule⁻² s⁻¹, $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.00}$ × 10⁻¹² cm³ molecule⁻¹ s⁻¹, n=4.10, m=0 and F_C=0.58.

The solid lines are fits to the experimental data according to the Troe formalism for termolecular reactions (Troe, 1983).

$$\begin{array}{}\text{(3)}& k_{\mathrm{1}}\left(T,p\right)={\displaystyle \frac{k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}\left[\mathrm{M}\right]k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}}{k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}\left[\mathrm{M}\right]+k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}}}F,\end{array}$$

where $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}$ (cm⁶ molecule⁻² s⁻¹) and $k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}$ (cm³ molecule⁻¹ s⁻¹) are the high-pressure and low-pressure limiting rate coefficients, respectively, T is the temperature (K), [M] is the molecular density (molecule cm⁻³), and n and m are temperature exponents. The broadening factor F (which accounts for the lower rate coefficients measured in the fall-off regime than predictions by the Lindemann–Hinshelwood mechanism) is expressed as

$$\begin{array}{}\text{(4)}& \log F={\displaystyle \frac{\log F_{\mathrm{C}}}{\mathrm{1}+\left[\log \right(\frac{k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}\left[\mathrm{M}\right]}{k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}})/N{]}^{\mathrm{2}}}},\end{array}$$

where $N=\mathrm{0.75}-\mathrm{1.27}\log F_{\mathrm{C}}$, and F_C is the broadening factor at the centre of the fall-off curve.

In order to reduce the number of variables when fitting, we assume that k_1,∞ is independent of the temperature (m=0). This assumption is reasonable as m is expected to be small, and the data at high pressures (neither in this work nor in the literature) do not accurately define this parameter. If all remaining variables ($k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$, k_1,∞, F_C, and m) are allowed to float, the least-squares optimization using Eqs. (3) and (4) gives $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}=\mathrm{3.03}\times {\mathrm{10}}^{-\mathrm{31}}$ cm⁶ molecule⁻² s⁻¹, $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.00}$ × 10⁻¹² cm³ molecule⁻¹ s⁻¹, F_C=0.58, and n=4.10 (see Table 2, Method 1). These parameters, with a small residual standard deviation (RSD) of 2.27 × 10⁻¹⁴ cm³ molecule⁻¹ s⁻¹, accurately reproduce all our measurements in N₂ bath gas, as shown in Fig. 9.

Table 2 Parameterization of k₁ in N₂.

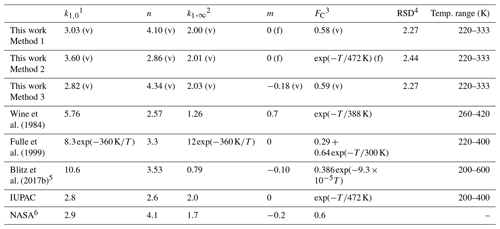
¹ Units of 10⁻³¹ cm⁶ molecule⁻² s⁻¹. ² Units of 10⁻¹² cm³ molecule⁻¹ s⁻¹. ³ Temperature (T) in kelvin. ⁴ RSD (residual standard deviation with the unit of 10⁻¹⁴ cm³ molecule⁻¹ s⁻¹) for the fitting is defined as $\left(\mathrm{\Sigma }\right(k\mathrm{1}-k_{{\mathrm{1}}_p}{)}^{\mathrm{2}}/(N-\mathrm{2}){)}^{\mathrm{0.5}}$, where k₁ and $k_{{\mathrm{1}}_p}$ are the measured and the fitted rate coefficients, and N is the total number of the data points. (v) denotes parameters allowed to vary during fitting, (f) denotes parameters fixed during fitting. ⁵ The parameters, which are different from those given in Blitz et al. (2017b), are from a personal communication with Mark Blitz (2022). The parameterization is based on the modified fall-off parameterization in Troe and Ushakov (2014); details are given in the Supplement. ⁶ The simplified form of the Troe expression for termolecular reactions used by NASA can be found in the Supplement.

As the range of temperatures encountered in the Earth's atmosphere is relatively narrow, temperature-dependent forms of F_C are no longer widely used in IUPAC evaluations, though a value of $F_{\mathrm{C}}=\exp (-T/\mathrm{472}\mathrm{K})$ is still recommended for the reaction between OH and SO₂. We therefore also explored the effect of setting F_C to $\exp (-T/\mathrm{472}\mathrm{K})$ and allowing a smaller set of variables, $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$, k_1,∞, and n, to float while fitting. This results in a 20 % higher $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$ of 3.60 × 10⁻³¹ cm⁶ molecule⁻² s⁻¹, an almost identical value of $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.01}$ × 10⁻¹² cm³ molecule⁻¹ s⁻¹ and n=2.86 (Table 2, Method 2). In addition, we examined the effect of varying the parameter m (i.e. making k_1,∞ temperature-dependent) while F_C was varied (but kept temperature-independent). In this case we obtained $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.03}$ × 10⁻¹² cm³ molecule⁻¹ s⁻¹ with $m=-\mathrm{0.18}$ and a lower value of $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$ (2.82 × 10⁻³¹ cm⁶ molecule⁻² s⁻¹) with n=4.34 (Table 2, Method 3).

The quality of the fits obtained using Methods 1, 2, or 3 is very similar (see values for the residual standard deviation and correlation coefficients in Table 2), as highlighted in Fig. S1 of the Supplement, where pressure- and temperature-dependent values of k₁ calculated using all three methods are plotted, along with the experimental data. We also show in Fig. S2 a plot of k₁ derived using each method versus altitude with the appropriate altitude-dependent change in temperature and pressure for a standard atmosphere. Clearly, Methods 1 and 3, which have the lowest residual standard deviations, are in excellent agreement throughout the atmosphere, with slight differences to Method 2 in the stratosphere. The pressure and temperature dependence of k₁ cancels each other in the lowest 10 km of the Earth's atmosphere, so that k₁ is roughly constant at a value close to 1 × 10⁻¹² cm³ molecule⁻¹ s⁻¹, which is about 5 % higher than the IUPAC and NASA recommendations.

For the purpose of modelling the Earth's atmosphere, it is more important to ensure that the data at low and intermediate pressures and temperatures are correctly reproduced by the parameterization, with correct definition of k_1,∞ of secondary importance. For this reason, we have chosen to use the parameters derived in Method 1 to calculate k₁ and for comparison with previous datasets and evaluations.

3.3 Comparison with previous parameterizations for N₂ bath gas

Despite the importance of the title reaction in the atmosphere there were no prior temperature-dependent measurements of k₁ in atmospherically relevant bath gases. In experiments designed to define k_1,0, low-pressure flow-tube studies (Leu, 1982; Lee et al., 1990) measured values of k₁ at N₂ pressures between 0.6 and 5 Torr. In order to access k_1,∞, Fulle et al. (1999) performed experiments in He bath gas at pressures of up to 96 bar. However, under all experimental conditions investigated so far, the title reaction is still in the fall-off regime, and neither high- nor low-pressure limits for the title reactions have been attained directly, experimentally.

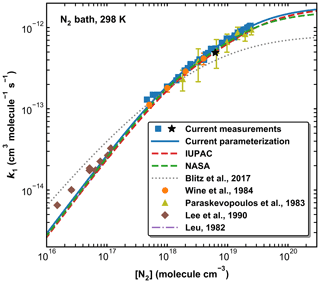
Figure 10 A comparison of previous measurements of k₁ at 298 K (N₂ bath gas only) with our parameterization and those of IUPAC, NASA, and Blitz et al. (2017b) (see Table 2 for details). The black star represents the measurement using HONO photolysis at 351 nm to generate OH.

In Table 2 we compare our values of k₀, k_∞, F_C, n, and m obtained in N₂ with the IUPAC and NASA expressions (both for N₂ bath gas), as well as parameterizations reported by previous studies (Wine et al., 1984; Fulle et al., 1999; Blitz et al., 2017b), which were mostly based on temperature-dependent measurements in other bath gases. The value of $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$ derived in this work (Method 1) is close to that of 2.8 × 10⁻³¹ and 2.9 × 10⁻³¹ cm⁶ molecule⁻² s⁻¹ preferred by IUPAC and NASA, respectively, and the current value of k_∞ is identical to the IUPAC recommendation of 2.0 × 10⁻¹² cm³ molecule⁻¹ s⁻¹; The current value of n is equal to the value of 4.1 used in the NASA expression but larger than the IUPAC preferred value of 2.6 unless we adopt the temperature-dependent value for F_C which IUPAC uses. In that case our value of n=2.86 is close to that of IUPAC. This is readily understood as our value of F_C=0.58 is very close to the “standard” NASA value of F_C=0.6.

Figure 10 provides a comparison between our data points and parameterization (Method 1) with the literature data at 298 K obtained in N₂ (Leu, 1982; Lee et al., 1990; Paraskevopoulos et al., 1983; Wine et al., 1984) and with the IUPAC and NASA evaluations. In Fig. S3 of the Supplement we plot the ratios of literature rate constants obtained at 298 K in N₂ to our parameterization. Also presented in Fig. 10 is a modified parameterization based on Blitz et al. (2017b) (master equation analyses of the Paraskevopoulos et al., 1983, and Wine et al., 1984, datasets). Note that the curve plotted cannot be reproduced using the parameters listed in Table 3 of Blitz et al. (2017b) but is based on data (listed in Table 2) sent in a personal communication with Mark Blitz (2022) and takes care of various errors in the published analysis. The comparison in N₂ is restricted to 298 K as, prior to this study, all temperature-dependent studies were performed in SiF₆, He, or Ar.

In the common pressure range (in the fall-off regime), our values of k₁ agree very well with those reported by Paraskevopoulos et al. (1983) and Wine et al. (1984). The fall-off curves described by our parameterization and the IUPAC and NASA recommendations are very similar over this pressure range, though our parameterization gives a slightly higher value of k₁ (298 K, 1 atm) (1.04 × 10⁻¹² cm³ molecule⁻¹ s⁻¹) than the values proposed by IUPAC and NASA (9.38 and 9.50 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹, respectively). In this context, we note that the value of k_1,∞ chosen by IUPAC was a compromise between the rate coefficients measured at extended pressures of He by Fulle et al. (1999) and the data from Blitz et al. (2017a). These values are however very divergent, with $k_{\mathrm{1},\mathrm{\infty }}=$ 3.6 × 10⁻¹² cm³ molecule⁻¹ s⁻¹ and $k_{\mathrm{1},\mathrm{\infty }}=$ 7.5 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹, respectively. We show below that at 220 K, our value of k₁ in 400 Torr of N₂ (13.71±0.41 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹), while clearly still in the fall-off regime, is a factor of 2 larger than the value of $k_{\mathrm{1},\mathrm{\infty }}=$ 7.5 × 10⁻¹³ cm³ molecule⁻¹ s⁻¹ reported by Blitz et al. (2017a). Similarly, our value obtained using 351 nm photolysis of HONO as OH source (i.e. when SO₂ excitation can be ruled out) results in $k_{\mathrm{1}}=\mathrm{8.44}\pm \mathrm{0.19}$ × 10⁻¹³ cm³ molecule⁻¹ s⁻¹ in 295 Torr of N₂ at 273 K, again above the high-pressure limit reported by Blitz et al. (2017a). Blitz et al. (2017b) suggested that the larger literature values of k_1,∞ available at the time of their study were a result of photoexcitation/dissociation of SO₂. However, our results have clearly shown that (a) such effects are absent in N₂ bath gas when using 248 nm photolysis to generate OH and (b) can be ruled out when working at 351 nm (see above). The excellent agreement between the present rate coefficients and those obtained previously in N₂ at 298 K (Paraskevopoulos et al., 1983; Wine et al., 1984) using completely different OH generation methods strongly suggests that data in N₂ are unaffected by such processes, though we cannot rule out that they are the cause of the large values of k_1,∞ obtained by Fulle et al. (1999) in He. The present and previous datasets in N₂ indicate that k_1,∞ is close to 2 × 10⁻¹² cm³ molecule⁻¹ s⁻¹.

At lower pressures, the IUPAC, NASA, and present parameterizations capture the flow-tube measurements at 1 Torr and above (Leu, 1982; Lee et al., 1990), whereas at the very lowest pressures, there is substantial deviation. This is likely to reflect bias in the flow-tube data caused, for example, by wall losses of OH and large corrections for axial diffusion.

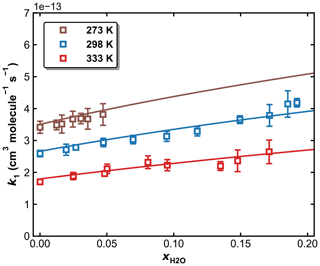
Figure 11 k₁ as a function of $x_{{\mathrm{H}}_{\mathrm{2}}O}$ in N₂–H₂O bath gas at a total pressure of 50 Torr and different temperatures of 273, 298, and 333 K. The symbols represent measurements, and the solid lines are fits to Eqs. (5) and (6), with $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.00}$ × 10⁻¹² cm⁶ molecule⁻² s⁻¹, $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}=\mathrm{3.03}$ × 10⁻³¹ cm⁶ molecule⁻² s⁻¹, n=4.10, m=0, and F_C=0.58. The resulting parameters for water are $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{1.65}$ × 10⁻³⁰ cm⁶ molecule⁻² s⁻¹, o=4.90.

3.4 Influence of water vapour on k₁

To examine the effects of water vapour (e.g. as a third-body quenching agent) on the kinetics of the title reaction, k₁ was measured in N₂–H₂O mixtures at 273, 298, and 333 K. A total pressure of 50 Torr (N₂+ H₂O) was chosen for these experiments as sensitivity to a potential increase in k₁ through the presence of water is highest at conditions far from k₁,_∞. As described in Sect. 2.2, both SO₂ (at 213.856 nm) and H₂O (at 184.95 nm) were monitored online by optical absorption. The large absorption cross-section of HNO₃ at 184.95 nm (1.61 × 10⁻¹⁷ cm² molecule⁻¹) (Dulitz et al., 2018) would hinder the accurate determination of the H₂O concentration, and H₂O₂ was therefore used as the OH precursor in all measurements in N₂–H₂O bath gas. The molar H₂O mixing ratio in N₂ ($x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}$) was varied up to 0.2 for measurements at 298 and 333 K and up to 0.05 at 273 K to avoid condensation of water in the reactor and optical absorption cells. The results are summarized in Table S2, and measured values of k₁ are plotted versus $x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}$ in Fig. 11, in which k₁ is seen to increase with the water content of the bath gas, indicating that H₂O is a more efficient third-body quencher than N₂ for the title reaction. This is illustrated in Fig. 12 where we plot the fall-off curves for k₁ in N₂ and H₂O bath gases. We note that, as for examining the water-vapour effect in the OH + NO₂ reaction (Amedro et al., 2020), continuous, in situ measurement of the SO₂ concentration is critical to obtaining the correct result as SO₂ concentrations (i.e. optical density at 213.86 nm) decreased when H₂O was added to the flowing ${\mathrm{H}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{2}}/{\mathrm{SO}}_{\mathrm{2}}/{\mathrm{N}}_{\mathrm{2}}$ mixture. This presumably reflects losses of SO₂ on the glass surfaces of the apparatus on which H₂O would have been adsorbed.

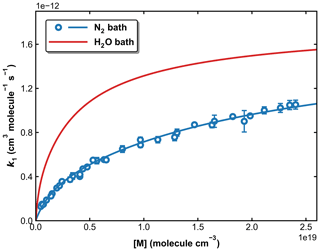
Figure 12 Fall-off curves for k₁ in N₂ and H₂O bath gases at 298 K. The solid lines are our preferred parameterization with $k_{\mathrm{1},\mathrm{\infty }}=\mathrm{2.0}$ × 10⁻¹² cm³ molecule⁻¹ s⁻¹ (independent of bath gas), $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}=\mathrm{3.03}$ × 10⁻³¹ cm⁶ molecule⁻² s⁻¹, and $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{1.65}$ × 10⁻³⁰ cm⁶ molecule⁻² s⁻¹, $F_{\mathrm{C}}^{{\mathrm{N}}_{\mathrm{2}}}=F_{\mathrm{C}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{0.58}$.

To evaluate the role of water in the rate coefficient of the title reaction, a parameterization of the third-body effect of H₂O is required, and we adopt the approach used in Amedro et al. (2020) for the OH + NO₂ reaction.

Extending Eq. (3), k₁ in the N₂–H₂O mixture can be expressed by

$$\begin{array}{}\text{(5)}& \begin{array}{rl}& k\left(T,p\right)=\\ & {\displaystyle \frac{\left(x_{{\mathrm{N}}_{\mathrm{2}}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}+x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-o}\right)\left[M\right]k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}}{\left(x_{{\mathrm{N}}_{\mathrm{2}}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}+x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-o}\right)\left[M\right]+k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}}}F,\end{array}\end{array}$$

where $x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}$ and $x_{{\mathrm{N}}_{\mathrm{2}}}$ are the mole fraction of H₂O and N₂, $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}$ is the low-pressure limiting rate coefficients (cm⁶ molecule⁻² s⁻¹) in pure H₂O, and o is a dimensionless temperature exponent. The broadening factor F is then

$$\begin{array}{}\text{(6)}& \begin{array}{rl}& \log F=\\ & {\displaystyle \frac{\log F_{\mathrm{C}}}{\mathrm{1}+{\left[\log \left(\frac{x_{{\mathrm{N}}_{\mathrm{2}}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-n}+x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{k}_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-o}\left)\right[M]}{k_{\mathrm{1},\mathrm{\infty }}{\left(\frac{T}{\mathrm{300}\mathrm{K}}\right)}^{-m}}\right)/N\right]}^{\mathrm{2}}}}.\end{array}\end{array}$$

Here, the low-pressure limiting rate coefficients in pure N₂ and pure H₂O are linearly mixed, and the same value of F_C is assumed for simplification. By inputting the values of $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{N}}_{\mathrm{2}}}$, k_1,∞, F_C, and n listed in Table 2 (Method 1), a multivariate, least-squares fit (solid lines in Fig. 9) results in $k_{\mathrm{1},\mathrm{0}}^{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{1.65}$ × 10⁻³⁰ cm⁶ molecule⁻² s⁻¹ and o=4.90, indicating that H₂O, as a third-body collider, is at least 5 times more efficient than N₂.

We also consider the use of different values of F_C for N₂ and H₂O, which may be more appropriate for bath gases with distinctly different properties (Burke and Song, 2017) and adopted for the OH+NO₂ reaction in He–H₂O mixtures (Amedro et al., 2020). In the present case, however, F_C for N₂ is already close to 0.6, so the differences are expected to be small, and the use of the more complex expression for the purpose of atmospheric modelling of the reaction is not warranted. This is detailed in the Supplement.

3.5 Atmospheric modelling of the OH + SO₂ reaction including the effect of water vapour

The chemistry and climate simulation model used is EMAC (ECHAM-MESSy), which uses the fifth-generation European Centre Hamburg general circulation model (ECHAM5; Roeckner et al., 2006) as core atmospheric general circulation model (Jöckel et al., 2006, 2010). In this study we used EMAC (ECHAM5 version 5.3.02, MESSy version 2.55.0) at T63L47MA-resolution, i.e. with a spherical truncation of T63 (∼ 1.8 by 1.8^∘ in latitude and longitude), with 47 vertical hybrid-terrain-following pressure levels up to 0.01 hPa. The model was weakly nudged in spectral space, applying Newtonian relaxation of the parameters temperature, vorticity, divergence, and surface pressure to meteorological reanalysis data (Jeuken et al., 1996). The model setup is identical to the simulation RED presented in Reifenberg et al. (2021), where the model was evaluated against an aircraft campaign over Europe. In addition, the model has been evaluated on many occasions (Pozzer et al., 2012a, b; Yan et al., 2019). For additional references, see http://www.messy-interface.org (last access: 2021). As in Reifenberg et al. (2021), EMAC was used in a chemical-transport model (Deckert et al., 2011) without feedbacks between photochemistry, radiation, and atmospheric dynamics. In this work, we performed three identical simulations for the year 2019 but with three different parameterizations of k₁ from this work and from the IUPAC and NASA evaluations panels. For the simulations we assume that O₂ has the same collisional quenching efficiency as N₂ as is for the case for nearly all termolecular processes of atmospheric importance.

In Fig. 13, we illustrate the impact of H₂O vapour on the rate coefficient, by plotting the reduction in k₁ at the Earth's surface (lowest 1–2 km of the atmosphere) when setting $x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}$ to zero relative to using EMAC water-vapour fields. The greatest effect of water vapour on k₁ is found in warm, tropical regions, where an average underestimation of the rate coefficient by up to ≈5 % is found when $x_{{\mathrm{H}}_{\mathrm{2}}\mathrm{O}}=\mathrm{0}$. At higher/lower latitudes, the effect is diminished, and water vapour accounts for only a few percent of the overall rate coefficient at 40^∘ N/S. The presence of water vapour does not impact values of k₁ above the boundary layer significantly.

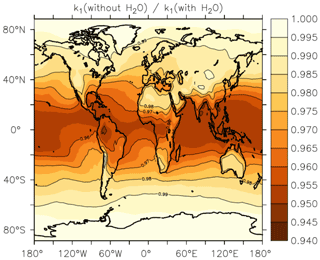
Figure 13 Annual, average effect of H₂O on k₁ expressed as the fractional change in the rate coefficient near the Earth's surface (altitude of 1–2 km) when setting the mole fraction of water vapour to zero in Eqs. (5) and (6).

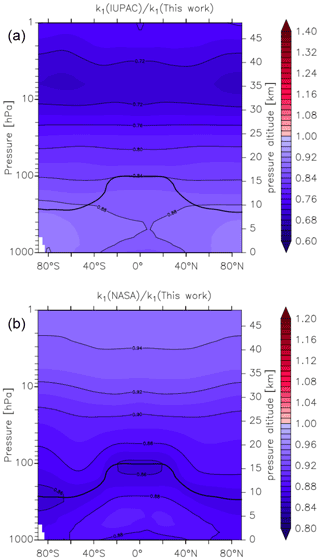
Figure 14 Global values of $\frac{k_{\mathrm{1}}\left(\mathrm{IUPAC}\right)}{k_{\mathrm{1}}\left(\mathrm{this}\mathrm{work}\right)}$ (a) and $\frac{k_{\mathrm{1}}\left(\mathrm{NASA}\right)}{k_{\mathrm{1}}\left(\mathrm{this}\mathrm{work}\right)}$ (b). k₁ was calculated using the parameters from this work and those presently recommended by the IUPAC and NASA data evaluation panels. The relatively thick black line between 300 and 100 hPa represents the mean model tropopause.

In Fig. 14 we compare our new parameterization with preferred parameterizations of the IUPAC and NASA evaluation panels and plot values of k₁(IUPAC) $/$ k₁ (this work) and k₁(NASA) $/$ k₁ (this work) at different altitudes and latitudes. We parameterized k₁ using the expressions given in this work (Eq. 5, Table 2) and in the latest evaluations of IUPAC (IUPAC, 2021) and NASA (Burkholder et al., 2020). k₁(IUPAC) $/$ k₁ (this work) varies between 0.88 close to the Earth's surface to 0.72 at altitudes above ∼ 30 km, whereas k₁(NASA) $/$ k₁ (this work) varies between 0.88 at the Earth's surface to 0.92 at ∼ 30 km. Thus, while both evaluations under-predict k₁ by ≈12 % at the Earth's surface (a combined consequence of their lower values of k₁ in N₂ (dry air) and neglecting water-vapour effects), the NASA parameterization does well in the lower stratosphere (under-predicting our result by less than 10 %), whereas the IUPAC parameters result in a rate coefficient that is too low by almost 30 %. At high altitudes, the divergent rate coefficients recommended by the evaluation panels reflect the choice of experimental data used to derive the low-pressure limiting rate coefficient and its temperature dependence.

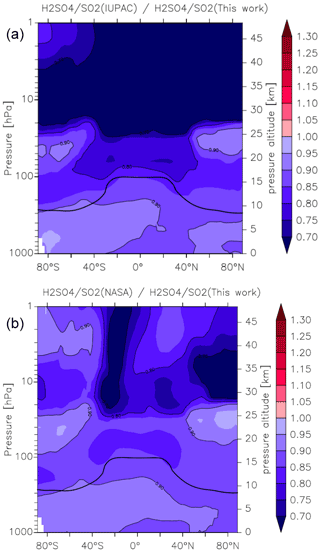
Figure 15 Effect of different parameterizations of k₁ on the global (zonal and yearly averaged) H₂SO₄ to SO₂ ratio. Panel (a) plots $\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}\left(\mathrm{IUPAC}\right)/\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}$ (this work), and panel (b) plots $\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}\left(\mathrm{NASA}\right)/\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}$ (this work). The relatively thick black line between 300 and 100 hPa represents the mean model tropopause.

As the reaction between OH and SO₂ ultimately results in the formation of H₂SO₄, the atmospheric ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{SO}}_{\mathrm{2}}$ ratio is sensitive to the rate coefficient k₁, with an increase in k₁ resulting in a decrease in SO₂ and an increase in H₂SO₄, thus amplifying the impact. In Fig. 15 we plot zonally and yearly averaged model values of $\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}\left(\mathrm{IUPAC}\right)/\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}$ (this work) and $\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}\left(\mathrm{NASA}\right)/\frac{{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}}{{\mathrm{SO}}_{\mathrm{2}}}$ (this work). Compared to the parameterization of k₁ in this work, the IUPAC evaluation returns ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{SO}}_{\mathrm{2}}$ ratios that are close to 0.9 at the Earth's surface but decrease to 0.7 in the lower to mid-stratosphere at low latitudes. Again, the NASA parameterization performs somewhat better, though here we also find an underestimation of the ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{SO}}_{\mathrm{2}}$ ratio of between 10 % and 20 % throughout most of the atmosphere. The impact on the H₂SO₄ to SO₂ ratio is thus similar to the change in the rate coefficients, so that the expected amplification is not observed in the model. This is related to an increase in the sink term of H₂SO₄ (via nucleation), which counteracts the increase in its production rate.

The modelling studies indicate that use of IUPAC and NASA parameterizations results in very different values of k₁ in some parts of the atmosphere and will result in divergent predictions of partitioning of reactive sulfur gases between SO₂ and H₂SO₄. The present parameterization, based on precise and accurate temperature-dependent measurements in the fall-off regime in N₂, does not rely on potentially erroneous data at very low and very high pressures and is expected to lead to more accurate values of k₁ for modelling the title reaction in the Earth's atmosphere, both at the surface (where the effect of water vapour has been considered for the first time) and at the low pressures and temperatures prevalent in the upper troposphere–lower stratosphere (UT-LS) region.

4 Conclusions

Rate coefficients for the reaction of SO₂+OH (k₁) in fall-off regime were experimentally determined in a wide range of pressures and temperatures relevant to the atmosphere. More than 100 individual measurements for k₁ were carried out in N₂ and ${\mathrm{N}}_{\mathrm{2}}/{\mathrm{H}}_{\mathrm{2}}\mathrm{O}$ bath gases using pulsed laser photolytic (PLP) generation of OH coupled to real-time detection of OH via laser-induced fluorescence (LIF). The presence of water vapour was found to enhance the rate coefficient of the title reaction significantly, indicating that H₂O is a more efficient third-body collider than N₂ or O₂ (by a factor of >5). Based on our comprehensive dataset in the fall-off regime, we derived a new parameterization of the rate coefficient which results in values of k₁ that are larger than those preferred by the IUPAC and NASA panels, leading to a more rapid removal of SO₂ through gas-phase oxidation than previously assumed and thus to an underestimation of the ${\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}/{\mathrm{SO}}_{\mathrm{2}}$ ratio in nearly all regions of the Earth's atmosphere.