Measurement report: Nitrogen isotopes (δ¹⁵N) and first quantification of oxygen isotope anomalies (Δ¹⁷O, δ¹⁸O) in atmospheric nitrogen dioxide

The isotopic composition of nitrogen and oxygen in nitrogen dioxide (NO₂) potentially carries a wealth of information about the dynamics of the nitrogen oxides (NO_x = nitric oxide (NO) + NO₂) chemistry in the atmosphere. While nitrogen isotopes of NO₂ are subtle indicators of NO_x emissions and chemistry, oxygen isotopes are believed to reflect only the O₃ $/$ NO_x $/$ VOC chemical regime in different atmospheric environments. In order to access this potential tracer of the tropospheric chemistry, we have developed an efficient active method to trap atmospheric NO₂ on denuder tubes and measured, for the first time, its multi-isotopic composition (δ¹⁵N, δ¹⁸O, and Δ¹⁷O). The Δ¹⁷O values of NO₂ trapped at our site in Grenoble, France, show a large diurnal cycle peaking in late morning at (39.2 ± 0.3) ‰ and decreasing at night until (20.5 ± 0.3) ‰. On top of this diurnal cycle, Δ¹⁷O also exhibits substantial daytime variability (from 29.7 ‰ to 39.2 ‰), certainly driven by changes in the O₃ to peroxyl radicals (RO₂) ratio. The nighttime decay of Δ¹⁷O(NO₂) appears to be driven by NO₂ slow removal, mostly from conversion into N₂O₅, and its formation from the reaction between O₃ and freshly emitted NO. As expected from a nighttime Δ¹⁷O(NO₂) expression, our Δ¹⁷O(NO₂) measured towards the end of the night is quantitatively consistent with typical values of Δ¹⁷O(O₃). Daytime N isotope fractionation is estimated using a general expression linking it to Δ¹⁷O(NO₂). An expression is also derived for the nighttime N isotope fractionation. In contrast to Δ¹⁷O(NO₂), δ¹⁵N(NO₂) measurements exhibit little diurnal variability (−11.8 ‰ to −4.9 ‰) with negligible isotope fractionations between NO and NO₂, mainly due to high NO₂ $/$ NO_x ratios, excepted during the morning rush hours. The main NO_x emission sources are estimated using a Bayesian isotope mixing model, indicating the predominance of traffic emissions in this area. These preliminary results are very promising for using the combination of Δ¹⁷O and δ¹⁵N of NO₂ as a probe of the NO_x sources and fate and for interpreting nitrate isotopic composition records.

1 Introduction

Nitrogen oxides (NO_x = NO₂ + NO) are at the heart of tropospheric chemistry, as they are involved in key reaction chains governing the production and destruction of compounds of fundamental interest for health, ecosystems, and climate issues (Brown, 2006; Finlayson-Pitts and Pitts, 2000; Jacob, 1999). For example, NO₂ photolysis followed by reaction of NO with peroxy radicals (RO₂ = HO₂ + RO₂) is the only significant source of ozone (O₃) in the troposphere, where it serves as a severe air pollutant and a greenhouse gas. Tropospheric O₃ also plays a major role in the production processes of radicals which are responsible for the oxidation and removal of compounds emitted into the atmosphere (Crutzen, 1996). This “cleaning” ability is referred to as the atmospheric oxidative capacity (AOC; Prinn, 2003). Additionally, NO_x species are at the core of the reactive nitrogen cycle as precursors of atmospheric nitrate (particulate NO${}_{\mathrm{3}}^{-}$ + gaseous HNO₃), which contributes to soil acidification and eutrophication (Galloway et al., 2004), as well as aerosol radiative forcing (Liao and Seinfeld, 2005). In order to better understand the reactive nitrogen (which includes NO_x and HNO₃) chemistry, the related AOC, and the contributions of precursor emissions to nitrate deposition, it is necessary to better constrain NO_x emission sources and individual oxidation processes.

Stable isotope analysis is a powerful tool for tracing emission sources, individual chemical mechanisms, and budgets of atmospheric trace gases (Kaye, 1987). Because physico-chemical and biological processes favour lighter or heavier isotopologues, the isotopic composition of a chemical species will often vary according to its formation pathway. This phenomenon of isotopic fractionation can thus be used to trace different processes involved in the formation of the chemical species being analysed. Isotopic enrichment (δ) of an element X is expressed in per mill (‰) and defined as ${\mathit{\delta }}^{n}X={(}^n{R}_{\mathrm{spl}}{/}^n{R}_{\mathrm{ref}}-\mathrm{1})$ with ⁿR being the elemental isotope abundance ratio of the heavy isotope over the light isotope (e.g. for oxygen isotopes ¹⁸R(¹⁸O $/$ ¹⁶O) ≡ ¹⁸R = x(¹⁸O) $/$ x(¹⁶O) or ¹⁷R(¹⁷O $/$ ¹⁶O) ≡ ¹⁷R = x(¹⁷O) $/$ x(¹⁶O), with x being the isotopic abundance) in a sample (ⁿR_spl) and in a reference (ⁿR_ref). The Vienna Standard Mean Ocean Water (VSMOW; Li et al., 1988) and atmospheric nitrogen (N₂; Mariotti, 1984) are the international references for oxygen and nitrogen ratios, respectively. Most natural isotopic fractionations are mass-dependent fractionations (MDFs; Urey, 1947), as is notably the case for terrestrial oxygenated species in which the triple oxygen composition follows δ¹⁷O ≈ 0.52 × δ¹⁸O (Thiemens, 1999). Yet, laboratory experiments (Thiemens and Heidenreich, 1983) and atmospheric observations (Johnston and Thiemens, 1997; Krankowsky et al., 1995; Vicars and Savarino, 2014) have shown that the isotopic composition of ozone formed in the atmosphere does not follow this canonical MDF relationship and reflects mass-independent fractionation (MIF) processes. The important deviation from the MDF oxygen relationship is called the oxygen-17 anomaly (Δ¹⁷O) and is defined here in its approximate linearized form as Δ¹⁷O = δ¹⁷O − 0.52 × δ¹⁸O. Our choice of this linear definition is mainly motivated by its convenience for mass-balance calculations and its validity for our large Δ¹⁷O values and variability. Overall, biases related to our choice of the linear definition are marginal in our conditions (Assonov and Brenninkmeijer, 2005). It follows that Δ¹⁷O inherited from ozone can be considered conserved during MDF processes.

The multi-isotopic composition of NO_x is therefore a very valuable tracer of its emissions and chemistry in the atmosphere. However, so far, Δ¹⁷O of atmospheric NO₂ (Δ¹⁷O(NO₂)) has been investigated only using laboratory (Michalski et al., 2014) and modelling (Alexander et al., 2020, 2009; Lyons, 2001; Morin et al., 2011) approaches with theoretical frameworks, and these results need to be tested against atmospheric observations. Walters et al. (2018) have presented a method of sampling and analysing nitrogen and oxygen stable isotopes of NO₂ collected separately at daytime and nighttime in an urban area, but they did not report on Δ¹⁷O. Dahal and Hastings (2016) have attempted to measure Δ¹⁷O of NO₂ collected on passive samplers, but the isotopic signal was partly degraded during the sampling and the analytical procedure. Building on their work, we present here an efficient method to collect atmospheric NO₂ for isotopic analysis and present the first measurements of triple oxygen isotopes and double nitrogen isotopes of atmospheric NO₂. Combined with mass-balance equations, oxygen isotopes are used to investigate the links between the variability of the oxygen isotope anomaly of NO₂ and its formation pathways. We also revisit the Morin et al. (2011) NO_x isotopic theoretical framework and extend it to urban environments. After estimating the nitrogen isotopic fractionation between NO and NO₂, we infer from δ¹⁵N of NO₂ (δ¹⁵N(NO₂)) the major emission sources of NO_x influencing our sampling site using an isotopic mixing model (Parnell et al., 2010).

2 Materials and methods

2.1 Sampling method

NO₂ was sampled on an active (pumped) collection system using denuder tubes. This method is more efficient to collect NO₂ than passive methods (Røyset, 1998), allowing for shorter collection times with a breakthrough of the absorption capacity below 1 % (Buttini et al., 1987; Williams and Grosjean, 1990). The sampled air was pumped through a ChemComb^® 3500 speciation cartridge (Thermo Scientific^®, USA). Initially used for the speciation of gases and aerosols, these advanced sampling platforms consist of a PM_2.5 impactor inlet connected to a stainless-steel cylinder that contains two glass honeycomb denuders connected in series for gas collection and a Teflon stage filter pack for aerosols. To collect NO₂, glass tubes were coated with an alkaline guaiacol solution. In basic medium, guaiacol (IUPAC name: 2-methoxyphenol) is known to react with NO₂ to form stable NO${}_{\mathrm{2}}^{-}$ ions (Nash, 1970), preserving the original NO₂ isotopic signal due to the basic nature of the medium (pH = 14 after 10 mL extraction). Because NO or peroxyacetyl nitrate (PAN) are not collected by guaiacol, this methodology avoids potential interferences from these compounds in later analyses (Buttini et al., 1987). Although nitrous acid (HONO) can bind as NO${}_{\mathrm{2}}^{-}$, it is unlikely to adversely impact the results as its mixing ratio (i.e. mole fraction) is much lower than NO₂ (by a factor of 10 to 20) even in very polluted cities (e.g. Harris et al., 1982; Michoud et al., 2014; Huang et al., 2017).

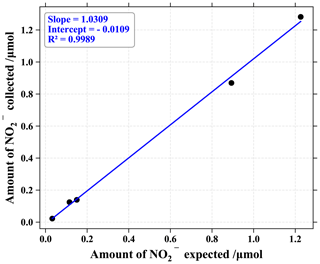
To evaluate the sampling system performance, a series of experiments were run with artificial gaseous NO₂. Using a commercial gas-standard generator (KinTek FlexStream^®) feed with zero air, diluted NO₂ (Metronics Dynacal^®) was sent through a ChemComb cartridge, while NO_x mixing ratio was measured upstream and downstream of the cartridge. From 1 to 30 nmol mol⁻¹ of NO₂ (representative of rural to urban atmospheric conditions), mixing ratios coming out of the cartridge were never above the noise level of the NO_x monitor (2.5 nmol mol⁻¹). To estimate the denuder's trapping efficiency, we passed different mixing ratios of gaseous NO₂ through the collection apparatus and measured the amount of NO${}_{\mathrm{2}}^{-}$ collected on the two denuders both connected in series. The denuder efficiency E was then calculated according to the following equation (Buttini et al., 1987):

$$\begin{array}{}\text{(1)}& E=\left(\mathrm{1}-{\displaystyle \frac{b}{a}}\right)\times \mathrm{100}\mathit{\%},\end{array}$$

with a and b representing the amount of NO${}_{\mathrm{2}}^{-}$ collected on the first and the second denuder, respectively. From 0.3 to 1.3 µmol of generated NO₂ (see Fig. 1), the mean E value was about (97 ± 3) %. The amount of NO${}_{\mathrm{2}}^{-}$ measured on the second denuder was reproducible and equivalent to blanks, representing on average 3 % of the quantity measured on the first denuder. In light of these results, the denuder in the second position was not subjected to isotopic analysis and allowed for trapping efficiency control.

Figure 1 Correlation plot of NO₂ collected on the first denuder tube of the sampling cartridge vs. NO₂ produced by the gas standard generator.

2.2 Isotopic analysis

Simultaneous isotopic analyses of δ¹⁵N, δ¹⁸O, and δ¹⁷O were performed using a Finnigan^® MAT253 isotope ratio mass spectrometer (IRMS) following techniques described by Casciotti et al. (2002) and Kaiser et al. (2007). The azide method (McIlvin and Altabet, 2005) was used with ≈ 100 nmol of nitrites converted to N₂O using a 50 : 50 by volume mixture of 2 M sodium azide and 100 % acetic acid. This chemical method has the advantage over the bacterial method to be free of nitrate interferences since HNO₃ is certainly trapped with NO₂ in the basic solution coating of the denuder tube. The principle of identical treatment (Brand, 1996) was strictly respected where the standards and samples possessed the same nitrite amount, water isotopes, total volume and matrix. Three international KNO₂ salt standards – RSIL-N7373, RSIL-N10219, and RSIL-N23 with respective δ¹⁵N $/$ δ¹⁸O values of −79.6/4.2 ‰, 2.8/88.5 ‰, and 3.7/11.4 ‰ – were used for normalization of the δ scale. Scale contraction factors were obtained with the linear regression between measured and known values of δ¹⁵N and δ¹⁸O. Although the three standards cover a wide range of isotopic composition in δ¹⁵N and δ¹⁸O, they do not have an isotopic anomaly in ¹⁷O. For the δ¹⁷O scale, MDF fractionation slope (0.52) is assumed for two of these laboratory-prepared nitrite standards (see Appendix A for more details). Accuracy of this analytical method on δ¹⁷O, δ¹⁸O, and δ¹⁵N measurements was estimated as the standard deviation (σ) of the residuals between our measurements of the RSIL standards and their expected values. Additionally, isotopic integrity from denuder's extraction to the analysis by IRMS has been investigated and showed no degradation over several weeks (see Appendix B), confirming that this method is suitable for isotopic analysis of NO₂, as first demonstrated by Walters et al. (2018). The uncertainties applied to our measurements of δ¹⁵N, δ¹⁷O, and δ¹⁸O are reported as the propagation error of the measurement uncertainty and the uncertainty resulting from sample storage. Uncertainty on Δ¹⁷O is derived from the propagation error of the overall uncertainty on δ¹⁷O and δ¹⁸O. In our study, average uncertainties on δ¹⁵N, δ¹⁷O, δ¹⁸O, and Δ¹⁷O are estimated to be ±0.1 ‰, ±0.8 ‰, ±1.8 ‰, and ±0.3 ‰, respectively (1σ uncertainties).

2.3 Study site and atmospheric NO₂ collection

Atmospheric NO₂ was collected at the Université Grenoble Alpes campus site. Located in the eastern Grenoble urban area (690 000 inhabitants), the campus stands between a major transportation route and the Isère river. The city is located at the confluence of three valleys surrounded by mountain chains that influence the atmospheric dynamics and the local air quality. During winter, persistent temperature inversions combined with intense domestic heating can lead to severe PM₁₀ pollution events (Largeron and Staquet, 2016) with daily-average mixing ratio above World Health Organization thresholds. In summer, emissions are mainly controlled by road traffic that can result in heightened ozone mixing ratios, especially during stagnant conditions.

Samplings were conducted on a platform 5 m above the ground surface. Ambient air was drawn through the cartridge with a Millipore vacuum pump at a flow rate of 10 L min⁻¹ (room temperature and one atmospheric pressure) adjusted using a Cole-Palmer^® flowmeter (accuracy ±3 %). In order to capture the daily variability in NO₂ isotopic composition, samples were collected during 24 h with 3 h sampling intervals during the day and 5 h sampling from midnight to 05:00 local time (LT)​​​​​​​. Ambient NO and NO₂ mixing ratios were measured with a 2B Technologies^® NO monitor (model 410) paired with a NO₂ converter (model 401).

Honeycomb denuders were cleaned and coated the day before sampling. After being generously rinsed (5 min under a stream of deionized water), the denuders were placed in a vacuum chamber (Thermo Scientific^® refrigerated vapour trap paired with a SpeedVac concentrator) and dried at 40 ^∘C during 1 h. Then, denuder's internal walls were individually coated with 10 mL of a 95 : 5 by volume mixture of 2.5 M KOH (in methanol) and ultrapure guaiacol prepared daily. Denuders were then drawn off, dried in the vacuum chamber at 40 ^∘C during 30 min to minimize blanks, hermetically sealed, and stored at ambient temperature in the dark until usage. The different components of the cartridge (impactor, filters, denuders) were cleaned, dried, and fitted together just before use. At the end of the sampling period, both denuders were removed from the ChemComb cartridge and rinsed with 10 mL of deionized water in order to leach trapped NO₂ out. A volume of 1 mL of the eluent was rapidly used to determine the nitrite amount using the Griess–Saltzman reaction and UV–Vis spectrometry at 544 nm. Recovered eluent (≈ 7 mL by denuder) was poured into a labelled 15 mL Corning^® tube and stored in a freezer until isotopic analysis the following days.

3 Atmospheric observations and multi-isotopic measurements

3.1 NO_x and O₃ atmospheric observations

Figure 2 shows the time evolution of the hourly NO₂, NO, and O₃ mixing ratios measured during the period covering two nights and 1 d (from 14 May 2019 21:00 LT to 16 May 2019 05:00 LT). Note that most of our NO measurements are found to be within the reported detection limit of the instrument except in the morning (see Table 1) and therefore have to be treated with a lot of caution. NO₂ mixing ratios during the sampling period ((6.1 ± 4.2) nmol mol⁻¹; mean ± 1 standard deviation) are in good agreement with the range of values measured at the local air quality site located a kilometre south of the sampling site (https://www.atmo-auvergnerhonealpes.fr/, last access: 18 August 2020).

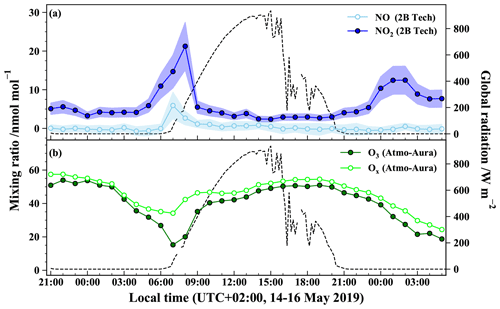
Figure 2 Temporal evolution of (a) NO (open circles) and NO₂ (closed circles) at the sampling site (the envelops represent ±1σ variations over 1 h) and of (b) O₃ (closed circles) and O_x (= O₃ + NO₂; open circles) at the air quality station during the sampling period. Markers represent for (a) the hourly mean derived from 1 min measurements and for (b) the hourly mean provided by the air quality monitoring station. Global solar radiation flux is represented by dashed lines (measured at 200 m from the sampling site by the IGE weather station with a Skye SP1110 pyranometer).

Table 1 Summary table of sampling periods (dates, local times), NO, NO₂, and O₃ mean mixing ratios over the collection periods, as well as calibrated NO₂ isotopic measurements of δ¹⁵N, δ¹⁸O, and Δ¹⁷O. All the sampling periods lasted 3 h except the last one that lasted 5 h. Averaged measurement uncertainties are provided just below the species names.

^∗ Data monitored at the local air quality monitoring site of Saint-Martin d'Hères located a kilometre south of the sampling site (https://www.atmo-auvergnerhonealpes.fr/).

During both nights, most of the NO_x is in the form of NO₂. After sunrise, there is a rapid interconversion between NO and NO₂, driven by NO₂ photolysis and reactions of NO with O₃ and peroxy radicals (Jacob, 1999). NO₂ levels are at a maximum on 15 May between 04:00 and 10:00 LT with a sharp peak of 21 nmol mol⁻¹ at 08:00 LT. After the morning rise, NO₂ decreases to reach a background mixing ratio of about (3.0 ± 0.5) nmol mol⁻¹. This diurnal variation is common in urban/suburban sites characterized by a morning peak caused by important NO_x emissions, mainly from road traffic (Mayer, 1999). As morning progresses, the boundary layer height increases rapidly, favouring fast dilution of NO_x mixing ratios. Moreover, during the day, NO₂ is converted to HNO₃, notably by its reaction with OH radicals. Thus, NO_x mixing ratio remains low during the day likely because of the combination of atmospheric dilution by vertical mixing and efficient chemical conversion by OH and organic radicals (Tie et al., 2007). In dense urban areas, a second NO_x traffic emission peak can occur in late afternoon, but it is not observed at our sampling site for that specific day. This surface pollution peak is usually weaker than the morning peak due to an elevated boundary layer and a longer period of evening commuting. After sunset, NO₂ mixing ratio increases gently and reaches a smooth peak with a maximum of 12 nmol mol⁻¹ around 01:00 LT, also recorded at the local air quality site. This NO₂ mixing ratio rise may be due to low NO emissions (converted to NO₂ by reaction with O₃) combined with a decreasing boundary layer height during the night which traps atmospheric species close to the surface (Tie et al., 2007; Villena et al., 2011).

Ozone also exhibits a diurnal variation typical of urban areas (Velasco et al., 2008). O₃ peaks around 50 nmol mol⁻¹ at the beginning of both nights and then declines continuously. Indeed, after sunset, O₃ production ceases, and its mixing ratio drops due to its dry deposition, reactions with organics, and O₃ titration by NO emitted from evening traffic, and industrial activities in the stable nocturnal boundary layer (Klein et al., 2019). O₃ reaches a minimum (about 15 nmol mol⁻¹) not at the end of the night but during the morning rush hour peak of NO. The O_x (= O₃ + NO₂) is a more conservative quantity than O₃, because it is less affected by conversion of O₃ into NO₂ through NO titration which is important in urban environments (Kleinman et al., 2002). For instance, between 06:00 and 08:00 LT, O₃ is strongly titrated by freshly emitted NO with its mixing ratio dropping to about 15 nmol mol⁻¹, while O_x reaches a moderate minimum of 34 nmol mol⁻¹. After this morning drop, O₃ recovers rapidly to about 46 nmol mol⁻¹ in the late morning, possibly caused by downward O₃ flux associated with the formation of the daytime thick boundary layer (Jin and Demerjian, 1993; Klein et al., 2019). During the rest of the day, O₃ and O_x keep increasing gently due to photochemical production and reach a close maximum at the end of the afternoon (Geng et al., 2008). After sunset, the important decline of both O₃ and O_x highlights the physical losses, notably O₃ deposition, and chemical loss of NO_x, typical of urban areas.

3.2 Multi-isotopic composition measurements of atmospheric NO₂

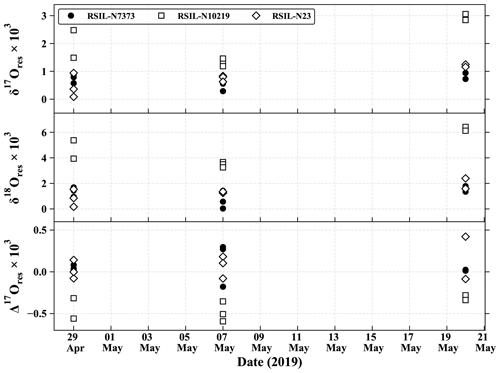
We present the data for the multi-isotopic composition of seven atmospheric NO₂ samples, while two additional samples were rejected as NO${}_{\mathrm{2}}^{-}$ amounts were too low to perform a reliable analysis. Table 1 reports ambient mean mixing ratios of NO, NO₂, and O₃ for the isotopic sampling intervals and corresponding measured NO₂ isotopic composition (δ¹⁵N(NO₂), δ¹⁸O(NO₂), and Δ¹⁷O(NO₂)). Figure 3 depicts the time series of measured δ¹⁵N, δ¹⁸O, and Δ¹⁷O of atmospheric NO₂. The temporal evolution of NO₂ oxygen and nitrogen isotopic composition is interpreted in the following section.

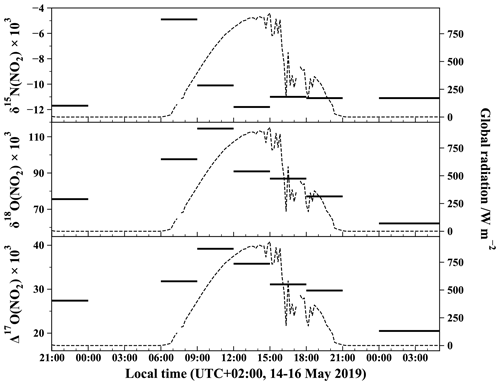
Figure 3 Temporal evolution of δ¹⁵N, δ¹⁸O, and Δ¹⁷O of atmospheric NO₂ measured with the azide method. Isotopic values for each 3 h slots are from the same NO₂ sample collected over 3 h (except for the last period which lasted 5 h). Global solar radiation flux is represented by dashed lines (measured at 200 m from the sampling site by the IGE weather station with a Skye SP1110 pyranometer).

4 Discussion of the multi-isotopic composition of atmospheric NO₂

4.1 Oxygen isotope composition

The time evolution of δ¹⁸O of atmospheric NO₂ (δ¹⁸O(NO₂)) shown in Fig. 3 exhibits a substantial diurnal variation with a daytime mean of (93.4 ± 13.9) ‰ and a nighttime mean of (68.9 ± 9.5) ‰. A maximum value of 114.5 ‰ is observed in the morning (09:00–12:00 LT interval) and a minimum value of 62.2 ‰ for the late-night interval (00:00–05:00 LT). Using a similar sampling apparatus during summer in the urban/suburban site of West Lafayette, USA, Walters et al. (2018) reported daytime and nighttime mean δ¹⁸O(NO₂) values of (86.5 ± 14.1) ‰ and (56.3 ± 7.1) ‰, respectively. Although our daytime values are higher than those of Walters et al. (2018), both datasets exhibit the same day–night contrast with a maximum during the day and a minimum at night. As expected from δ¹⁸O values, Δ¹⁷O(NO₂) follows a similar diurnal variation with a maximum value of 39.2 ‰ for the 09:00–12:00 LT interval and a minimum value of 20.5 ‰ for the 00:00–05:00 LT interval. High Δ¹⁷O values are expected to reflect the importance of ozone in the oxidation of NO to NO₂. Since daytime and nighttime chemistries are radically different, interpretations of our Δ¹⁷O measurements and their implications are discussed separately by day and night.

4.1.1 Fundamentals of NO_x chemistry and isotopic transfers

NO_x is mainly produced under the form of NO by combustion and lighting processes (Dennison et al., 2006; Young, 2002) as well as by the biological activity of soils (Davidson and Kingerlee, 1997). In the daytime, NO and NO₂ rapidly interconvert in a timescale of about 1–2 min, establishing a photostationary steady state (PSS; Leighton 1961):

$$\begin{array}{}\text{(R1)}& {\displaystyle }{\mathrm{NO}}_{\mathrm{2}}+h\mathit{\nu }\stackrel{M}{⟶}\mathrm{O}\left({}^{\mathrm{3}}\mathrm{P}\right)+\mathrm{NO},\text{(R2)}& {\displaystyle }\mathrm{O}\left({}^{\mathrm{3}}\mathrm{P}\right)+{\mathrm{O}}_{\mathrm{2}}\stackrel{M}{⟶}{\mathrm{O}}_{\mathrm{3}},\end{array}$$

with M=N₂ or O₂;

$$\begin{array}{}\text{(R3)}& \mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\to {\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\end{array}$$

This so-called null cycle can be disturbed by RO₂ radicals when NO_x mixing ratios are relatively high, typically above 30–100 pmol mol⁻¹ (Seinfeld and Pandis, 2006; Monks, 2005):

$$\begin{array}{}\text{(R4)}& \mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}\to {\mathrm{NO}}_{\mathrm{2}}+\mathrm{RO}.\end{array}$$

The reaction between NO and RO₂ competes with the NO + O₃ reaction, allowing NO₂ formation without the consumption of an ozone molecule in the cycle (Monks, 2005). This results in ozone production and can lead to severe ozone build-up in polluted areas. At night, RO₂ mixing ratios are strongly reduced making ozone the main NO oxidant following Reaction (R3).

NO_x is mainly removed from the atmosphere via the oxidation of NO₂ into nitric acid during the day,

$$\begin{array}{}\text{(R5)}& {\mathrm{NO}}_{\mathrm{2}}+\mathrm{OH}\stackrel{M}{⟶}{\mathrm{HNO}}_{\mathrm{3}},\end{array}$$

and at night,

$$\begin{array}{}\text{(R6)}& {\displaystyle }{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{3}}\stackrel{M}{⟶}{\mathrm{NO}}_{\mathrm{3}}+{\mathrm{O}}_{\mathrm{2}},\text{(R7)}& {\displaystyle }{\mathrm{NO}}_{\mathrm{3}}+{\mathrm{MO}}_{\mathrm{2}}\stackrel{M}{⟶}{\mathrm{N}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{5}}\stackrel{{\mathrm{H}}_{\mathrm{2}}\mathrm{O},\mathrm{aerosol}}{⟶}\mathrm{2}{\mathrm{NHO}}_{\mathrm{3}}.\end{array}$$

In this framework, Δ¹⁷O(NO₂) is driven by the relative importance of the different NO₂ production channels, because NO₂ loss processes do not fractionate in terms of oxygen mass-independent anomaly. Each NO₂ production channel generates a specific mass-independent isotopic anomaly Δ¹⁷O on the produced NO₂ (Kaiser et al., 2004). Based on the NO₂ continuity equation, this can be expressed with the following Δ¹⁷O(NO₂) mass-balance equation (Morin et al., 2011):

$$\begin{array}{}\text{(2)}& \begin{array}{rl}{\displaystyle \frac{\mathrm{d}}{\mathrm{d}t}}\left(\left[{\mathrm{NO}}_{\mathrm{2}}\right]\times \mathit{\Delta }{}^{\mathrm{17}}\mathrm{O}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right)& =\sum _i\left(P_i\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_i\left({\mathrm{NO}}_{\mathrm{2}}\right)\right)\\ -& \left(\sum _j{L}_j\right)\times \mathit{\Delta }{}^{\mathrm{17}}\mathrm{O}\left({\mathrm{NO}}_{\mathrm{2}}\right),\end{array}\end{array}$$

with [NO₂] being the atmospheric NO₂ mixing ratio, P_i and L_j the NO₂ production/emission and loss rates, respectively (i.e. mixing ratio of involved species multiplied by the kinetic constant of the considered chemical reaction), and Δ¹⁷O_i(NO₂) the specific isotope anomaly transferred to NO₂ through the production reaction i.

4.1.2 Δ¹⁷O_day(NO₂)

By day, the NO_x photochemical cycle (Reactions R1 to R4) achieves a steady state in 1–2 min, which is several orders of magnitude faster than NO₂ loss reactions (Atkinson et al., 1997) and emission rate (NO_x is mainly emitted under the form of NO; Villena et al., 2011). It follows that NO and NO₂ short variations can be neglected; i.e. $\frac{\mathrm{d}}{\mathrm{d}t}\left[{\mathrm{NO}}_{\mathrm{2}}\right]\approx \mathrm{0}$ and $\frac{\mathrm{d}}{\mathrm{d}t}\left[\mathrm{NO}\right]\approx \mathrm{0}$ on short timescales. In addition, fast interconversions between NO and NO₂ generate quickly an isotopic equilibrium between NO and NO₂ resulting in Δ¹⁷O(NO₂) ≈ Δ¹⁷O(NO) (Michalski et al., 2014; Morin et al., 2007). With these approximations, considering only the main reactions and neglecting halogen chemistry, Eq. (2) yields the following (Morin et al., 2007):

$$\begin{array}{}\text{(3)}& \begin{array}{rl}& \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)\approx \\ & {\displaystyle \frac{\begin{array}{c}{k}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)\\ +k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)\end{array}}{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]}},\end{array}\end{array}$$

with $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ being the ozone isotopic anomaly transferred to NO during its oxidation to NO₂ via Reaction (R3) (also called the transfer function of the isotope anomaly of ozone to NO₂; Savarino et al., 2008) and $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ being the RO₂ isotopic anomaly transferred to NO during its oxidation to NO₂ via Reaction (R4). $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ can be considered to be negligible (Alexander et al., 2020; Michalski et al., 2003), because RO₂ is mainly formed by the reactions R + O₂ and H + O₂ and because the isotopic anomaly of atmospheric O₂ is very close to 0 ‰ (Barkan and Luz, 2003). This assumption has been estimated to affect the overall Δ¹⁷O of RO₂ values by less than 1 ‰ (Röckmann et al., 2001). As a result, Eq. (3) can be simplified, giving a $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ driven by the relative importance of Reaction (R3) (NO + O₃) and Reaction (R4) (NO + RO₂) in the NO oxidation and by the oxygen isotopic anomaly transferred from O₃ to NO₂:

$$\begin{array}{}\text{(4)}& \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)\approx T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right),\end{array}$$

with

$$\begin{array}{}\text{(5)}& T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]}}.\end{array}$$

$\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ has been determined experimentally by Savarino et al. (2008). They reported $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)=\mathrm{1.18}\pm \mathrm{0.07}\times \mathit{\Delta }{}^{\mathrm{17}}\mathrm{O}\left({\mathrm{O}}_{\mathrm{3}}\right)+(\mathrm{6.6}\pm \mathrm{1.5})$ ‰ with Δ¹⁷O(O₃) being the bulk ozone isotopic anomaly. Δ¹⁷O(O₃) has been measured in Grenoble in 2012 (Vicars and Savarino, 2014) with a mean value of (26.2 ± 1.3) ‰, corresponding to a $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ value of (37.5 ± 2.8) ‰, which, according to Eq. (4), would give a maximum $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ value of (37.5 ± 2.8) ‰. It is consistent with our maximum measured Δ¹⁷O(NO₂) value of 39.2 ‰ for the 09:00–12:00 LT interval. In light of the known uncertainties, the small difference is not significant and is much smaller than the diurnal variations of Δ¹⁷O(NO₂). Note that the Δ¹⁷O calibration is not very accurate for the most enriched samples, because nitrite standards with high Δ¹⁷O are still not readily available. In a laboratory study, Michalski et al. (2014) measured the Δ¹⁷O of NO₂ formed by the photochemical NO–NO₂–O₃ cycle and reported Δ¹⁷O(NO₂) = (39.3 ± 1.9) ‰. Despite experimental conditions that are not strictly applicable to our atmospheric conditions (e.g. NO_x ≫ O₃, light source, absence of volatile organic compounds (VOCs)), their value is surprisingly close to our maximum value. Assuming that our maximum Δ¹⁷O(NO₂) value corresponds to $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ close to unity (Reaction R3 (NO + O₃) ≫ Reaction R4 (NO + RO₂)), we use a $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ value of 39.2 ‰ for the following calculations. Combining Eqs. (4) and (5), an expression for the RO₂ mixing ratio can be derived as

$$\begin{array}{}\text{(6)}& \left[{\mathrm{RO}}_{\mathrm{2}}\right]={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}}}\left({\displaystyle \frac{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}}-\mathrm{1}\right).\end{array}$$

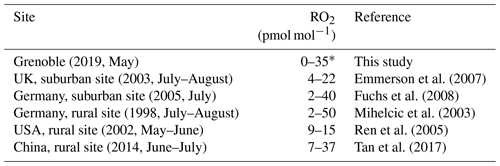
Figure 4 shows the daytime evolution of $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ calculated from Eq. (4) and RO₂ calculated from Eq. (6). $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ varies between 0.76 and 1 with a mean daytime value of 0.86 (the measured daytime Δ¹⁷O(NO₂) mean value is (33.5 ± 3.9) ‰), meaning that 86 % of NO₂ is formed via Reaction (R3) (oxidation of NO by O₃). The mean estimated RO₂ mixing ratio is (13.8 ± 11.2) pmol mol⁻¹. Note that RO₂ = 0 pmol mol⁻¹ for the 09:00–12:00 LT interval originates from our assumption of $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ = 1 for our highest Δ¹⁷O(NO₂) value; in reality, it only means that RO₂ is so low that Reaction (R3) (NO + O₃) ≫ Reaction (R4) (NO + RO₂). Overall, our RO₂ values are found to be within the range of values measured at urban/peri-urban sites (see Table 2). However, RO₂ diurnal variation at our site does not follow the pattern of previous measurements which usually report a diurnal variation with a maximum varying from noon to early afternoon (Fuchs et al., 2008; Tan et al., 2017), whereas this study shows a maximal mixing ratio in late afternoon. With such a limited dataset (only 1 d of sampling), it is not possible to draw general conclusions on the NO_x $/$ RO₂ chemistry dynamics. An important recommendation for further investigation is to conduct isotopic measurements with accurate measurements of key atmospheric radicals/oxidants, e.g. NO, O₃, and possibly RO₂, in order to test quantitatively our isotopic approach. Additionally, the use of a chemical box model is also recommended, because it would allow us to account for non-equilibrium effects in isotopic transfers and thus strengthen the interpretation of isotopic measurements in the investigation of the reactive nitrogen cycle in urban atmospheres.

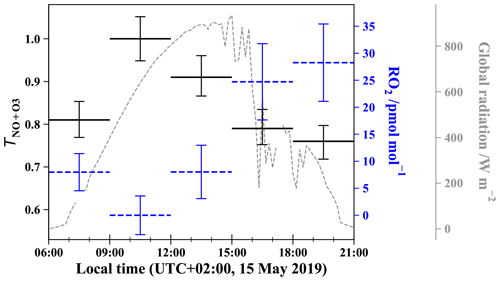
Figure 4 Daytime evolution of $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ (solid black lines), estimated from Eq. (4) using measured Δ¹⁷O(NO₂) in Grenoble, and of RO₂ mixing ratios (dashed blue lines), estimated from Eq. (6). Error bars for $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ are derived from standard deviations of Δ¹⁷O(NO₂) and Δ¹⁷O(O${}_{\mathrm{3}}^{\ast }$) measured in Grenoble (Vicars and Savarino, 2014). RO₂ error bars are derived from O₃ measurement uncertainties and errors on $T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ (by comparison, errors on reaction constants can be neglected). Global solar radiation flux is represented by dashed lines (measured at 200 m from the sampling site by the IGE weather station with a Skye SP1110 pyranometer).

Table 2 Mean daytime RO₂ mixing ratio ranges measured during field campaigns in various environments and seasons.

^∗ Derived from Eq. (6) using Δ¹⁷O values of atmospheric NO₂ in Grenoble.

Morin et al. (2011) simulated the diurnal variation of Δ¹⁷O(NO₂) in a remote marine boundary layer without the effect of NO_x emissions. They assumed Δ¹⁷O(O₃) = 30 ‰ ($\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$ = 45 ‰) resulting in higher overall Δ¹⁷O(NO₂) values compared to our study. Their simulated Δ¹⁷O(NO₂) exhibited large diurnal variations with maximum values at night (close to 41 ‰) and minimum values at noon of 28 ‰. This is consistent with RO₂ mixing ratio reaching a maximum around local noon in clean environments. In contrast to their model simulations, our daytime Δ¹⁷O(NO₂) measurements are higher than our nighttime measurements. We will show in the following section that this difference originates from the absence of NO_x emissions in the Morin et al. (2011) photochemical modelling.

4.1.3 Δ¹⁷O_night(NO₂)

Without photolysis at night and associated RO₂ production, ozone is the unique NO oxidant. NO and NO₂ are no longer in photochemical equilibrium, because NO₂ cannot be converted back into NO. As a result, the oxygen isotopic composition of NO₂ formed during the night is determined by the oxygen isotopic composition of O₃ and emitted NO. Additionally, in order to estimate the overall isotopic signature of sampled NO₂ at night, we need to determine the residuals of NO₂ formed during the day that are still present during the night:

$$\begin{array}{}\text{(7)}& \begin{array}{rl}\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}\right)& \approx x\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)+{\displaystyle \frac{(\mathrm{1}-x)}{\mathrm{2}}}\\ & \times \left(\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)+\mathit{\Delta }{}^{\mathrm{17}}\mathrm{O}\left(\mathrm{NO}\right)\right),\end{array}\end{array}$$

with x being the fraction of NO₂ formed during the day to the total NO₂ measured at night and with (1−x) representing the fraction of NO₂ which has been produced during the night to the total NO₂ measured at night. NO is mainly emitted by combustion processes in which a nitrogen atom (from atmospheric N₂ or N present in fuel) is added to an oxygen atom formed by the thermal decomposition of O₂ (Zeldovich, 1946). With Δ¹⁷O(O₂) being close to 0 ‰ (Barkan and Luz, 2003), NO emissions are very likely to have a Δ¹⁷O ≈ 0 ‰ or at least be negligible compared to $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$. Using Eq. (7) and assuming a negligible isotope anomaly for NO, the time evolution of Δ¹⁷O(NO₂) over the night can be calculated. It is worth pointing out that the x fraction becomes very small at the end of the night, allowing us to further simplify Eq. (7): $\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}\right)=\frac{\mathrm{1}}{\mathrm{2}}\times \mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)$. Thus, if there are nighttime NO emissions, a measurement of Δ¹⁷O(NO₂) at the end of the night is also an interesting way of deriving Δ¹⁷O(O₃) which is difficult to measure directly. The nighttime variation of the x fraction is estimated considering that the nighttime lifetime of NO₂ relative to oxidation via ozone and dry deposition is 7.2 h (O₃ chemical sink is dominant over deposition by a factor > 10⁴ with $k_{{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{3}}}=\mathrm{1.4}\times {\mathrm{10}}^{-\mathrm{13}}\exp [-\mathrm{2470}(K)/T]$ cm³ mol⁻¹ s⁻¹; Atkinson et al., 2004; NO₂ dry velocity V_d = 0.25 cm s⁻¹; Holland et al., 1999; and assuming a nighttime boundary layer height of 500 m). For the 00:00–05:00 LT interval, we calculate a mean value of Δ¹⁷O(NO₂) = 19.9 ‰ (with an overall error of about 1.6 ‰), which is very close to our measured Δ¹⁷O(NO₂) of 20.5 ‰. This first dataset of nighttime Δ¹⁷O(NO₂) measurements appears to confirm our understanding of nocturnal NO₂ formation (Alexander et al., 2020; Michalski et al., 2014). NO emissions in urban areas have a very significant influence on Δ¹⁷O(NO₂), leading to a behaviour in opposition to the one observed in remote locations. As illustrated by Morin et al. (2011), Δ¹⁷O(NO₂) is predicted to be maximal at night in remote areas where NO emissions are negligible, reflecting the isotopic signature of NO₂ at sunset. In areas where nighttime NO emissions are high, nighttime Δ¹⁷O(NO₂) can be up to a factor of 2 smaller than in remote areas.

4.2 Nitrogen isotope composition

Measured δ¹⁵N(NO₂) values range from −11.8 ‰ to −4.9 ‰ with no clear diurnal variation and values clustering around an overall mean of (−10.2 ± 2.2) ‰ (see Fig. 3). Using a similar method, Walters et al. (2018) collected atmospheric NO₂ over 1 month in a urban/suburban location during the summer. They reported a mean δ¹⁵N value of (−11.4 ± 6.9) ‰, very close to our mean value but with a wider overall range (from −31.4 ‰ to +0.4 ‰). In another urban area but using passive samplers, Dahal and Hastings (2016) reported mean δ¹⁵N(NO₂) values of (−8.3 ± 0.9) ‰ and (−6.4 ± 1.4) ‰ for summer and winter periods, respectively. All these values are within the δ¹⁵N range for NO emitted by industrial combustion and traffic sources which are reported to vary from −19.7 ‰ to −13.7 ‰ and from −9 ‰ to −2 ‰, respectively (Miller et al., 2017; Walters et al., 2015). Interestingly, all the δ¹⁵N values measured at our sampling site fall within a narrow range, from about −12 ‰ to −10 ‰, except for the sample collected between 06:00 and 09:00 LT which has a much higher value of −4.9 ‰. This singular value is well correlated with the morning NO traffic emission spike (see Fig. 2). However, once emitted into the atmosphere, NO can undergo isotopic fractionations that modify the nitrogen isotope distribution in NO₂ relative to emitted NO (Freyer et al., 1993). In order to use δ¹⁵N(NO₂) as a tracer of NO_x sources, we need to quantify these nitrogen isotopic shifts to correct measured δ¹⁵N(NO₂). Nitrogen isotopic fractionation, defined as Δ(NO₂−NO_x) = δ¹⁵N(NO₂)−δ¹⁵N(NO_x), is the result of a combination of three effects: (1) an equilibrium isotope effect (EIE) between NO and NO₂, (2) a kinetic isotope effect (KIE) during NO oxidation to NO₂, and (3) a photochemical isotope fractionation effect (PHIFE) during NO₂ photolysis (other NO₂ sinks are negligible during the day). The overall daytime nitrogen isotopic shift of NO₂ relative to emitted NO_x (Δ_day(NO₂−NO_x)) can be estimated using the steady-state isotopic mass balance for NO₂. Li et al. (2020) derived an expression for Δ(NO₂−NO_x) by assuming that the conversion of NO to NO₂ is solely driven by O₃. This could therefore lead to uncertainties on the NO₂ shift when other conversion pathways become significant with respect to the NO conversion by O₃. A more general expression for Δ(NO₂−NO_x) can be derived by taking into account the conversion of NO to NO₂ via other species, notably RO₂ (see Eq. C11 in Appendix C). In our urban environment, we only consider the conversion of NO into NO₂ via O₃ and RO₂ during the day. Assuming ${\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)}\approx {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}\right)}$ (see derivation in Appendix C), Δ_day(NO₂−NO_x) can be expressed by

$$\begin{array}{}\text{(8)}& \begin{array}{rl}{\mathit{\Delta }}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)& ={\displaystyle \frac{{\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }{A}_{\mathrm{day}}^{\ast }+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{day}}^{\ast }+\mathrm{1}}}\\ & \times \left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right),\end{array}\end{array}$$

with

$${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }={\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)}-{\mathit{\alpha }}_{\mathrm{PHIFE}}$$

and

$$A_{\mathrm{day}}^{\ast }={\displaystyle \frac{J_{{\mathrm{NO}}_{\mathrm{2}}}}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[\mathrm{NO}\right]}}={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{\mathrm{NO}}_{\mathrm{2}}\right]}},$$

where $f_{{\mathrm{NO}}_{\mathrm{2}}}=\left[{\mathrm{NO}}_{\mathrm{2}}\right]/\left[{\mathrm{NO}}_x\right]$, ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$ is the fractionation factor of combined KIE and PHIFE (LCIE represents the Leighton cycle isotope effect), and α_EIE is the EIE fractionation factor. $A_{\mathrm{day}}^{\ast }$ is defined as the ratio of the NO₂ lifetime with respect to isotopic exchanges over the daytime NO₂ chemistry lifetime. $J_{{\mathrm{NO}}_{\mathrm{2}}}$ is the NO₂ photolysis rate, $k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}$ is the rate constant of reaction NO + O₃, $k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}$ is the rate constant of reaction NO + RO₂, and $k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}$ is the rate constant of the isotopic exchange (Reaction CR1) (see Appendix D for rate constants data). Interestingly, we can combine Eqs. (8) and (6) and express $A_{\mathrm{day}}^{\ast }$ as a function of oxygen isotopic variables discussed in the previous section:

$$\begin{array}{}\text{(9)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{\mathrm{NO}}_{\mathrm{2}}\right]}}\left({\displaystyle \frac{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}}\right).\end{array}$$

Since our NO measurements are not precise, and we do not have direct measurements of $J_{{\mathrm{NO}}_{\mathrm{2}}}$ or RO₂, we use Eq. (9) to estimate the NO₂ isotopic fractionation shift. Note that although Li et al. (2020) only consider the NO conversion via O₃ in the analysis of their nitrogen isotopic data, they found an excellent agreement between their calculated values and field isotopic measurements at Jülich, Germany (Freyer et al., 1993). Nonetheless, the reason for this accordance remains unclear, as it could be attributable to an equivalent KIE of NO + O₃ and NO + RO₂ but also to the dominance of the NO oxidation via O₃ over RO₂.

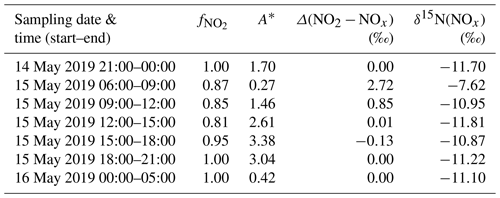
Table 3 Summary of measured $f_{{\mathrm{NO}}_{\mathrm{2}}}$, calculated A^∗ values using Eq. (9) for daytime and Eq. (11) for nighttime, calculated isotopic fractionation between NO₂ and NO_x (Δ(NO₂ − NO_x)) using Eq. (8) for daytime and Eq. (10) for nighttime, and δ¹⁵N(NO_x) estimated with Δ(NO₂ − NO_x) and measured δ¹⁵N(NO₂).

At night, the isotopic fractionation shift Δ_night(NO₂−NO_x) is driven by EIE, KIE, the N isotopic composition of NO emissions and $f_{{\mathrm{NO}}_{\mathrm{2}}}$, given that $J_{{\mathrm{NO}}_{\mathrm{2}}}$ is null (see derivation in Appendix C):

$$\begin{array}{}\text{(10)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)\approx \\ & {\displaystyle \frac{\begin{array}{c}{A}_{\mathrm{night}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-\left(\frac{\mathrm{1}+\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)}{\mathrm{1}+\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)}\right)\right)\\ +\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)\end{array}}{A_{\mathrm{night}}^{\ast }+\mathrm{1}}}\left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right),\end{array}\end{array}$$

where δ¹⁵N(NO_emis) is the N isotopic composition of NO emissions, and

$$\begin{array}{}\text{(11)}& A_{\mathrm{night}}^{\ast }={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{\mathrm{NO}}_{\mathrm{2}}\right]}}={\displaystyle \frac{E\left(\mathrm{NO}\right)}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[\mathrm{NO}\right]\left[{\mathrm{NO}}_{\mathrm{2}}\right]}},\end{array}$$

with E(NO) the NO emission flux. From laboratory experiments, Li et al. (2020) reported fractionation factors of 1.0289 ± 0.0019 and 0.990 ± 0.005 for α_EIE and ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$, respectively. Using these experimental values and the ambient mixing ratios of ozone, NO, and NO₂ measured at our sampling site, we estimate the time evolution of Δ(NO₂−NO_x) from Eqs. (8) and (9) for daytime. At night, [NO] ≪ [NO₂]; hence, $f_{{\mathrm{NO}}_{\mathrm{2}}}$ tends towards 1 and ${\mathit{\Delta }}_{\mathrm{night}}({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x)\approx \mathrm{0}$ (Table 3 provides the calculated values). Δ(NO₂−NO_x) values are found to be negligible during the entire sampling period except between 06:00 and 09:00 LT with a mean Δ(NO₂−NO_x) value of 2.7 ‰ due to lower $f_{{\mathrm{NO}}_{\mathrm{2}}}$ and $A_{\mathrm{day}}^{\ast }$ values. Overall, in our moderately polluted environment, nitrogen oxide isotope effects appear to induce very small nitrogen isotopic shift, reflecting the fact that NO_x is overwhelmingly under the form of NO₂ (mean $f_{{\mathrm{NO}}_{\mathrm{2}}}$ = 0.93). Our results are in good agreement with the Δ(NO₂−NO_x) range (between 1.3 and 2.5 ‰) calculated from isotopic measurements at West Lafayette, USA (Walters et al., 2018). Moreover, Li et al. (2020) calculated a mean Δ(NO₂−NO_x) of (1.3 ± 3.2) ‰ from isotopic measurements near San Diego, USA (NO_x mixing ratios varied from 1 to 9 nmol mol⁻¹).

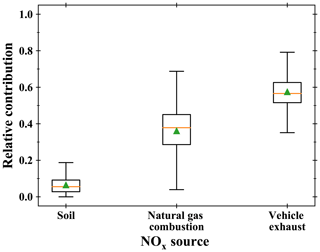
Using estimated δ¹⁵N(NO_x), we evaluate the relative contributions of the dominant NO_x sources at our site using the Bayesian isotopic mixing model SIAR (Stable Isotope Analysis in R; Parnell et al., 2010). Initially developed for ecological studies (Inger et al., 2006; Samelius et al., 2007), isotopic mixing models have been recently used for atmospheric applications, notably to identify major NO_x sources of aerosol nitrate from δ¹⁵N (Jin et al., 2021; Zong et al., 2017; Fan et al., 2019). Using as inputs not only isotopic measurements but also their uncertainties, the SIAR model can be used to calculate potential NO_x source solutions as probability distributions. A recent emission inventory of NO_x in the Grenoble urban area estimated that, in 2016, 52 % of emitted NO_x could be attributed to transport, 26 % to industries, 20 % to the residential/tertiary sectors, and 2 % to agriculture (Topin et al., 2019). Looking at the type of energy consumed by each sector, we estimate that at this time of the year, our sampling site was mostly influenced by fossil-fuel combustion NO_x sources, mainly gasoline/diesel and natural gas, and by biogenic NO_x sources (soils emissions). As shown by previous studies, δ¹⁵N of NO_x emitted by vehicle exhausts depends on the fuel type, the reduction emission technology, and the vehicle run time with values ranging from −21 ‰ to −2 ‰ (Walters et al., 2015). As 90 % of traffic-related NO_x is emitted by diesel-powered engines in the Grenoble urban area (Atmo-Auvergne-Rhônes-Alpes, 2018), we use a value of (−4.7 ± 1.7) ‰, representative of the U.S. vehicle fleet (Miller et al., 2017) for which about 80 % of its traffic-NO_x emissions originate from diesel vehicles (Dallmann et al., 2013). For δ¹⁵N of NO_x emitted by natural gas combustion, we use a value of (−16.5 ± 1.7) ‰ which is the average isotopic signature of natural-gas-burning power plants and residential furnace exhausts (Walters et al., 2015). Despite the large range of δ¹⁵N values for biogenic NO_x (from −59.8 % to −19.9 %) (Li and Wang, 2008; Yu and Elliott, 2017; Walters et al., 2015), these values are still very distinct from δ¹⁵N of fossil-fuel combustion NO_x, making possible to roughly estimate the relative contributions of different NO_x sources at our sampling site. We use a soil–NO_x δ¹⁵N value of (−33.8 ± 12.2) ‰ (Zong et al., 2017), which is the average of values taken from several studies on NO_x emitted by natural and fertilized soil (Felix and Elliott, 2014; Li and Wang, 2008). Over our sampling period, the SIAR model results indicate traffic as the dominant NO_x emission source with a mean relative contribution of (57 ± 8) % (see Fig. 5). Natural gas combustion is found to be the second main NO_x emission source ((36 ± 12) %) before soil emissions which account for only (7 ± 5) %. The limited nature of our measurement dataset (only 1 d of sampling) prevents us from drawing any general and robust conclusions on the relative contributions of NO_x emissions at our site. Nonetheless, we note that the SIAR overall source apportionment is in close agreement with the Grenoble urban area emission inventory concerning traffic emissions (52 % in 2016), lending some support to the idea that δ¹⁵N of NO₂ is a reliable tracer of NO_x emission sources after correction for LCIE and EIE.

Figure 5 Potential NO_x emission source partitioning using the SIAR model based on estimated δ¹⁵N(NO_x). Reference values for each source were taken from Miller et al. (2017), Walters et al. (2015), and Zong et al. (2017).

5 Conclusion

The primary goal of this preliminary work was to address an efficient and portable sampling system for atmospheric NO₂ fitting with accurate isotopic analysis of double nitrogen and triple oxygen isotopes. First simultaneous measurements of the multi-isotopic composition (δ¹⁵N, δ¹⁸O, and Δ¹⁷O) of atmospheric NO₂ are reported here, notably at relatively high temporal resolution (3 h). Over the course of more than 1 d in the Grenoble urban/suburban environment, Δ¹⁷O(NO₂) is found to vary diurnally with a maximum value of (39.2 ± 0.3) ‰ during the day and a minimum value of (20.5 ± 0.3) ‰ at night. At photo-stationary state, high Δ¹⁷O(NO₂) values result from the ozone predominance in NO oxidation pathways, whereas lower values reflect the influence of peroxy radicals. We estimate from our Δ¹⁷O(NO₂) measurements that 86 % of NO₂ produced by day originates from the oxidation of NO by O₃. Moreover, a mean daytime peroxy radical mixing ratio of (13.8 ± 11.2) pmol mol⁻¹ is derived from the oxygen isotopic measurements. At night, NO_x photochemistry shuts down; hence, Δ¹⁷O(NO₂) decreases under the growing influence of the isotopic footprint from NO emitted by night. The Δ¹⁷O(NO₂) measurement towards the end of the night is found to be quantitatively consistent with typical values of Δ¹⁷O(O₃). The δ¹⁵N(NO₂) measurements show little variations, from −11.8 ‰ to −4.9 ‰, with mostly negligible N isotope fractionations between NO and NO₂ due to the high NO₂ $/$ NO_x ratios. After correction of nitrogen isotopic fractionations, we use a Bayesian isotope mixing model to estimate the relative contributions of the dominant NO_x emissions sources. The results indicate the predominance of traffic NO_x emissions in this area at (57 ± 8) %, which is before natural gas combustion and soil emission.

Despite the limited nature of our measurement dataset, our results shed light on the sensitivity of NO₂ isotopic signature to the atmospheric chemical regimes and emissions of the local environment. This isotopic approach can be applied to various environments in order to probe further the oxidative chemistry and help to constrain the NO_x fate in a more quantitative way. In the future, the interpretation of the isotopic data should be extended with the use of a photochemical box model including isotopic anomaly transfers and local emissions in order to solve persistent issues of atmospheric oxidation mechanisms. Moreover, samplings and multi-isotopic analysis of atmospheric nitrate performed in parallel to those of NO₂ would certainly be of interest for the study of the full reactive nitrogen cycle.

Appendix A: Isotopic standards and calibration

This method of analysis induces isotope fractionations during NO${}_{\mathrm{2}}^{-}$ $/$ N₂O conversion and ionization in the spectrometer, as well as isotope exchanges between NO${}_{\mathrm{2}}^{-}$ and its medium. Indeed, while isotope exchanges between nitrite and its matrix are minimized due to the basic pH, the chemistry required to convert nitrite to N₂O involves a step in an acidic medium that promotes an exchange of oxygen isotopes (Casciotti et al., 2007). In order to eliminate the effects of these isotope splits, the system is calibrated using standards of known isotopic composition, which are subjected to the same treatment as the samples. This is called the identical treatment principle (Brand, 1996). By subjecting compounds of known isotopic composition to the same treatment as the samples, the isotope fractionation induced by the analytical protocol can be estimated, and the sample values can be corrected. Standards are first dissolved in a basic aqueous medium (pH = 12), and then, from this stock solution, five series of each standard are prepared in several amount ranges, namely 40, 80, 100, 120, and 150 nmol, in order to estimate the effects of the amount of a material on its isotopic measurement. The matrix used for their preparation is the same as that of the samples, i.e. a mixture of KOH 2 M/guaiacol in deionized water. Correction factors are obtained by linear regression between the raw and the expected values of δ¹⁵N, δ¹⁸O, and δ¹⁷O of the standards. Three international references of known δ¹⁵N and δ¹⁸O values are used for this work. These are nitrite salts, named RSIL-N7373, RSIL-N10219, and RSIL-N23 with respective δ¹⁵N $/$ δ¹⁸O values of −79.6/4.2 ‰, 2.8/88.5 ‰, and 3.7/11.4 ‰. Although the three standards cover a wide range of isotopic composition in δ¹⁵N and δ¹⁸O, they do not have an isotopic anomaly in ¹⁷O. As we are not aware of any available international reference nitrite standards with a known ¹⁷O anomaly, we are currently in the process of manufacturing our own standards. As this step is still under development and in order to be able to assess the accuracy of our ¹⁷O measurements of atmospheric NO₂ samples, we have estimated the isotope fractionation that ¹⁷O undergoes during the analysis. RSIL-N7373 and RSIL-N23 standards have a Δ¹⁷O = 0 ‰, so we estimate their ¹⁷O composition such that δ¹⁷O = 0.52 × δ¹⁸O. For standard RSIL-N10219, we measure a negative Δ¹⁷O around −7 ‰. We therefore apply the mass-independent relation such that δ¹⁷O_std(RSIL-N10219) = Δ¹⁷O_raw(RSIL-N10219) + 0.5 × δ¹⁸O_std(RSIL-N10219).

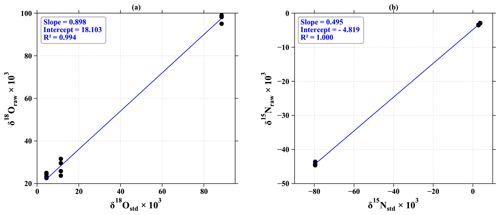
Figure A1 Calibration of (a) ¹⁸O and (b) ¹⁵N with nitrite standards at 100 nmol measured by the chemical azide method. The measured δ¹⁸O (δ¹⁸O_raw) and δ¹⁵N (δ¹⁵N_raw) values of NO${}_{\mathrm{2}}^{-}$ standards are plotted against their certified reference δ¹⁸O (δ¹⁸O_std) and δ¹⁵N (δ¹⁵N_std) values.

The isotopic exchange of ¹⁸O is estimated at 11 % for standards at 100 nmol (Fig. A1), which is in line with Kobayashi et al. (2021), who have estimated the degree of O isotope exchange in the azide method between H₂O and NO${}_{\mathrm{2}}^{-}$ to be (10.8 ± 0.3) %. The ¹⁵N calibration curve allows us to ensure a good fractionation rate during the analysis. Indeed, given the 1:1 association of the nitrogen atoms of nitrite and azide, the theoretical value of the calibration slope must be 0.5. The slight deviation from our measured value can be attributed to a blank effect, estimated here at 2 % of the size of the standards (6 % for those at 40 nmol).

Appendix B: Sample's isotopic stability

Oxygen isotopes in nitrites are very labile (Böhlke et al., 2007), but the basic pH of the eluent limits isotopic exchanges. To ensure isotopic integrity from denuder's extraction to analysis by IRMS, we followed the procedure by Walters et al. (2018) to quantify isotopic exchanges that might occur with the eluted matrix during storage. Thus, three solutions each containing 500 nmol of a KNO₂ salt (RSIL-N7373, RSIL-N10219 and RSIL-N23) were prepared in the eluted matrix and kept frozen. We monitored the nitrite standard isotopic composition prepared in the eluted guaiacol matrix during 22 d. To do so, 100 nmol were collected from the individual solutions, analysed, and refrozen until the next analysis. The temporal evolution of the δ¹⁷O,δ¹⁸O, and Δ¹⁷O differences between our measurements of RSIL standards (prepared in the KOH/guaiacol eluted matrix) and their certified reference values is plotted in Fig. B1. It represents the temporal drift of the isotopic signal with respect to reference values. If the deviation is constant, it means that the isotopic signal is not degraded with time, and its standard deviation is considered the uncertainty in our δ¹⁷O(NO₂) and δ¹⁸O(NO₂) measurements. As shown in Fig. B1, deviation of the three standards was stable over the 22 d experiment with an overall mean of (1.1 ± 0.8) ‰, (2.3 ± 1.8) ‰, and (−0.1 ± 0.3) ‰ for δ¹⁷O, δ¹⁸O, and Δ¹⁷O, respectively. Note that RSIL-N10219 shows higher δ¹⁷O and δ¹⁸O residuals than the two other standards. The reason for this difference in behaviour is still not fully understood. As residuals remain steady over several weeks, we consider this method suitable for the oxygen analysis of NO₂, and the uncertainties applied to our isotopic measurements are reported as the propagation error of the mean measurement uncertainty and the mean uncertainty resulting from NO${}_{\mathrm{2}}^{-}$ storage. In our study, average uncertainties on δ¹⁷O, δ¹⁸O, and Δ¹⁷O are estimated to be ±0.8 ‰, ±1.8 ‰, and ± 0.3 ‰, respectively (1σ uncertainties).

Figure B1 Temporal evolution of δ¹⁷O,δ¹⁸O, and Δ¹⁷O differences between our measurements of RSIL standards (prepared in the KOH/guaiacol eluted matrix) and their certified reference values. Error bars derived from measurement uncertainties are approximately equivalent to the size of the markers.

Appendix C: Deriving the N isotopic fractionation from isotopic exchange and the extended Leighton cycle

We follow the same approach as Li et al. (2020) but take into account all the oxidation pathways of NO into NO₂, not only via O₃. The reactions considered in deriving the combined isotopic fractionation are the following:

$$\begin{array}{}\text{(CR1)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{14}}\mathrm{NO}\to {}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{15}}\mathrm{NO}\\ & k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}},\end{array}\text{(CR2)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{15}}\mathrm{NO}\to {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{}^{\mathrm{14}}\mathrm{NO}\\ & k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\times {\mathit{\alpha }}_{\mathrm{EIE}},\end{array}\text{(CR3)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\to {}^{\mathrm{14}}\mathrm{NO}+\mathrm{O}\\ & J_{{\mathrm{NO}}_{\mathrm{2}}},\end{array}\text{(CR4)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\to {}^{\mathrm{15}}\mathrm{NO}+\mathrm{O}\\ & J_{{\mathrm{NO}}_{\mathrm{2}}}\times {\mathit{\alpha }}_{\mathrm{PHIFE}},\end{array}\text{(CR5)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{14}}\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\to {}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\\ & k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}},\end{array}\text{(CR6)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{15}}\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\to {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\\ & k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\times {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)},\end{array}\text{(CR7)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{14}}\mathrm{NO}+X_i\to {}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\\ & k_{\mathrm{NO}+X_i},\end{array}\text{(CR8)}& {\displaystyle }\begin{array}{rl}& {}^{\mathrm{15}}\mathrm{NO}+X_i\to {}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{2}}\\ & k_{\mathrm{NO}+X_i}\times {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+X_i\right)},\end{array}\end{array}$$

with X_i = RO₂, BrO, ClO, …, .

C1 Daytime N fractionation

During the day, NO₂ photolysis is the overwhelmingly dominant NO₂ sink, and NO oxidation is the main NO₂ source (see Fig. C1). The assumption of steady state on ¹⁵NO₂ for the extended Leighton cycle leads to

$$\begin{array}{}\text{(C1)}& \begin{array}{rl}& k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{14}}\mathrm{NO}\right]+J_{{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{PHIFE}}\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]=\\ & \sum \left(k_{\mathrm{NO}+X_i}{\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+X_i\right)}\left[{}^{\mathrm{15}}\mathrm{NO}\right]\left[X_i\right]\right)\\ & +k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{15}}\mathrm{NO}\right],\end{array}\end{array}$$

where $k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}$ is the rate constant for the nitrogen isotopic exchange between NO and NO₂, $J_{{\mathrm{NO}}_{\mathrm{2}}}$ is the NO₂ photolysis rate with α_PHIFE being its isotopic fractionation factor, $\sum k_{\mathrm{NO}+X_i}\left[X_i\right]$ the sum of all the NO oxidation pathways to NO₂, X_i the NO oxidant (i.e. O₃, RO₂, BrO, ClO …), and $k_{\mathrm{NO}+X_i}$ the rate constant for the reaction of NO + X_i with ${\mathit{\alpha }}_{\mathrm{KIE}(\mathrm{NO}+X_i)}$ being its isotopic fractionation factor. Equation (C1) can be rearranged to give

$$\begin{array}{}\text{(C2)}& {\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]}}={\displaystyle \frac{\begin{array}{c}\sum \left(k_{\mathrm{NO}+X_i}{\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+X_i\right)}\left[X_i\right]\right)\\ +k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\end{array}}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]+J_{{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{PHIFE}}}}.\end{array}$$

Meanwhile, ¹⁴NO₂ in steady state leads to

$$\begin{array}{}\text{(C3)}& {\displaystyle \frac{\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}{\left[{}^{\mathrm{14}}\mathrm{NO}\right]}}={\displaystyle \frac{\sum k_{\mathrm{NO}+X_i}\left[X_i\right]}{J_{{\mathrm{NO}}_{\mathrm{2}}}}}.\end{array}$$

We define $A_{\mathrm{day}}^{\ast }$ as the ratio of the ¹⁴NO₂ lifetime with respect to isotopic exchange with ¹⁴NO (${\mathit{\tau }}_{\mathrm{exchange}-{\mathrm{NO}}_{\mathrm{2}}}$) over the daytime ¹⁴NO₂ chemical lifetime (${\mathit{\tau }}_{\mathrm{chem}-{\mathrm{NO}}_{\mathrm{2}}}$) (Li et al., 2020):

$$\begin{array}{}\text{(C4)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{{\mathit{\tau }}_{\mathrm{exchange}-{\mathrm{NO}}_{\mathrm{2}}}}{{\mathit{\tau }}_{\mathrm{chem}-{\mathrm{NO}}_{\mathrm{2}}}}}={\displaystyle \frac{J_{{\mathrm{NO}}_{\mathrm{2}}}}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]}}.\end{array}$$

Using Eq. (C3), Eq. (C4) becomes

$$\begin{array}{}\text{(C5)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{\sum k_{\mathrm{NO}+X_i}\left[X_i\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

We also define $T_{\mathrm{NO}+X_i}$ as the relative importance of the oxidation pathway of NO into NO₂ via the oxidant X_i:

$$\begin{array}{}\text{(C6)}& T_{\mathrm{NO}+X_i}={\displaystyle \frac{k_{\mathrm{NO}+X_i}\left[X_i\right]}{\sum k_{\mathrm{NO}+X_i}\left[X_i\right]}},\end{array}$$

with necessarily $\sum T_{\mathrm{NO}+X_i}=\mathrm{1}$.

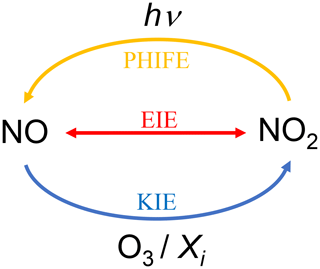
Figure C1 Sketch of the nitrogen fractionation processes between NO and NO₂. PHIFE represents the photochemical isotope fractionation effect, KIE represents the kinetic isotope effect, and EIE represents the equilibrium isotope effect.

Using the definitions (C5) and (C6), Eq. (C2) becomes

$$\begin{array}{}\text{(C7)}& {\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]}}={\displaystyle \frac{\begin{array}{c}{A}_{\mathrm{day}}^{\ast }{k}_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]{\mathit{\alpha }}_{\mathrm{KIE}}\\ +k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\end{array}}{\begin{array}{c}{k}_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]+\\ A_{\mathrm{day}}^{\ast }{k}_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]{\mathit{\alpha }}_{\mathrm{PHIFE}}\end{array}}},\end{array}$$

with ${\mathit{\alpha }}_{\mathrm{KIE}}=\sum T_{\mathrm{NO}+X_i}+{\mathit{\alpha }}_{\mathrm{KIE}(\mathrm{NO}+X_i)}$.

Using $R\left({}^{\mathrm{15}}\mathrm{N}{/}^{\mathrm{14}}\mathrm{N},\mathrm{NO}\right)=R_{\mathrm{NO}}\left({}^{\mathrm{15}}\mathrm{N}\right)/R_{\mathrm{NO}}\left({}^{\mathrm{14}}\mathrm{N}\right)={}^{\mathrm{15}}{R}_{\mathrm{NO}}$ (with $\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left(\mathrm{NO}\right){=}^{\mathrm{15}}{R}_{\mathrm{NO}}{/}^{\mathrm{15}}{R}_{\mathrm{standard}}-\mathrm{1}$) and $R{(}^{\mathrm{15}}\mathrm{N}{/}^{\mathrm{14}}\mathrm{N},{\mathrm{NO}}_{\mathrm{2}})=R_{{\mathrm{NO}}_{\mathrm{2}}}({}^{\mathrm{15}}\mathrm{N})/R_{{\mathrm{NO}}_{\mathrm{2}}}({}^{\mathrm{14}}\mathrm{N})={}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}$ (with $\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right){=}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}/{}^{\mathrm{15}}{R}_{\mathrm{standard}}-\mathrm{1}$), Eq. (C7) becomes

$$\begin{array}{}\text{(C8)}& {\displaystyle \frac{{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}}{{}^{\mathrm{15}}{R}_{\mathrm{NO}}}}={\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{14}}\mathrm{NO}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}={\displaystyle \frac{A_{\mathrm{day}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}{\mathrm{1}+A^{\ast }{\mathit{\alpha }}_{\mathrm{PHIFE}}}},\text{(C9)}& {\displaystyle \frac{{}^{\mathrm{15}}{R}_{\mathrm{NO}}}{{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}}}-\mathrm{1}={\displaystyle \frac{A_{\mathrm{day}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{PHIFE}}-{\mathit{\alpha }}_{\mathrm{KIE}}\right)-\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{day}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}.\end{array}$$

As a result, the daytime isotopic shift of NO₂ relative to NO, defined as Δ(NO₂−NO) = δ¹⁵N(NO₂)−δ¹⁵N(NO), is given by

$$\begin{array}{}\text{(C10)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}-\mathrm{NO}\right)=\\ & {\displaystyle \frac{A_{\mathrm{day}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-{\mathit{\alpha }}_{\mathrm{PHIFE}}\right)+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{day}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}\\ & \times \left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right).\end{array}\end{array}$$

Using the isotopic balance $\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_x\right)=f_{{\mathrm{NO}}_{\mathrm{2}}}\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)+(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}})\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left(\mathrm{NO}\right)$ with $f_{{\mathrm{NO}}_{\mathrm{2}}}$ = [NO₂] $/$ [NO_x] (Li et al., 2020), the isotopic shift of NO₂ relative to NO_x, defined as $\mathit{\Delta }({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x)=\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)-\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_x\right)$, can be expressed by

$$\begin{array}{}\text{(C11)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)=\\ & {\displaystyle \frac{A_{\mathrm{day}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-{\mathit{\alpha }}_{\mathrm{PHIFE}}\right)+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{day}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}\\ & \times \left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right)\left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right).\end{array}\end{array}$$

Since fractionation factors are close to unity and $\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\approx \mathrm{1}$, Eq. (C11) can be further simplified by keeping only the dominant terms (Li et al., 2020):

$$\begin{array}{}\text{(C12)}& \begin{array}{rl}{\mathit{\Delta }}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)& \approx {\displaystyle \frac{{\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }{A}_{\mathrm{day}}^{\ast }+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A^{\ast }+\mathrm{1}}}\\ & \times \left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right),\end{array}\end{array}$$

with ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }={\mathit{\alpha }}_{\mathrm{KIE}}-{\mathit{\alpha }}_{\mathrm{PHIFE}}$.

Considering the localization of our sampling site (urban mid-latitude area), only NO + RO₂ and NO + O₃ are thought to be significant as NO₂ formation pathways; hence, ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$ becomes

$$\begin{array}{}\text{(C13)}& \begin{array}{rl}{\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }& =T_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\times {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)}\\ & +T_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\times {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}\right)}-{\mathit{\alpha }}_{\mathrm{PHIFE}},\end{array}\end{array}$$

and Eq. (C5) becomes

$$\begin{array}{}\text{(C14)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

Equations (4) and (5) from Sect. 4.1.2 can be combined to give

$$\begin{array}{}\text{(C15)}& \begin{array}{rl}& k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]=\\ & {\displaystyle \frac{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}}{k}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right].\end{array}\end{array}$$

Using Eq. (C15), Eq. (C14) becomes

$$\begin{array}{}\text{(C16)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}\left({\displaystyle \frac{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}{\mathit{\Delta }{{}^{\mathrm{17}}\mathrm{O}}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}\right)}}\right).\end{array}$$

We consider several particular cases. The first case is when ${\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}\right)}\approx {\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)}$. Previous studies found that the NO + O₃ reaction falls within the family of “normal kinetic isotope fractionation” with the NO₂ produced being depleted in ¹⁵N (Walters and Michalski, 2016) compared to residual reactant NO. To our knowledge, no such experiment has been carried out for the NO + RO₂ reaction. Nonetheless, considering the very close, and both very low, activation energies for the reaction NO + O₃ and NO + RO₂, it is quite likely that the fractionation factors of these two reactions are similar. It follows that we obtain the same expression for ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$ as for the α_LCIE given in Li et al. (2020):

$$\begin{array}{}\text{(C17)}& {\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }={\mathit{\alpha }}_{\mathrm{KIE}\left(\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}\right)}-{\mathit{\alpha }}_{\mathrm{PHIFE}}.\end{array}$$

And Eq. (C12) becomes

$$\begin{array}{}\text{(C18)}& \begin{array}{rl}{\mathit{\Delta }}_{\mathrm{day}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)& ={\displaystyle \frac{{\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }{A}_{\mathrm{day}}^{\ast }+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{day}}^{\ast }+\mathrm{1}}}\\ & \times \left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right).\end{array}\end{array}$$

Equation (C18) with $A_{\mathrm{day}}^{\ast }$ given by Eq. (C16) is the expression that we use to analyse our daytime nitrogen isotopic measurements. Another particular case considered by Li et al. (2020) is $k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]\gg k_{\mathrm{NO}+{\mathrm{RO}}_{\mathrm{2}}}\left[{\mathrm{RO}}_{\mathrm{2}}\right]$; in that case, ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$ is still given by Eq. (C12) but A^∗ is simplified:

$$\begin{array}{}\text{(C19)}& A_{\mathrm{day}}^{\ast }={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

Equation (C16) with $A_{\mathrm{day}}^{\ast }$ given by Eq. (C19) and ${\mathit{\alpha }}_{\mathrm{LCIE}}^{\ast }$ given by Eq. (C17) is the same expression as Eq. (8) in Li et al. (2020).

C2 Nighttime N fractionation

An expression similar to Eq. (C1) can be derived for nighttime conditions, when NO₂ photolysis is null and hence when there is no recycling between NO and NO₂. In addition, the conversion of NO into NO₂ occurs only via reaction with O₃ because the mixing ratios of other NO oxidants are usually negligible at night. The main source of NO_x at night is the NO emissions. The assumption of steady state on short timescales can only hold for ¹⁴NO and ¹⁵NO, not NO₂, leading to an equation equivalent to Eq. (C1):

$$\begin{array}{}\text{(C20)}& \begin{array}{rl}& k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{14}}\mathrm{NO}\right]+E\left({}^{\mathrm{15}}\mathrm{NO}\right)=\\ & k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}{\mathit{\alpha }}_{\mathrm{KIE}}\left[{}^{\mathrm{15}}\mathrm{NO}\right]\left[{\mathrm{O}}_{\mathrm{3}}\right]\\ & +k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{15}}\mathrm{NO}\right],\end{array}\end{array}$$

with E(¹⁵NO) being the ¹⁵NO emission flux and α_KIE is the fractionation factor of NO + O₃. Equation (C20) can be rearranged to give

$$\begin{array}{}\text{(C21)}& {\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]}}={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}{\mathit{\alpha }}_{\mathrm{KIE}}\left[{\mathrm{O}}_{\mathrm{3}}\right]+k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]+E\left({}^{\mathrm{15}}\mathrm{NO}\right)/\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

Meanwhile, the steady state on ¹⁴NO gives (nitrogen isotopic exchanges are neglected as NO emissions are largely dominated by ¹⁴NO)

$$\begin{array}{}\text{(C22)}& E\left({}^{\mathrm{14}}\mathrm{NO}\right)=k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]\left[{\mathrm{O}}_{\mathrm{3}}\right],\end{array}$$

with E(¹⁴NO) being the ¹⁴NO emission flux. For nighttime, we define $A_{\mathrm{night}}^{\ast }$ as the ratio of the ¹⁴NO lifetime with respect to isotopic exchange with ¹⁴NO₂ (τ_{exchange−NO}) over the nighttime ¹⁴NO chemical lifetime (τ_chem−NO):

$$\begin{array}{}\text{(C23)}& A_{\mathrm{night}}^{\ast }={\displaystyle \frac{{\mathit{\tau }}_{\mathrm{exchange}-\mathrm{NO}}}{{\mathit{\tau }}_{\mathrm{chem}-\mathrm{NO}}}}={\displaystyle \frac{k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

Using Eq. (C22), Eq. (C23) gives

$$\begin{array}{}\text{(C24)}& A_{\mathrm{night}}^{\ast }={\displaystyle \frac{E\left({}^{\mathrm{14}}\mathrm{NO}\right)}{k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}.\end{array}$$

NO_x is overwhelmingly emitted in the form of NO. The isotopic signature of NO emissions can be characterized with ${}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}$ and $\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)={}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}/{}^{\mathrm{15}}{R}_{\mathrm{standard}}-\mathrm{1}$. Using the definition of ${}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}$, Eqs. (C23), and (C24), Eq. (C21) becomes

$$\begin{array}{}\text{(C25)}& {\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]}}={\displaystyle \frac{\begin{array}{c}{A}_{\mathrm{night}}^{\ast }{k}_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]{\mathit{\alpha }}_{\mathrm{KIE}}\\ +k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}{\mathit{\alpha }}_{\mathrm{EIE}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\end{array}}{\begin{array}{c}{k}_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]+\\ \left({}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}E\left({}^{\mathrm{14}}\mathrm{NO}\right)\right)/\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\end{array}}}.\end{array}$$

And then, using Eq. (C24), Eq. (C25) becomes

$$\begin{array}{}\text{(C26)}& {\displaystyle }\begin{array}{rl}{\displaystyle \frac{{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}}{{}^{\mathrm{15}}{R}_{\mathrm{NO}}}}& ={\displaystyle \frac{\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]\left[{}^{\mathrm{14}}\mathrm{NO}\right]}{\left[{}^{\mathrm{15}}\mathrm{NO}\right]\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]}}\\ & ={\displaystyle \frac{A_{\mathrm{night}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}{\mathrm{1}+A_{\mathrm{night}}^{\ast }\left({}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}/{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}\right)}}\end{array},\text{(C27)}& {\displaystyle \frac{{}^{\mathrm{15}}{R}_{\mathrm{NO}}}{{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}}}-\mathrm{1}={\displaystyle \frac{\begin{array}{c}{A}_{\mathrm{night}}^{\ast }\left(\left({}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}/{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}\right)-{\mathit{\alpha }}_{\mathrm{KIE}}\right)\\ -\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)\end{array}}{A_{\mathrm{night}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}.\end{array}$$

Following the approach used in the derivation of daytime isotopic shift, the nighttime isotopic shift of NO₂ relative to NO is given by

$$\begin{array}{}\text{(C28)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-\mathrm{NO}\right)=\\ & {\displaystyle \frac{A_{\mathrm{night}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-\left({}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}/{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}\right)\right)+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{night}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}\\ & \times \left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right).\end{array}\end{array}$$

Using the isotopic balance $\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_x\right)=f_{{\mathrm{NO}}_{\mathrm{2}}}\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)+(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}})\mathit{\delta }{}^{\mathrm{15}}\mathrm{N}\left(\mathrm{NO}\right)$, the nighttime isotopic shift of NO₂ relative to NO_x, can be expressed by

$$\begin{array}{}\text{(C29)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)=\\ & {\displaystyle \frac{A_{\mathrm{night}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-\left(\frac{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)}{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)}\right)\right)+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{night}}^{\ast }{\mathit{\alpha }}_{\mathrm{KIE}}+{\mathit{\alpha }}_{\mathrm{EIE}}}}\\ & \times \left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right)\left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right),\end{array}\end{array}$$

where δ¹⁵N(NO_emis) is the nitrogen isotopic composition of NO emissions.

Keeping the dominant terms, Eq. (C29) can be further simplified following the daytime derivation:

$$\begin{array}{}\text{(C30)}& \begin{array}{rl}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)\approx \\ & {\displaystyle \frac{A_{\mathrm{night}}^{\ast }\left({\mathit{\alpha }}_{\mathrm{KIE}}-\left(\frac{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)}{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)}\right)\right)+\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{A_{\mathrm{night}}^{\ast }+\mathrm{1}}}\\ & \times \left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right).\end{array}\end{array}$$

We consider two particular cases. When $A_{\mathrm{night}}^{\ast }\ll \mathrm{1}$ ($k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\gg k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]$), i.e isotopic exchange much faster than NO oxidation, Eq. (C28) becomes

$$\begin{array}{}\text{(C31)}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-\mathrm{NO}\right)={\displaystyle \frac{\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{{\mathit{\alpha }}_{\mathrm{EIE}}}}\left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right).\end{array}$$

Keeping the dominant terms, Eq. (C31) can be simplified:

$$\begin{array}{}\text{(C32)}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-\mathrm{NO}\right)\approx \left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right).\end{array}$$

As expected, the nighttime isotopic shift of NO₂ relative to NO depends only on the isotopic exchange fractionation in that case. In the same way, Eq. (C29) becomes

$$\begin{array}{}\text{(C33)}& \begin{array}{rl}{\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)& ={\displaystyle \frac{\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{{\mathit{\alpha }}_{\mathrm{EIE}}}}\\ & \times \left(\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\right)\left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right).\end{array}\end{array}$$

Keeping the dominant terms, Eq. (C33) can be simplified:

$$\begin{array}{}\text{(C34)}& {\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-{\mathrm{NO}}_x\right)\approx {\displaystyle \frac{\left({\mathit{\alpha }}_{\mathrm{EIE}}-\mathrm{1}\right)}{{\mathit{\alpha }}_{\mathrm{EIE}}}}\left(\mathrm{1}-f_{{\mathrm{NO}}_{\mathrm{2}}}\right).\end{array}$$

When $A_{\mathrm{night}}^{\ast }\gg \mathrm{1}$ ($k_{\mathrm{NO}+{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]\ll k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]$), i.e NO oxidation much faster than isotopic exchange, Eq. (C28) yields

$$\begin{array}{}\text{(C35)}& \begin{array}{rl}{\mathit{\Delta }}_{\mathrm{night}}\left({\mathrm{NO}}_{\mathrm{2}}-\mathrm{NO}\right)& =\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)\\ & -\left({\displaystyle \frac{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)}{{\mathit{\alpha }}_{\mathrm{KIE}}}}\right),\end{array}\end{array}$$

leading to

$$\begin{array}{}\text{(C36)}& {\displaystyle }\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left(\mathrm{NO}\right)=\left({\displaystyle \frac{\mathrm{1}+{\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right)}{{\mathit{\alpha }}_{\mathrm{KIE}}}}\right)\text{(C37)}& {}^{\mathrm{15}}{R}_{\mathrm{NO}}={\displaystyle \frac{{}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{emis}}}}{{\mathit{\alpha }}_{\mathrm{KIE}}}}.\end{array}$$

As expected, the nighttime isotopic shift of NO relative to NO emissions depends only on the isotopic fractionation factor of the NO + O₃ reaction in that case. It is possible to estimate the nighttime isotopic shift of NO₂ relative to NO or NO_x on long timescales by assuming crudely that ¹⁴NO₂ is in steady state:

$$\begin{array}{}\text{(C38)}& k_{\mathrm{loss}-{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{14}}{\mathrm{NO}}_{\mathrm{2}}\right]=k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}\left[{}^{\mathrm{14}}\mathrm{NO}\right]\left[{\mathrm{O}}_{\mathrm{3}}\right],\end{array}$$

with $k_{\mathrm{loss}-{\mathrm{NO}}_{\mathrm{2}}}$ representing the equivalent of a first-order rate constant. If the ¹⁴NO₂ loss is a second-order reaction such as NO₂ + O₃ loss, then $k_{\mathrm{loss}-{\mathrm{NO}}_{\mathrm{2}}}=k_{{\mathrm{NO}}_{\mathrm{2}}+{\mathrm{O}}_{\mathrm{3}}}\left[{\mathrm{O}}_{\mathrm{3}}\right]$. In the same way, assuming that the NO₂ oxidation into nitrate via O₃ is not fractionating, ¹⁵NO₂ in steady state gives

$$\begin{array}{}\text{(C39)}& k_{\mathrm{loss}-{\mathrm{NO}}_{\mathrm{2}}}\left[{}^{\mathrm{15}}{\mathrm{NO}}_{\mathrm{2}}\right]=k_{\mathrm{NO}+{\mathrm{O}}_{\mathrm{3}}}{\mathit{\alpha }}_{\mathrm{KIE}}\left[{}^{\mathrm{15}}\mathrm{NO}\right]\left[{\mathrm{O}}_{\mathrm{3}}\right].\end{array}$$

Using Eq. (C38), Eq. (C39) becomes

$$\begin{array}{}\text{(C40)}& {}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}={\mathit{\alpha }}_{\mathrm{KIE}}{}^{\mathrm{15}}{R}_{\mathrm{NO}}.\end{array}$$

Using Eq. (C37), Eq. (C40) becomes

$$\begin{array}{}\text{(C41)}& {}^{\mathrm{15}}{R}_{{\mathrm{NO}}_{\mathrm{2}}}={}^{\mathrm{15}}{R}_{\mathrm{NO},\mathrm{emis}}\end{array}$$

or

$$\begin{array}{}\text{(C42)}& {\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{2}}\right)={\mathit{\delta }}^{\mathrm{15}}\mathrm{N}\left({\mathrm{NO}}_{\mathrm{emis}}\right).\end{array}$$

Under those conditions (negligible isotopic exchange), a measurement of δ¹⁵N(NO₂) is a measurement of δ¹⁵N(NO_emis), preferably towards the end of the night in order for ¹⁴NO₂ to move towards steady state.

In the text, ¹⁴NO₂ and ¹⁴NO are referred to as NO₂ and NO for convenience.

Appendix D: Kinetic data used

Table D1 Rate constants used for calculations.