the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
Influence of aerosol copper on HO2 uptake: a novel parameterized equation
Huan Song
Xiaorui Chen
Qi Zou
Zhaofeng Tan
Hendrik Fuchs
Alfred Wiedensohler
Daniel R. Moon
Dwayne E. Heard
María-Teresa Baeza-Romero
Mei Zheng
Andreas Wahner
Astrid Kiendler-Scharr
Yuanhang Zhang
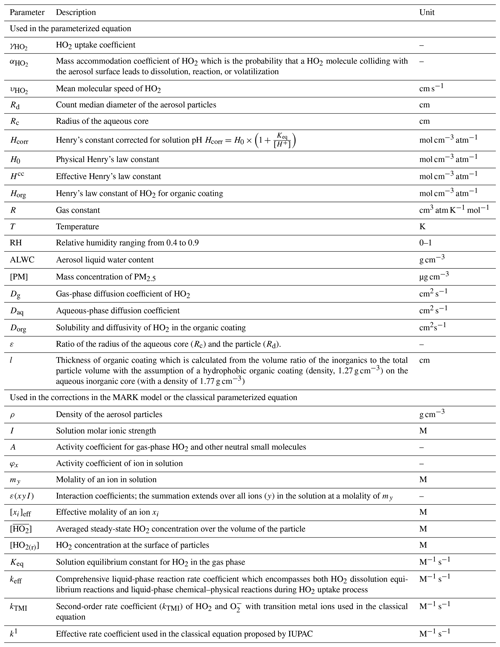
Heterogeneous uptake of hydroperoxyl radicals (HO2) onto aerosols has been proposed to be a significant sink of HOx, hence impacting the atmospheric oxidation capacity. Accurate calculation of the HO2 uptake coefficient is key to quantifying the potential impact of this atmospheric process. Laboratory studies show that can vary by orders of magnitude due to changes in aerosol properties, especially aerosol soluble copper (Cu) concentration and aerosol liquid water content (ALWC). In this study we present a state-of-the-art model called MARK to simulate both gas- and aerosol-phase chemistry for the uptake of HO2 onto Cu-doped aerosols. Moreover, a novel parameterization of HO2 uptake was developed that considers changes in relative humidity (RH) and condensed-phase Cu ion concentrations and which is based on a model optimization using previously published and new laboratory data included in this work. This new parameterization will be applicable to wet aerosols, and it will complement current IUPAC recommendations. The new parameterization is as follows (the explanations for symbols are in the Appendix):
All parameters used in the paper are summarized in Table A1. Using this new equation, field data from a field campaign were used to evaluate the impact of the HO2 uptake onto aerosols on the ROx (= OH + HO2 + RO2) budget. Highly variable values for HO2 uptake were obtained for the North China Plain (median value < 0.1).
- Article
(2011 KB) - Full-text XML
-
Supplement
(844 KB) - BibTeX
- EndNote
The atmospheric cleaning capacity of the troposphere is largely determined by the concentrations of the hydroxyl radical, which are closely linked with the concentrations of the hydroperoxyl (HO2) radical. In the established chemical mechanism, the coupling of OH and HO2 is strongly determined by the reaction of OH + VOCs (volatile organic compounds) ∕ CO ∕ HCHO ∕ CH4 ∕ H2 ∕ SO2 and HO2 + NO (Seinfeld, 1986). The reactivity from aerosol uptake cannot compete with the known gas-phase reactivity of OH, whereas it may compete with the reactivity of NO toward HO2 under some conditions such as low NO (Tang et al., 2017). For high aerosol mass load, the reaction rate of HO2 with aerosol particles could be fast enough to influence the concentration of HOx radicals and, consequently, reduce ozone production from HO2 + NO (Kanaya et al., 2009; Li et al., 2019b).
From a global perspective, the impact of HO2 uptake on the calculated HOx concentrations is diagnosed to be about 10 %–40 % (Jacob, 2000; Whalley et al., 2015, 2010; Mao et al., 2010; Li et al., 2019b, a), and often the value of (the heterogeneous uptake coefficient; Schwartz, 1984, 1986) is assumed to be a single value, 0.2 (Tie et al., 2001; Martin et al., 2003). The model results of HO2 uptake influence are lowered when a parameterized equation of is used without considering the influence of transition metal ions (TMIs) (Thornton et al., 2008). The reasons for the lower reactivity in the absence of TMIs include the lower reaction rate of aqueous HO2 ∕ O reactions (Thornton et al., 2008). However, in spite of the lower HO2 uptake coefficient used, a significant impact on the calculated [OH] and O3 production rate is suggested for air masses over the areas of Chinese megacities (Macintyre and Evans, 2011). A model study (Xue et al., 2014) considering the aerosol uptake of HO2 showed an impact on the simulated HO2 concentrations and local O3 production rates in Chinese urban regions: Beijing, Shanghai, and Guangzhou. Furthermore, researchers have proposed that in the North China Plain (Li et al., 2019a, b), the reduced HO2 uptake owing to reduction of the aerosol surface area is considered to be the key reason for the increased surface ozone concentration over the last few years when a value of 0.2 was used for .
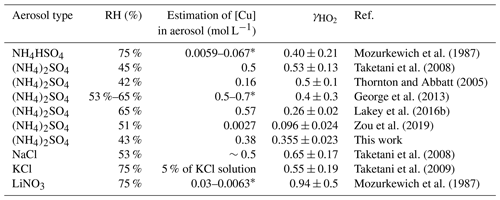
Previous studies show that the value of from the laboratory, field, and modelling studies spans several orders of magnitude, ranging from <0.002 for dry aerosols (Cooper and Abbatt, 1996; Taketani et al., 2008; George et al., 2013) to 0.2 for liquid deliquesced aerosols. Much higher values of have been measured and calculated for Cu-doped aerosols (Mozurkewich et al., 1987; Taketani et al., 2008; Thornton et al., 2008; Cooper and Abbatt, 1996; Lakey et al., 2016b; George et al., 2013). For fine particles, the reactions of HO2 with soluble Cu ions may be fast enough; thus the uptake coefficient is limited by the mass accommodation coefficient α. Due to the widespread distribution of Cu2+ ions in ambient particles, the absence of an accurate evaluation of is one of the largest uncertainties for the determination of the impact of HO2 uptake on pressing atmospheric issues such as ozone formation.
In this study, we reanalysed several datasets of the aerosol uptake of HO2 from laboratory studies reported in the literature and a new dataset of the HO2 uptake coefficient on Cu-doped ammonium sulfate aerosols at 43 % relative humidity as well as proposing a novel parameterized equation (abbreviated as NEq. in the paper) for the prediction of that best fits all the laboratory results. Furthermore, for the Wangdu field campaign, we also calculated according to the NEq. and the impact of HO2 uptake on HOx (=OH + HO2) budget was evaluated.
2.1 The model
A Multiphase Reaction Kinetic (MARK) model is developed in this study for the simulation of for the laboratory experiments. The reaction mechanism and reaction rate constants are summarized in Tables S1–S4 in the Supplement. The MARK model is currently capable of simulating inorganic deliquescent aerosol at ambient pressure and temperature. The model directly calculates the averaged quasi-first-order gas-phase HO2 uptake loss rate at steady state, khet (s−1) in Eq. (1). is retrieved by Eq. (2), considering the influence of the aerosol liquid water content (ALWC) (g cm−3) rather than surface density because of the influence of the RH on the uptake process (Kuang et al., 2018; Bian et al., 2014).
The meanings of the symbols are summarized in the Appendix. The units of aqueous reagents are converted to molec cm−3 in the model by kmt. To combine both gas-phase molecular diffusion and liquid-phase interface mass transport processes, the approach adopted uses one variable called kmt (Schwartz, 1984, 1986), which is used in the calculation for gas–liquid multiphase reactions in many modelling studies (Lelieveld and Crutzen, 1991; Chameides and Stelson, 1992; Sander, 1999; Hanson et al., 1994). The definition of kmt is given by Eq. (3):
The rate of gas-phase reactants (X) diffusing and dissolving to the condensed phase can be calculated in the framework of aqueous-phase reactions as (where X is the reactant molecule). Moreover, the conversion rate of aqueous-phase reactants to the gas phase can be calculated as . The unit of kmt is s−1, as kmt contains the conversion from m of the gas-phase molecule concentrations to m of the aqueous-phase molecule concentrations and in the other direction. For larger particles (radius > 1 µm), kmt is mainly determined by the gas-phase diffusion of HO2. For smaller particles (radius < 1 µm), kmt is mainly determined by the accommodation process. The MARK model can simultaneously simulate gas and liquid two-phase reaction systems in the same framework.
The aerosol particle condensed phase is not an ideal solution. Consequently, the effective Henry's law constant Hcc should be applied in the model calculation that takes into account the effects of solution pH and the salting out effect in the small gas-phase molecule (such as HO2, OH, and O2) due to the existence of electrolytes in the solution (Ross and Noone, 1991). This study uses the ISORROPIA II thermodynamic model (Fountoukis and Nenes, 2007) to calculate the ALWC and components' concentrations for metastable deliquescent aerosols. The effective Cu2+ concentration in the aqueous phase, which is strongly influenced by non-ideal solution ionic strength, is also calculated following Ross and Noone (Ross and Noone, 1991).
2.2 Corrections to in the MARK model
2.2.1 Henry's law of gas-phase reactants
The aerosol particle condensed-phase solution is not an ideal solution, as commented before. The addition of an electrolyte to water interferes with the gas dissolution and the organization of water molecules around the gas. This frequently results in a decrease in the solubility, or a salting out effect. This salting out effect is frequently a linear function of the molar ionic strength I. H0 is estimated to be about 3900 M atm−1 at 298 K for HO2 (Thornton et al., 2008; Golden et al., 1990; Hanson et al., 1992), and its temperature dependence is given accordingly to the IUPAC recommendation (Ammann et al., 2013; IUPAC Task Group on Atmospheric Chemical Kinetic Data Evaluation, http://iupac.pole-ether.fr, last access: 30 November 2020). H0 should be corrected by the solution pH and the salting out effect. In the MARK model, these two corrections are incorporated as Hcc:
The activity coefficient A for HO2 and other neutral small molecules such as H2O2 and O2 can be expressed as (Ross and Noone, 1991)
According to this correction, Hcc of HO2 increases with RH. The ratio of Hcc to H0 ranges from 0.03 (40 % RH, I=16.7) to 0.34 (80 % RH, I=5.5). Although the salting effect and the ionic strength are mainly driven by [NH] and (SO), ionic strength increases quickly from 5.9 to 9.5 with (Cu2+) from 0.1 to 1 M and limits the solubility of HO2 gas molecules.
2.2.2 Aerosol particle condensed-phase Cu2+ molality calculation
Inorganic species in ambient aerosol particles may be in the form of aqueous ions or in the form of precipitated solids in thermodynamic equilibrium with atmospheric gases and water vapour. The salts in the metastable aerosol are all dissolved in the aqueous phase. For metastable aerosols, this paper uses thermodynamic models to calculate ALWC and aerosol particle condensed-phase component concentrations. In this work the ISORROPIA II (Fountoukis and Nenes, 2007; Capps et al., 2012) thermodynamic equilibrium model for inorganic aerosol systems is used to take this into account.
At low relative humidity, the aqueous phase is highly concentrated (i.e. with a high ionic strength), and the solution is strongly non-ideal; consequently the activity coefficient and salting out effect must be taken into account for the calculation of aerosol chemistry. The ion activity coefficient refers to the effective concentration of ions participating in an electrochemical reaction in an electrolyte solution.
Since there is no direct evidence of the existence of Cu∕Fe redox reactions of HO2 which produce H2O rather than H2O2 as proposed by Mao et al. (2013), in the scope of this paper, HO2 uptake produces H2O separately by Cu- and Fe-free ions as proposed by many research studies (Mozurkewich et al., 1987; Hanson et al., 1992; Thornton and Abbatt, 2005; Thornton et al., 2008; Taketani et al., 2009; Macintyre and Evans, 2011). Fe-free ions can be seen as the equivalent Cu-free ions in the application of the MARK model or the parameterized equation mentioned below.
Based on Ross and Noone (1991), for an ion (xi) of charge zi (), the activity coefficient (φx) is
where D is given by Eq. (7):
I is the ionic strength of a solution [M], which can be calculated as the following equation:
where ε(xyI) denotes “interaction coefficients”, and the summation extends over all ions (y) in the solution at a molality of my. For ions of similar charge, ε(xyI) is set to zero. For ions of unequal charge, ε(xyI) may be calculated from the logarithm solution mean activity coefficient log (A±) (Clegg et al., 1998) of the single electrolyte at the same I according to Eq. (9):
In the condensed phase of aerosol particles, the effective molality of an ion xi ([xi]eff) can be calculated as
In the aerosol particle condensed phase, an effective concentration rather than the total concentration of the Cu ions should be calculated in catalytic aqueous reactions with HO2. The effective concentration of TMIs can be calculated as
where [Cu2+]eff is the effective aerosol condensed-phase soluble copper concentration. In this paper, [Cu]equ represents the equivalent copper concentrations from other TMIs such as Fe and Mn.
2.2.3 The conversion formula of [] and HO2(r)
Gas-phase HO2 molecules dissolve in the particle condensed phase and diffuse from the surface of a particle toward the centre in parallel with aqueous-phase reactions. We need to evaluate , the assumed averaged steady-state HO2 concentration over the volume of the particle. [HO2(r)] is HO2 concentration at the surface of particles. The ratio of these two concentrations can be calculated as (Schwartz, 1986, 1984)
where q is given by Eq. (13):
and Daq is the aqueous-phase diffusion coefficient [cm2 s−1], keff is the comprehensive liquid-phase reaction rate coefficient which encompasses both HO2 dissolution equilibrium reactions and liquid-phase chemical-physical reactions during HO2 uptake process. keff is calculated by Eq. (19) and includes the influence of the salting out effect of HO2 and the ionic strength effects on TMIs. keff will change dramatically according to the concentration of equivalent copper ions and the diameter of particles. Higher Cu concentration will make the ratio smaller and cause larger uncertainties, however, in the copper-doped aerosol particle, because of the high value of keff (typical value is 2.9×106 M s−1 with 1 M Cu2+ and 3.25×104 M s−1 with 0.001 M Cu2+) and small count median diameter (Rd) (usually smaller than 1 µm), the ratio is close to 1. At a diameter of 100 nm, and a relative humidity between 40 % and 90 %, the condensed-phase copper ion concentration varies from 10−5 to 1 M, the average ratio of the surface HO2 concentration and the condensed-phase HO2 concentration is beyond 0.87 at every Cu concentration. The ratios are calculated by simulation of keff and the accordingly calculations by Eqs. (12) and (13). Thus, in this model, we assume the surface concentration of HO2 equals to the condensed-phase average HO2 concentration.
2.3 Laboratory results for the HO2 accommodation coefficient
The accommodation coefficient of HO2 () is independent of the concentrations of Cu-free ions if the viscosity of particles is maintained, while the more accurate accommodation coefficient can only be measured with no limitation of the aqueous mass transfer flux; in this situation, is equal to . HO2 uptake coefficients are summarized for copper-doped inorganic aerosol particles from various previous laboratory studies. The uptake coefficient of HO2 is approximately 0.5 for sulfate aerosol and even higher for chlorine or nitrate aerosol because of the catalytic effect of Cu2+ on aqueous HO2 ∕ O (Table 1). In this situation, the aqueous reactions are fast enough for the uptake process to be limited primarily by the mass transport process (accommodation), and the uptake coefficient is equal to the accommodation coefficient. Thus, the MARK model typically uses of 0.5. We also tested the influence of the accommodation coefficient on the calculated HO2 uptake coefficient in a field campaign; for details, please see the Supplement.
2.4 The experimental setup and methodology of the latest results of
In this study, we also included the latest results which were measured at Leeds. The experimental setup and methodology used to make the new measurements of γ(HO2) reported here have been described in detail elsewhere (Moon et al., 2018; Lakey et al., 2016b; George et al., 2013), and so only brief details are given here. In summary, the experiments were performed by moving an HO2 injector backwards and forwards along the concentric axis of a laminar aerosol flow tube, hence changing the contact time between HO2 and the aerosols. Measurements of [HO2] were performed using laser-induced fluorescence (LIF) spectroscopy at low pressure (the fluorescence assay by gas expansion (FAGE) technique (Heard and Pilling, 2003)), and the total aerosol surface area was determined with a Scanning Mobility Particle Sizer (SMPS) at the end of the flow tube. Aerosol particles were produced using a constant output atomizer (TSI, 3076), and the aerosol concentration and hence surface area could be varied, being controlled using a high-efficiency particulate air (HEPA) filter in a bypass arrangement. Atomizer solutions were prepared by dissolving 0.01 mol of ammonium sulfate (AS) (Fisher Scientific, >99 %) with varying amounts of copper (II) sulfate (Fisher Scientific, >98 %) in 500 mL of Milli-Q water. The data were analysed as described in George et al. (2013). The pseudo-first-order loss rate coefficient (k′) was obtained from the gradient of a plot of ln(HO2 signal) against the interaction time between HO2 and the aerosol before sampling by the FAGE detector. The uptake coefficient (γ(HO2)) was obtained from the linear least-squares gradient of the plot of k′ against the surface area concentration of aerosols in the flow tube. The error given on all measurements of γ(HO2) represents 2σ of the uncertainty of the fitted gradient. A correction to k' was applied to take into account non-plug flow conditions in the flow tube using the Brown method.
3.1 Parameter sensitivity analysis of the MARK model
Hygroscopic inorganic particles are one of the most important components of PM2.5 in ambient air. The annual average contribution of inorganic aerosol to PM2.5 is between 25 % and 48 % across China (Tao et al., 2017), especially NH, SO, NO, and other inorganic ions. In laboratory studies of radical heterogeneous reactions, (NH4)2SO4 aerosol is most widely studied because of its simple components and its easy way of being generated and because it is an important component of urban aerosols (Cheng et al., 2012; Yin et al., 2005). A simplified approach was used to explore the mechanism of HO2 heterogeneous uptake to derive a parameterized equation for the uptake coefficient, .
In this study, (NH4)2SO4 aerosol uptake reactions of HO2 are simulated by the MARK model, and good correlation between simulation results and experimental results is obtained, especially considering the influence of both [Cu2+] and RH (for the lack of measured data the parameterized equation is only valid in the range of 40 % to 90 % RH).
Figure 1 shows the influences of both factors, RH and condensed-phase pH together with Cu+2 concentration, on the heterogeneous process of HO2. As the RH rises, the exhibits a logarithmic growth. Higher RH means a higher water content, which dilutes the bulk-phase ions and thus promotes the activity coefficients of reactant ions in the aerosol particle condensed phase and the solubility of gas-phase reactants such as OH, HO2, and H2O2.
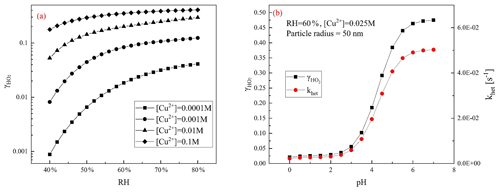
Figure 1Influence of various parameters upon predicted by the MARK model. (a) increases with the RH at different [Cu2+]; (b) denoted by black squares and black line and khet in red circles and red line increase with aerosol particle condensed-phase pH.
presents a sigmoid-shaped growth with aerosol particle condensed-phase pH. In the model, it is found that as the pH rises, the uptake coefficient rises rapidly because HO2 is a weak acid (pKa = 4.7) and has a low solubility in an acidic environment. The higher condensed-phase pH is favourable for the dissolution equilibrium of the gas-phase HO2. This trend is consistent with the observed second-order rate constant of HO2 ∕ O reviewed by Bielski et al. (1985). Moreover, aqueous-phase reaction rates of HO2 ∕ O and Cu2+ ∕ Cu+ increase with an increase of condensed-phase pH because in an alkaline environment HO2 is more dissociated to O, which has a quicker reaction rate with Cu2+ ∕ Cu+. The pH of the ambient atmospheric aerosol is measured to be generally below 5, even when the concentration of NH3 is high as in Beijing and Xi'an (Ding et al., 2019; Guo et al., 2017) with a range of 3–5. In this range, is highly affected by aerosol condensed-phase pH, mainly because of the change of HO2 solubility.
3.2 Model validation
Although the MARK model simulation results in this paper are not obtained by adjusting parameters to fit the experimental data points, the MARK model fitted well with these results under different ambient RH and Cu2+ concentrations.
At present, there are experimental measurements of at different RHs (Thornton et al., 2008; Taketani et al., 2008, 2009; Taketani and Kanaya, 2010; Taketani et al., 2012; Matthews et al., 2014; Thornton and Abbatt, 2005), but there is no systematic experimental study of this dependence, where only RH is changed and not other parameters. Many research studies proposed that is higher for aqueous inorganic aerosol than for dry inorganic aerosol. Although previous experiments did not directly measure the dependence of RH, the change of the uptake coefficient met the simulation trend (see Fig. 2). For hygroscopic inorganic aerosols, RH significantly affects the aerosol liquid water content, changing its ionic strength, aqueous reagent activity coefficients, and the solubility of gas-phase reactants such as OH, HO2, and H2O2.
Aerosol condensed-phase copper ion concentration is another important factor of HO2 uptake by adjusting the aqueous reaction rates between HO2 ∕ O and Cu. As shown in Fig. 2. when the condensed-phase copper ion concentration is less than 1– M, the heterogeneous uptake of HO2 is not significant and may mainly be driven by the self-reaction of HO2. This threshold is consistent with the results of previous research studies (Mozurkewich et al., 1987; Lakey et al., 2016b). The threshold is also consistent in different heterogeneous media of aerosol and cloud or rain droplets. As the copper concentration increases, the solution ionic strength increases, and rapidly rises to the limit of the accommodation coefficient and the limitation of the HO2 solubility.
What is more, laboratory measurement uncertainties will directly influence the evaluation of the deviation between the modelled HO2 uptake coefficient and the measured results because all the parameters inputted in the MARK model are in reference to the measurement conditions. However, it is difficult to calculate the detailed uncertainties from all factors that influence because of the non-linear reaction system. Uncertainties of the experimental conditions such as RH and particle diameters are combined into the reported values of . Taking all these into account, we calculated an averaged uncertainty for the experimental values of in different ranges of Cu ion concentration. Laboratory measurement uncertainty has the largest value of 35.1 % in the range of to 0.01 M soluble copper concentration, 14.9 % below M, and 9.3 % higher than 0.01 M. In general, good agreement is achieved between the MARK model results and the results of the previous laboratory studies, which were also classified based on a statistical parameter, the root mean square error (RMSE) (Fig. 2). In this paper, the relative error of each measured data point is considered to calculate the weighted average in RMSE:
where is the MARK model result at each Cu2+ concentration and RH, is the central value of each measurement result, and ωi is its corresponding relative error.
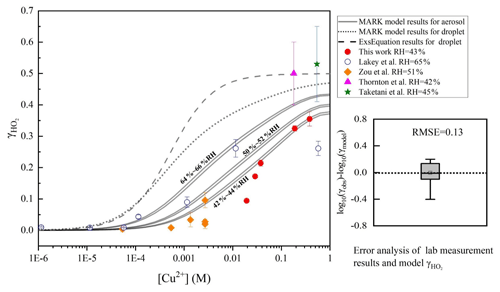
Figure 2Dependence of on aerosol copper concentration. Red filled circles denote the results at 43% RH measured at Leeds included in this paper. Blue hollow circles at 65 % RH (Lakey et al., 2016b). Yellow filled diamonds denote results at 51 % RH (Zou et al., 2019), filled purple triangle at 42 % RH (Thornton and Abbatt, 2005), and filled green star at 45 % RH (Taketani et al., 2008). The dashed grey line denotes the results of the classical parameterized equation (named CEq. in this paper) with deliquesced aerosol particles (Thornton et al., 2008; Hanson et al., 1992, 1994; Jacob, 2000; Kolb et al., 1995), which was confirmed by research studies of reactive gas molecular uptake on dilute solution droplets (Magi et al., 1997) and on aqueous surfaces (Utter et al., 1992; Müller and Heal, 2002; Hu et al., 1995). The solid grey lines represent the model results of the MARK model in this study at various RHs (two lines represent the range of RH from 64 % to 66 %, 50 % to 52 %, and 42 % to 44 %), and the short dotted line represents the result in the MARK model of HO2 with dilute solution droplets. The root mean square error (RMSE) between the MARK modelled values and the full dataset is 0.13. The aerosol pH is set as 4.5 based on the aqion 7.0.8 interface, considering the participation of Cu ions (for details, please see https://www.aqion.de/, last access: 30 November 2020).
3.3 Comparison of the classical parameterized equation and the MARK model
The classical parameterized equation (CEq.) (Thornton et al., 2008; Hanson et al., 1992, 1994; Jacob, 2000; Kolb et al., 1995; Ammann et al., 2013; IUPAC Task Group on Atmospheric Chemical Kinetic Data Evaluation, http://iupac.pole-ether.fr, last access: 30 November 2020) proposed for the HO2 uptake of deliquesced aerosol particles has been proved to provide good estimation of the reactive gas molecular uptake coefficient on dilute solution droplets (Magi et al., 1997) and on aqueous surfaces (Utter et al., 1992; Müller and Heal, 2002; Hu et al., 1995).
When the classical parameterized equation (CEq.) is applied to the calculate HO2 uptake coefficient with copper-doped aerosol, CEq has a higher deviation of between the measured results compared to the MARK model. All input parameters are the same, except that the MARK model involved more liquid-phase reactions instead of only considering the second-order rate coefficient (kTMI) of HO2 and O with transition metal ions as the CEq. did. kTMI is the most important parameter in the calculation of the uptake coefficient. Based on the research by Bielski et al. (1985), we used the effective rate constant of HO () with Cu ions as 1.5×107 M−1 s−1 rather than the more commonly used value of 1×109 M−1 s−1 considering the pH limitation (pH is about 3–5 in the ambient aerosol particle condensed phase as discussed above). The prior value (1.5×107 M−1 s−1) reflects the rate of reaction between HO2 and Cu2+, more prevalent in acidic aerosol such as ammonium sulfate, and the latter (1×109 M−1 s−1) between O and Cu2+ ions, which is more prevalent in aerosols with a pH greater than the pKa of HO2, such as NaCl (Bielski et al., 1985). This treatment within the calculation can bring predictions more in line with experimental results in the CEq., as shown by the dashed line in Fig. 2.
IUPAC (Ammann et al., 2013; IUPAC Task Group on Atmospheric Chemical Kinetic Data Evaluation, http://iupac.pole-ether.fr, last access: 30 November 2020) proposed the effective rate coefficient k1 for the reaction of HO2_total () with Cu ions as 5×105 M−1 s−1 to achieve the best fit based on the calculation results from Lakey et al. (2016b). The low reaction rates used here in the CEq. are assumed to likely result from the combined effects of solute strength effects as discussed by Lakey et al. (2016b). The MARK model uses the same framework with the CEq and considers the Setchenov salting out and ionic strength effects on HO2 uptake more comprehensively and in more detail and proposes keff as the effective reaction coefficient (Eq. 19). Considering the small RMSE between the MARK model and the laboratory studies, we proposed a novel parameterized equation (NEq.) to better describe the influence of [Cu2+] and RH on .
3.4 A novel parameterized equation of
When the full reaction system reaches steady state, the reaction of HO2 in the aqueous particle phase can be expressed as the following reaction scheme (Schwartz, 1984, 1987; Schwartz and Freiberg, 1981):
Gas-phase HO2(g) molecules transport onto the surface of the aerosol particles; HO2(r) then dissolves in the condensed phase to give HO2(a). The reactions between Cu2+ ∕ Cu+and HO2 can be seen as catalytic reactions because in the model simulations, the total amount of [Cu2+] + [Cu+] does not change with reaction time. The rate of HO2 aqueous reaction with copper ions is noted as keff. For fine particles, we can safely assume that the interface concentration [HO2(r)] is equal to the condensed-phase average concentration due to rapid diffusion in the liquid phase (details have been discussed in Sect. 2.2.3). For the submicrometer aerosol particles with which most uptake reaction occurs, the influence of the gas-phase diffusion limitation can be neglected. Hanson et al. (1994) proposed the definition of the uptake coefficient as ), where ca,surf is the surface concentration of the reactant, and cg,surf is the gas-phase concentration. In the process of HO2 uptake, we deduce the parameterized equation (NEq.) of in the framework of the resistance model:
From Eq. (18), it can be deduced that can be calculated by optimizing keff under different ambient environmental conditions from the MARK model results. The MIPFIT model (Markwardt, 2009; Lewis et al., 2009) in the IDL software program is used to optimize keff using the Levenberg–Marquardt algorithm. Because the equation is empirical, the initial value of keff is set as 1. keff is related to [Cu2+]eff, which is the sum of condensed-phase soluble copper concentration and other equivalent copper concentrations mentioned (Eq. 11). The exponent of [Cu2+]eff is globally fitted using the MIPFIT method. It is found that the overall R2 is higher than 0.97 and the residual is minimized when the exponent is 0.65. f(ALWC,[PM]) has a negative exponential relationship to [PM] and has a positive linear relationship to RH.
We further calculated the RMSE of the modelled data and NEq. (Eq. 15) data under different RH conditions. The range of values shows the difference between the modelled data and NEq. data at different Cu2+ concentrations. At low RH and consequently relatively low ALWC, is more sensitive to [Cu2+], especially at low [Cu2+]. This sensitivity cannot be fully represented in the parameterized equation. What is more, at low [Cu2+] and low RH, the value of is smaller than in other conditions, so the uncertainty of becomes larger.
All the RMSE values are smaller than 0.2, which indicates a minor deviation from the laboratory results in our equation. In the typical ambient urban atmospheric environment, with an aerosol mass concentration of 10–300 µg m−3, aqueous Cu2+ concentration of 10 molar concentration, and a relative humidity between 40 %–90 %, the NEq. can be used. Beyond this range, the application of the NEq. may cause a large deviation. The HO2 uptake under dry conditions needs further investigation in the future, but it is probably not of high priority because the effective reaction volume becomes 10 % or less of the aerosol volume for dry conditions, and the HO2 uptake may then be neglected for typical tropospheric conditions (Taketani et al., 2008; Kanaya et al., 2009; Taketani and Kanaya, 2010; Thornton et al., 2008; George et al., 2013).
3.5 Evaluation of the impact of the new HO2 uptake parameterization in the Wangdu campaign
Many model studies (Lakey et al., 2015; Martinez et al., 2003; Tie et al., 2001; Whalley et al., 2015) suggest that the heterogeneous uptake of HO2 radicals affects the global distribution of trace gases and the atmospheric oxidant capacity, especially in regions with high aerosol loading or low NOx concentration. The importance of aerosol chemistry as a sink for ozone precursors in North China Plain has been suggested in many model studies (Li et al., 2019b; Lou et al., 2014). The competition of HO2 with aerosol and gas-phase reactants is crucial when evaluating the influence of heterogeneous reactions on the atmospheric oxidant capacity.
Based on the results of a comprehensive field campaign performed in summer 2014 at a rural site (Wangdu) in the North China Plain (Tan et al., 2020), the HO2 uptake coefficient and the ratios of the HO2 uptake loss rates (TR) to the sum of the ROx termination rates (TR) are calculated with direct measurements of the ROx radicals, trace gas species, ALWC, and the aerosol condensed-phase component concentrations (please see the Supplement for details). The experimental determined ROx termination rates include reaction channels from OH + NO2, OH + NO, HO2 + HO2, HO2 + RO2, and RO2 + NO. Considering the solubility and size distribution of particle metal copper (Fang et al., 2017; Hsu et al., 2010a; Mao et al., 2013), we can estimate in the daytime and night-time.
3.5.1 Average results of observed meteorological parameters and trace gas concentration in the Wangdu campaign
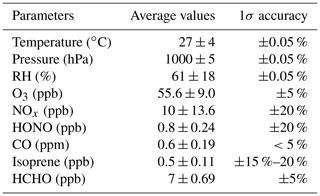
Wangdu is located in the centre of the Beijing–Tianjin–Hebei area, and it is a regional site. The observations were carried out in the summer with serious photochemical smog pollution events (Tan et al., 2017, 2020). Table 2 summarizes the meteorological and chemical conditions in this field campaign. In terms of parameters such as temperature, pressure, and relative humidity, the Wangdu area is a high-temperature and high-humidity environment with a monsoon climate.
3.5.2 Calculation of soluble copper ion concentration
During this campaign, the total concentration of heavy metal ions in fine particles (smaller than 2.5 µm) was measured using a commercial instrument based on a non-destructive X-ray fluorescence technique (Xact 625, Cooper Environmental). Since the concentration of soluble copper concentration rather than total copper concentration is used in the model, it is necessary to analyse the ratio of soluble copper to total copper in the aerosol particles. For particle radii smaller than 2.5 µm of which are the bins of aerosols that contribute most to HO2 uptake, the mass fraction of Cu is about 33 % to −100 µm% compared with two other size bins in ambient aerosols (2.5–10 µm, >10 µm) (Mao et al., 2013). According to previous research results, the dissolution ratio of copper in aerosol particles varies from 20 % to 70 % in different regions, the solubility being lower in smaller particles (Fang et al., 2017; Hsu et al., 2004, 2010b). Therefore, when using the NEq. to calculate the HO2 heterogeneous uptake coefficient, it is necessary to reduce the copper concentration considering the solubility and the distribution in the accumulation mode of aerosol particles. We assume 50 % copper is soluble in the particle condensed phase, and 50 % copper is in the accumulation mode. Thus, we assume 25 % of total aerosol metal copper concentration is soluble in the accumulation mode when calculating in the Wangdu campaign. The hourly resolution total copper concentration (ng m−3) is divided by the aerosol volume concentration and the atomic mass of copper (64) to obtain the total copper molar concentration in the aerosol (mol L−1). rather depends on copper concentration, so we also evaluate the influence of copper solubility on the uptake coefficient. What is more, the unequal distribution of copper ions will also influence the HO2 uptake coefficient (details in the Supplement).
3.5.3 estimated in the Wangdu field campaign
By inputting the soluble copper concentration, aerosol mass concentration, aerosol particle geometric mean diameter, and the corresponding relative humidity and temperature into the NEq., we can obtain an estimation of in suburban Wangdu, which is shown in Fig. 4a and b. The time resolution is 1 h. The aerosol pH is calculated using the thermodynamic model ISORROPIA-II (Fountoukis and Nenes, 2007), and the averaged value is 3.41 ± 0.69 (1σ). The average aerosol mass concentration is 67.2 ± 39.7 µg m−3, and the average Cu concentration is 35.8 ± 57.7 ng m−3. The fit to a Gaussian function results in a value of 0.116 ± 0.086 (1σ) in the Wangdu campaign ( will increase from 0.065 ± 0.051 (1σ) at 10 % solubility to 0.196 ± 0.142 (1σ) at 70 % solubility for the summary of daytime and night-time data).
Tan et al. (2017) compared the measured and modelled OH, HO2, and RO2 radicals in the Wangdu campaign. However, in this paper, they did not discuss the influence of HO2 uptake. A very recent publication (Tan et al., 2020) calculated in the Wangdu campaign based on the comparison of field measurement data for HO2 and concentrations calculated by the box model. The paper proposes that all calculated in this way from the Wangdu campaign can be fitted to a Gaussian distribution around the value of 0.08 ± 0.13 (1σ). This value is in the range of our estimation in this paper, considering the influence of aerosol morphology and the indirect measurement uncertainty (please see the Supplement).
The experimentally determined ROx termination rates include reaction channels from OH + NO2, OH + NO, HO2 + HO2, HO2 + RO2, and RO2 + NO. The ratio (R1) of the HO2 uptake loss rate () to the whole ROx loss rate () is calculated by Eqs. (21) and (22).
[ASA] is the aerosol surface area (µm2 cm−3).
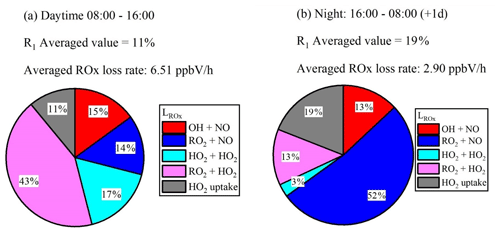
Figure 3R1 calculated by the NEq. Pie charts show the values of R1 and the loss rates for ROx during daytime (a) and night-time (b). The averaged daytime (08:00–16:00) ROx radical loss rate is 6.5 ppbV h−1, and that for night-time (16:00–08:00 (+1 d)) is 2.9 ppbV h−1.
No significant difference of is observed during daytime and night-time. The HO2 uptake coefficient is slightly higher at night due to the higher RH (57.6 % in the day and 67.4 % at night). However, because of the high uncertainty of the uptake coefficient, such a high trend cannot be concluded for other cases. HO2 heterogeneous uptake reactions with aerosol particles have a small impact on ROx radical termination in the daytime, as shown in Fig. 4a. However, HO2 uptake may be important in the termination of ROx radicals at night, shown in Fig. 4b. The daytime ratio R1 is lower than it is at night because of the lack of photochemical reactions and thus a longer HO2 lifetime at night. The high proportion of RO2 + NO during night-time is due to high [NO] at dawn.
The RO2 concentration is also important when evaluating the impact of HO2 uptake. Using the modelled value of RO2 concentration in the Wangdu campaign, a higher proportion of HO2 uptake to about 21 % of ROx sinks in the daytime can be calculated. However, using the modified field measured RO2 concentration in the Wangdu campaign, HO2 uptake is less important in the budget of ROx as shown in Fig. 4a, which is in line with the results from Tan et al. (2020).
3.5.4 Discussion of uncertainties of estimated in the Wangdu field campaign
The impact of HO2 aerosol uptake on the ROx budget is complicated by large uncertainties in the HO2 uptake coefficient under ambient conditions. The NEq. is applicable under the assumption of steady-state concentrations and with metastable or liquid aerosol particles (if the ambient RH over a completely liquid aerosol decreases below the deliquescence RH, the aerosol may not crystalize immediately but may constitute a supersaturated aqueous solution (i.e., in the metastable state) (Song et al., 2018)). The approximate calculation of HO2 concentration gradients within the aerosol particle condensed phase also cause deviations for larger particles or high copper equivalent concentration.
Organic content of an aerosol particle may affect several important parameters in the uptake model (Lakey et al., 2016b, 2015). For example, the aerosol pH, hygroscopic properties of the aerosol, the rate of diffusion of HO2 within the aerosol, and a reduction in the concentration of Cu2+ via the formation of complexes could affect the ability of Cu to undergo redox reactions with HO2 and O. Hence, it is expected that the presence of organic matter would change the value of . We tested the core-shell morphology of aerosol particles' influence on HO2 uptake in the Wangdu campaign (details in the Supplement). Organic matter will lower the uptake coefficient about 25 % to 40 % under the assumption that 20 %–50 % PM2.5 mass is organic matter.
Another uncertainty comes from aerosol particles' morphology. The bulk diffusion coefficient of HO2 and other reactive molecules should be lower in the situation of semi-solid particles (Berkemeier et al., 2016; Shiraiwa et al., 2010; Mikhailov et al., 2009) and would change with the water activity and the organic components (Price et al., 2015). For crystalline or amorphous solid aerosol particles, HO2 will undergo surface reactions and diffuse across the surface rather than be accommodated within the aerosol bulk. The MARK model has limitations in the calculation of with semi-solid aerosol particles. In the Wangdu campaign, κsca (optical aerosol hygroscopicity parameter) ranges from 0.05 to 0.35, with an average of 0.22. The ambient RH during the Wangdu campaign shows significant diurnal variations and varies greatly from 15 % to 97 %, with an average value of 61 % (Kuang et al., 2019), indicating that the percentage of solid aerosol particles is relatively low and hence does not significantly influence . In any case, aerosol particles' morphology relative to an aqueous phase will influence the uptake coefficient of HO2. The uptake process would vary with mixing state of the particles; thus the predicted values here may be biased as a result but represent an average over bulk aerosols.
The interaction between organics and soluble copper and the influence of organics on aerosol properties will lead to further uncertainty in the calculation of the uptake coefficient. Lakey et al. (2016a, b, 2015) also showed that the addition of an organic compound to Cu2+-doped aerosols such as oxalic acid, which forms oxalate ions (C2O4)2− in the aerosol, results in a lower value of as such ions form a complex with the TMIs.
As noted above, the value (0.116 ± 0.086 (1σ)) estimated by the NEq. represents the upper limitation of in the Wangdu field campaign.
Taketani et al. (2012) collected the filter samples of aerosol in Mt. Tai and Mt. Mang, North China, and re-aerosolized from the water extracts of sampled particles. The measured uptake coefficients for Mt. Tai samples ranged between 0.09 and 0.40, while those at Mt. Mang were between 0.13 and 0.34. Li et al. (2019b) suggest that the rapid decrease of PM2.5 in China has slowed down the reactive uptake rate of HO2 radicals by aerosol particles and could have been the main reason for the increase in ozone in the North China Plain in recent years. They apply a value of the uptake coefficient of 0.2 in their model calculations. However, the results of the MARK model and of the NEq. in this paper suggest that the HO2 uptake coefficient could be smaller and highly variable for typical conditions in the North China Plain. Further research is needed to study the effects of the heterogeneous uptake of HO2 in the gas phase and heterogeneous physicochemical reactions under different environmental conditions in different regions. The novel parameterized equation proposed in this paper provides an effective way for a more detailed calculation of the effects of HO2 heterogeneous reactions on the atmospheric radical budget, ozone production, and particulate matter generation. This is the first attempt to parameterize the heterogeneous uptake coefficient of HO2 with aerosol particles in a campaign in China. This equation estimates the in a comprehensive field campaign, which is in agreement with the simulation results from the comparison of gas-phase radical concentrations (Tan et al., 2020). Overall, we can conclude that the HO2 uptake process needs to be considered in photochemical box models for the study of the HOx radical budget. The exact value is highly variable with respect to the change of copper concentrations in the aerosol particle condensed phase and other factors. The measurement of condensed-phase soluble copper and other TMIs and organic content, as well as the aerosol liquid water, should be included in future field campaigns for the study of the HOx radical budget.
Data supporting this publication are available upon request from the corresponding author (k.lu@pku.edu.cn).
The supplement related to this article is available online at: https://doi.org/10.5194/acp-20-15835-2020-supplement.
KL conceived the study. HS and KL developed the MARK model for multiphase simulations. XC and QZ improved the codes of the MARK model. ZT, HF, KL, AW, MZ, AW, AKS, and YZ contributed to the related measurements of the Wangdu field campaign. DEH, DRM, and MTBR contributed the laboratory studies of HO2 uptake coefficients, and they contributed to the writing of the manuscript. HS performed the model simulations and prepared the manuscript with KL and ZT, which was enhanced by contributions from all the co-authors.
The authors declare that they have no conflict of interest.
We gratefully acknowledge the kind help from Lisa Whalley for her technical help of the HO2 uptake measurement and the science team of the Wangdu field campaign.
This research has been supported by the Beijing Municipal Natural Science Foundation for Distinguished Young Scholars (grant no. JQ19031), the National Natural Science Foundation of China (grant nos. 21976006, 91544225, 21522701, and 91844301), and the National Research Program for Key Issues in Air Pollution Control (grant no. DQGG0103-01).
This paper was edited by Markus Ammann and reviewed by four anonymous referees.
Ammann, M., Cox, R. A., Crowley, J. N., Jenkin, M. E., Mellouki, A., Rossi, M. J., Troe, J., and Wallington, T. J.: Evaluated kinetic and photochemical data for atmospheric chemistry: Volume VI – heterogeneous reactions with liquid substrates, Atmos. Chem. Phys., 13, 8045–8228, https://doi.org/10.5194/acp-13-8045-2013, 2013.
Berkemeier, T., Steimer, S. S., Krieger, U. K., Peter, T., Pöschl, U., Ammann, M., and Shiraiwa, M.: Ozone uptake on glassy, semi-solid and liquid organic matter and the role of reactive oxygen intermediates in atmospheric aerosol chemistry, Phys. Chem. Chem. Phys., 18, 12662–12674, 2016.
Bian, Y. X., Zhao, C. S., Ma, N., Chen, J., and Xu, W. Y.: A study of aerosol liquid water content based on hygroscopicity measurements at high relative humidity in the North China Plain, Atmos. Chem. Phys., 14, 6417–6426, https://doi.org/10.5194/acp-14-6417-2014, 2014.
Bielski, B. H., Cabelli, D. E., Arudi, R. L., and Ross, A. B.: Reactivity of HO2/O radicals in aqueous solution, Journal of physical and chemical reference data, 14, 1041–1100, 1985.
Capps, S. L., Henze, D. K., Hakami, A., Russell, A. G., and Nenes, A.: ANISORROPIA: the adjoint of the aerosol thermodynamic model ISORROPIA, Atmos. Chem. Phys., 12, 527–543, https://doi.org/10.5194/acp-12-527-2012, 2012.
Chameides, W. L. and Stelson, A. W.: Aqueous-phase chemical processes in deliquescent seasalt aerosols, Ber. Bunsen Phys. Chem., 96, 461–470, 1992.
Cheng, M. C., You, C. F., Cao, J. J., and Jin, Z. D.: Spatial and seasonal variability of water-soluble ions in PM2.5 aerosols in 14 major cities in China, Atmos. Environ., 60, 182–192, https://doi.org/10.1016/j.atmosenv.2012.06.037, 2012.
Clegg, S. L., Brimblecombe, P., and Wexler, A. S.: Thermodynamic model of the system at tropospheric temperatures, J. Phys. Chem. A, 102, 2137–2154, https://doi.org/10.1021/jp973042r, 1998.
Cooper, P. L. and Abbatt, J. P. D.: Heterogeneous interactions of OH and HO2 radicals with surfaces characteristic of atmospheric particulate matter, J. Phys. Chem.-Us., 100, 2249–2254, https://doi.org/10.1021/Jp952142z, 1996.
Ding, J., Zhao, P., Su, J., Dong, Q., Du, X., and Zhang, Y.: Aerosol pH and its driving factors in Beijing, Atmos. Chem. Phys., 19, 7939–7954, https://doi.org/10.5194/acp-19-7939-2019, 2019.
Fang, T., Guo, H., Zeng, L., Verma, V., Nenes, A., and Weber, R. J.: Highly Acidic Ambient Particles, Soluble Metals, and Oxidative Potential: A Link between Sulfate and Aerosol Toxicity, Environ. Sci. Technol., 51, 2611–2620, https://doi.org/10.1021/acs.est.6b06151, 2017.
Fountoukis, C. and Nenes, A.: ISORROPIA II: a computationally efficient thermodynamic equilibrium model for K+-Ca2+-Mg2+-NH-Na+-SO-NO-Cl−-H2O aerosols, Atmos. Chem. Phys., 7, 4639–4659, https://doi.org/10.5194/acp-7-4639-2007, 2007.
George, I. J., Matthews, P. S. J., Whalley, L. K., Brooks, B., Goddard, A., Baeza-Romero, M. T., and Heard, D. E.: Measurements of uptake coefficients for heterogeneous loss of HO2 onto submicron inorganic salt aerosols, Phys. Chem. Chem. Phys., 15, 12829–12845, 2013.
Golden, D. M., Bierbaum, V. M., and Howard, C. J.: Re-evaluation of the bond-dissociation energies (Delta-Hdbe) for H-OH, H-OOH, H-OO-, H-O., H-OO-, and H-OO, J. Phys. Chem.-Us., 94, 5413–5415, https://doi.org/10.1021/j100376a046, 1990.
Guo, H., Weber, R. J., and Nenes, A.: High levels of ammonia do not raise fine particle pH sufficiently to yield nitrogen oxide-dominated sulfate production, Sci. Rep.-Uk, 7, 12109, https://doi.org/10.1038/s41598-017-11704-0, 2017.
Hanson, D. R., Burkholder, J. B., Howard, C. J., and Ravishankara, A. R.: Measurement of OH and HO2 radical uptake coefficients on water and sulfuric-acid surfaces, J. Phys. Chem.-Us., 96, 4979–4985, https://doi.org/10.1021/J100191a046, 1992.
Hanson, D. R., Ravishankara, A. R., and Solomon, S.: Heterogeneous reactions in sulfuric-acid aerosol: A framework for model calculations, J. Geophys. Res.-Atmos., 99, 3615–3629, https://doi.org/10.1029/93jd02932, 1994.
Heard, D. E. and Pilling, M. J.: Measurement of OH and HO2 in the troposphere, Chem. Rev., 103, 5163–5198, https://doi.org/10.1021/cr020522s, 2003.
Hsu, S.-C., Liu, S. C., Lin, C. Y., Hsu, R. T., Huang, Y. T., and Chen, Y. W.: Metal compositions of PM10 and PM2.5 aerosols in Taipei during spring, 2002, Terr. Atmos. Ocean. Sci., 15, 925–948, https://doi.org/10.3319/tao.2004.15.5.925(adse), 2004.
Hsu, S.-C., Wong, G. T. F., Gong, G.-C., Shiah, F.-K., Huang, Y.-T., Kao, S.-J., Tsai, F., Candice Lung, S.-C., Lin, F.-J., Lin, I. I., Hung, C.-C., and Tseng, C.-M.: Sources, solubility, and dry deposition of aerosol trace elements over the East China Sea, Mar. Chem., 120, 116–127, https://doi.org/10.1016/j.marchem.2008.10.003, 2010a.
Hsu, S.-C., Liu, S. C., Tsai, F., Engling, G., Lin, II, Chou, C. K. C., Kao, S. J., Lung, S. C. C., Chan, C. Y., Lin, S. C., Huang, J. C., Chi, K. H., Chen, W. N., Lin, F. J., Huang, C. H., Kuo, C. L., Wu, T. C., and Huang, Y. T.: High wintertime particulate matter pollution over an offshore island (Kinmen) off southeastern China: An overview, J. Geophys. Res.-Atmos., 115, https://doi.org/10.1029/2009jd013641, 2010b.
Hu, J., Shi, Q., Davidovits, P., Worsnop, D., Zahniser, M., and Kolb, C.: Reactive uptake of Cl2(g) and Br2(g) by aqueous surfaces as a function of Br−and I−ion concentration: The effect of chemical reaction at the interface, J. Phys. Chem., 99, 8768–8776, 1995.
Jacob, D. J.: Heterogeneous chemistry and tropospheric ozone, Atmos. Environ., 34, 2131–2159, https://doi.org/10.1016/s1352-2310(99)00462-8, 2000.
Kanaya, Y., Pochanart, P., Liu, Y., Li, J., Tanimoto, H., Kato, S., Suthawaree, J., Inomata, S., Taketani, F., Okuzawa, K., Kawamura, K., Akimoto, H., and Wang, Z. F.: Rates and regimes of photochemical ozone production over Central East China in June 2006: a box model analysis using comprehensive measurements of ozone precursors, Atmos. Chem. Phys., 9, 7711–7723, https://doi.org/10.5194/acp-9-7711-2009, 2009.
Kolb, C., Worsnop, D., Zahniser, M., Davidovits, P., Keyser, L., Leu, M.-T., Molina, M., Hanson, D., Ravishankara, A., and Williams, L.: Laboratory studies of atmospheric heterogeneous chemistry, in: Progress and problems in atmospheric chemistry, World Sci., 3, 771–875, 1995.
Kuang, Y., Zhao, C. S., Zhao, G., Tao, J. C., Xu, W., Ma, N., and Bian, Y. X.: A novel method for calculating ambient aerosol liquid water content based on measurements of a humidified nephelometer system, Atmos. Meas. Tech., 11, 2967–2982, https://doi.org/10.5194/amt-11-2967-2018, 2018.
Kuang, Y., Tao, J., Xu, W., Yu, Y., Zhao, G., Shen, C., Bian, Y., and Zhao, C.: Calculating ambient aerosol surface area concentrations using aerosol light scattering enhancement measurements, Atmos. Environ., 216, 116919, https://doi.org/10.1016/j.atmosenv.2019.116919, 2019.
Lakey, P. S. J., George, I. J., Whalley, L. K., Baeza-Romero, M. T., and Heard, D. E.: Measurements of the HO2 Uptake Coefficients onto Single Component Organic Aerosols, Environ. Sci. Technol., 49, 4878–4885, https://doi.org/10.1021/acs.est.5b00948, 2015.
Lakey, P. S. J., Berkemeier, T., Krapf, M., Dommen, J., Steimer, S. S., Whalley, L. K., Ingham, T., Baeza-Romero, M. T., Pöschl, U., Shiraiwa, M., Ammann, M., and Heard, D. E.: The effect of viscosity and diffusion on the HO2 uptake by sucrose and secondary organic aerosol particles, Atmos. Chem. Phys., 16, 13035–13047, https://doi.org/10.5194/acp-16-13035-2016, 2016a.
Lakey, P. S. J., George, I. J., Baeza-Romero, M. T., Whalley, L. K., and Heard, D. E.: Organics Substantially Reduce HO2 Uptake onto Aerosols Containing Transition Metal ions, J. Phys. Chem. A, 120, 1421–1430, https://doi.org/10.1021/acs.jpca.5b06316, 2016b.
Lelieveld, J. and Crutzen, P. J.: The role of clouds in tropospheric photochemistry, J. Atmos. Chem., 12, 229–267, https://doi.org/10.1007/bf00048075, 1991.
Lewis, J., Argyle, R., Bunclarck, P., Evans, D., Gonzales-Solares, E., and Markwardt, C. B.: Non-linear Least Squares Fitting in IDL with MPFIT, arXiv preprint arXiv:0902.2850, 2009.
Li, H., Wang, D., Cui, L., Gao, Y., Huo, J., Wang, X., Zhang, Z., Tan, Y., Huang, Y., Cao, J., Chow, J. C., Lee, S.-c., and Fu, Q.: Characteristics of atmospheric PM2.5 composition during the implementation of stringent pollution control measures in shanghai for the 2016 G20 summit, Sci. Total Environ., 648, 1121–1129, 10.1016/j.scitotenv.2018.08.219, 2019a.
Li, K., Jacob, D. J., Liao, H., Shen, L., Zhang, Q., and Bates, K. H.: Anthropogenic drivers of 2013–2017 trends in summer surface ozone in China, P. Natl. Acad. Sci. USA, 116, 422, https://doi.org/10.1073/pnas.1812168116, 2019b.
Lou, S., Liao, H., and Zhu, B.: Impacts of aerosols on surface-layer ozone concentrations in China through heterogeneous reactions and changes in photolysis rates, Atmos. Environ., 85, 123-138, 10.1016/j.atmosenv.2013.12.004, 2014.
Macintyre, H. L. and Evans, M. J.: Parameterisation and impact of aerosol uptake of HO2 on a global tropospheric model, Atmos. Chem. Phys., 11, 10965–10974, https://doi.org/10.5194/acp-11-10965-2011, 2011.
Magi, L., Schweitzer, F., Pallares, C., Cherif, S., Mirabel, P., and George, C.: Investigation of the uptake rate of ozone and methyl hydroperoxide by water surfaces, J. Phys. Chem. A, 101, 4943–4949, 1997.
Mao, J., Jacob, D. J., Evans, M. J., Olson, J. R., Ren, X., Brune, W. H., Clair, J. M. St., Crounse, J. D., Spencer, K. M., Beaver, M. R., Wennberg, P. O., Cubison, M. J., Jimenez, J. L., Fried, A., Weibring, P., Walega, J. G., Hall, S. R., Weinheimer, A. J., Cohen, R. C., Chen, G., Crawford, J. H., McNaughton, C., Clarke, A. D., Jaeglé, L., Fisher, J. A., Yantosca, R. M., Le Sager, P., and Carouge, C.: Chemistry of hydrogen oxide radicals (HOx) in the Arctic troposphere in spring, Atmos. Chem. Phys., 10, 5823–5838, https://doi.org/10.5194/acp-10-5823-2010, 2010.
Mao, J., Fan, S., Jacob, D. J., and Travis, K. R.: Radical loss in the atmosphere from Cu-Fe redox coupling in aerosols, Atmos. Chem. Phys., 13, 509–519, https://doi.org/10.5194/acp-13-509-2013, 2013.
Markwardt, C. B.: Non-linear least squares fitting in IDL with MPFIT, arXiv preprint arXiv:0902.2850, 2009.
Martin, R. V., Jacob, D. J., Yantosca, R. M., Chin, M., and Ginoux, P.: Global and regional decreases in tropospheric oxidants from photochemical effects of aerosols, J. Geophys. Res.-Atmos., 108, 4097, https://doi.org/10.1029/2002jd002622, 2003.
Martinez, M., Harder, H., Kovacs, T. A., Simpas, J. B., Bassis, J., Lesher, R., Brune, W. H., Frost, G. J., Williams, E. J., Stroud, C. A., Jobson, B. T., Roberts, J. M., Hall, S. R., Shetter, R. E., Wert, B., Fried, A., Alicke, B., Stutz, J., Young, V. L., White, A. B., and Zamora, R. J.: OH and HO2 concentrations, sources, and loss rates during the Southern Oxidants Study in Nashville, Tennessee, summer 1999, J. Geophys. Res.-Atmos., 108, 4617, https://doi.org/10.1029/2003jd003551, 2003.
Matthews, P. S. J., Baeza-Romero, M. T., Whalley, L. K., and Heard, D. E.: Uptake of HO2 radicals onto Arizona test dust particles using an aerosol flow tube, Atmos. Chem. Phys., 14, 7397–7408, https://doi.org/10.5194/acp-14-7397-2014, 2014.
Mikhailov, E., Vlasenko, S., Martin, S. T., Koop, T., and Pöschl, U.: Amorphous and crystalline aerosol particles interacting with water vapor: conceptual framework and experimental evidence for restructuring, phase transitions and kinetic limitations, Atmos. Chem. Phys., 9, 9491–9522, https://doi.org/10.5194/acp-9-9491-2009, 2009.
Moon, D. R., Taverna, G. S., Anduix-Canto, C., Ingham, T., Chipperfield, M. P., Seakins, P. W., Baeza-Romero, M.-T., and Heard, D. E.: Heterogeneous reaction of HO2 with airborne TiO2 particles and its implication for climate change mitigation strategies, Atmos. Chem. Phys., 18, 327–338, https://doi.org/10.5194/acp-18-327-2018, 2018.
Mozurkewich, M., Mcmurry, P. H., Gupta, A., and Calvert, J. G.: Mass Accommodation Coefficient for Ho2 Radicals on Aqueous Particles, J. Geophys. Res.-Atmos., 92, 4163–4170, 1987.
Müller, B. and Heal, M. R.: The mass accommodation coefficient of ozone on an aqueous surface, Phys. Chem. Chem. Phys., 4, 3365–3369, 2002.
Price, H. C., Mattsson, J., Zhang, Y., Bertram, A. K., Davies, J. F., Grayson, J. W., Martin, S. T., O'Sullivan, D., Reid, J. P., and Rickards, A. M.: Water diffusion in atmospherically relevant α-pinene secondary organic material, Chem. Sci., 6, 4876–4883, 2015.
Ross, H. B. and Noone, K. J.: A numerical investigation of the destruction of peroxy radical by Cu ion catalyzed-reactions of atmospheric particles, J. Atmos. Chem., 12, 121–136, https://doi.org/10.1007/bf00115775, 1991.
Sander, R.: Modeling atmospheric chemistry: Interactions between gas-phase species and liquid cloud/aerosol particles, Surv. Geophys., 20, 1–31, 1999.
Schwartz, S. E. and Freiberg, J. E.: Mass-transport limitation of the rate of reaction of gases in liquid droplets-application to oxidation of SO2 in aqueous solutions, Atmos. Environ., 15, 1129–1144, https://doi.org/10.1016/0004-6981(81)90303-6, 1981.
Schwartz, S. E.: Gas phase and aqueous phase chemistry of HO2 in liquid water clouds, J. Geophys. Res.-Atmos., 89, 1589–1598, https://doi.org/10.1029/JD089iD07p11589, 1984.
Schwartz, S. E.: Mass-transport considerations pertinent to aqueous phase reactions of gases in liquid-water clouds, in: Chemistry of multiphase atmospheric systems, Springer, 415–471, 1986.
Schwartz, S. E.: Both sides now-The chemistry of clouds, Annals of the New York Academy of Sciences, 502, 83–144, https://doi.org/10.1111/j.1749-6632.1987.tb37648.x, 1987.
Seinfeld, J. H.: ES&T books: atmospheric chemistry and physics of air pollution, Environ. Sci. Technol., 20, 863–863, 1986.
Shiraiwa, M., Pfrang, C., and Pöschl, U.: Kinetic multi-layer model of aerosol surface and bulk chemistry (KM-SUB): the influence of interfacial transport and bulk diffusion on the oxidation of oleic acid by ozone, Atmos. Chem. Phys., 10, 3673–3691, https://doi.org/10.5194/acp-10-3673-2010, 2010.
Song, S., Gao, M., Xu, W., Shao, J., Shi, G., Wang, S., Wang, Y., Sun, Y., and McElroy, M. B.: Fine-particle pH for Beijing winter haze as inferred from different thermodynamic equilibrium models, Atmos. Chem. Phys., 18, 7423–7438, https://doi.org/10.5194/acp-18-7423-2018, 2018.
Taketani, F., Kanaya, Y., and Akimoto, H.: Kinetics of heterogeneous reactions of HO2 radical at ambient concentration levels with (NH4)2SO4 and NaCl aerosol particles, J. Phys. Chem. A, 112, 2370–2377, 2008.
Taketani, F., Kanaya, Y., and Akimoto, H.: Heterogeneous loss of HO2 by KCl, synthetic sea salt, and natural seawater aerosol particles, Atmos. Environ., 43, 1660–1665, 2009.
Taketani, F. and Kanaya, Y.: Kinetics of HO2 Uptake in Levoglucosan and Polystyrene Latex Particles, J. Phys. Chem. Lett., 1, 1701–1704, https://doi.org/10.1021/jz100478s, 2010.
Taketani, F., Kanaya, Y., Pochanart, P., Liu, Y., Li, J., Okuzawa, K., Kawamura, K., Wang, Z., and Akimoto, H.: Measurement of overall uptake coefficients for HO2 radicals by aerosol particles sampled from ambient air at Mts. Tai and Mang (China), Atmos. Chem. Phys., 12, 11907–11916, https://doi.org/10.5194/acp-12-11907-2012, 2012.
Tan, Z., Hofzumahaus, A., Lu, K., Brown, S. S., Holland, F., Huey, L. G., Kiendler-Scharr, A., Li, X., Liu, X., and Ma, N.: No Evidence for a Significant Impact of Heterogeneous Chemistry on Radical Concentrations in the North China Plain in Summer 2014, Environ. Sci. Technol., 54, 5973–5979, https://doi.org/10.1021/acs.est.0c00525, 2020.
Tan, Z., Fuchs, H., Lu, K., Hofzumahaus, A., Bohn, B., Broch, S., Dong, H., Gomm, S., Häseler, R., He, L., Holland, F., Li, X., Liu, Y., Lu, S., Rohrer, F., Shao, M., Wang, B., Wang, M., Wu, Y., Zeng, L., Zhang, Y., Wahner, A., and Zhang, Y.: Radical chemistry at a rural site (Wangdu) in the North China Plain: observation and model calculations of OH, HO2 and RO2 radicals, Atmos. Chem. Phys., 17, 663–690, https://doi.org/10.5194/acp-17-663-2017, 2017.
Tang, M., Huang, X., Lu, K., Ge, M., Li, Y., Cheng, P., Zhu, T., Ding, A., Zhang, Y., Gligorovski, S., Song, W., Ding, X., Bi, X., and Wang, X.: Heterogeneous reactions of mineral dust aerosol: implications for tropospheric oxidation capacity, Atmos. Chem. Phys., 17, 11727–11777, https://doi.org/10.5194/acp-17-11727-2017, 2017.
Tao, J., Zhang, L., Cao, J., and Zhang, R.: A review of current knowledge concerning PM2.5 chemical composition, aerosol optical properties and their relationships across China, Atmos. Chem. Phys., 17, 9485–9518, https://doi.org/10.5194/acp-17-9485-2017, 2017.
Thornton, J. and Abbatt, J. P. D.: Measurements of HO2 uptake to aqueous aerosol: Mass accommodation coefficients and net reactive loss, J. Geophys. Res.-Atmos., 110, https://doi.org/10.1029/2004JD005402, 2005.
Thornton, J. A., Jaegle, L., and McNeill, V. F.: Assessing known pathways for HO2 loss in aqueous atmospheric aerosols: Regional and global impacts on tropospheric oxidants, J. Geophys. Res.-Atmos., 113, D05303, https://doi.org/10.1029/2007JD0092361, 2008.
Tie, X., Brasseur, G., Emmons, L., Horowitz, L., and Kinnison, D.: Effects of aerosols on tropospheric oxidants: A global model study, J. Geophys. Res.-Atmos., 106, 22931–22964, https://doi.org/10.1029/2001jd900206, 2001.
Utter, R. G., Burkholder, J. B., Howard, C. J., and Ravishankara, A. R.: Measurement of the mass accommodation coefficient of ozone on aqueous surfaces, J. Phys. Chem.-Us., 96, 4973–4979, https://doi.org/10.1021/j100191a045, 1992.
Whalley, L. K., Furneaux, K. L., Goddard, A., Lee, J. D., Mahajan, A., Oetjen, H., Read, K. A., Kaaden, N., Carpenter, L. J., Lewis, A. C., Plane, J. M. C., Saltzman, E. S., Wiedensohler, A., and Heard, D. E.: The chemistry of OH and HO2 radicals in the boundary layer over the tropical Atlantic Ocean, Atmos. Chem. Phys., 10, 1555–1576, https://doi.org/10.5194/acp-10-1555-2010, 2010.
Whalley, L. K., Stone, D., George, I. J., Mertes, S., van Pinxteren, D., Tilgner, A., Herrmann, H., Evans, M. J., and Heard, D. E.: The influence of clouds on radical concentrations: observations and modelling studies of HOx during the Hill Cap Cloud Thuringia (HCCT) campaign in 2010, Atmos. Chem. Phys., 15, 3289–3301, https://doi.org/10.5194/acp-15-3289-2015, 2015.
Xue, L. K., Wang, T., Gao, J., Ding, A. J., Zhou, X. H., Blake, D. R., Wang, X. F., Saunders, S. M., Fan, S. J., Zuo, H. C., Zhang, Q. Z., and Wang, W. X.: Ground-level ozone in four Chinese cities: precursors, regional transport and heterogeneous processes, Atmos. Chem. Phys., 14, 13175–13188, https://doi.org/10.5194/acp-14-13175-2014, 2014.
Yin, J., Allen, A. G., Harrison, R. M., Jennings, S. G., Wright, E., Fitzpatrick, M., Healy, T., Barry, E., Ceburnis, D., and McCusker, D.: Major component composition of urban PM10 and PM2.5 in Ireland, Atmos. Res., 78, 149–165, https://doi.org/10.1016/j.atmosres.2005.03.006, 2005.
Zou, Q., Song, H., Tang, M., and Lu, K.: Measurements of HO2 uptake coefficient on aqueous (NH4)2SO4 aerosol using aerosol flow tube with LIF system, Chinese Chem. Lett., 30, 2236–2240, https://doi.org/10.1016/j.cclet.2019.07.041, 2019.