The influence of ¹⁴CO₂ releases from regional nuclear facilities at the Heidelberg ¹⁴CO₂ sampling site (1986–2014)

Atmospheric Δ¹⁴CO₂ measurements are a well-established tool to estimate the regional fossil-fuel-derived CO₂ component. However, emissions from nuclear facilities can significantly alter the regional Δ¹⁴CO₂ level. In order to accurately quantify the signal originating from fossil CO₂ emissions, a correction term for anthropogenic ¹⁴CO₂ sources has to be determined. In this study, the HYSPLIT atmospheric dispersion model has been applied to calculate this correction for the long-term Δ¹⁴CO₂ monitoring site in Heidelberg. Wind fields with a spatial resolution of 2.5^∘ × 2.5^∘, 1^∘ × 1^∘, and 0.5^∘ × 0.5^∘ show systematic deviations, with coarser resolved wind fields leading to higher mean values for the correction. The finally applied mean Δ¹⁴CO₂ correction for the period from 1986–2014 is 2.3 ‰ with a standard deviation of 2.1 ‰ and maximum values up to 15.2 ‰. These results are based on the 0.5^∘ × 0.5^∘ wind field simulations in years when these fields were available (2009, 2011–2014), and for the other years they are based on 2.5^∘ × 2.5^∘ wind field simulations, corrected with a factor of 0.43. After operations at the Philippsburg boiling water reactor ceased in 2011, the monthly nuclear correction terms decreased to less than 2 ‰, with a mean value of 0.44 ± 0.32 ‰ from 2012 to 2014.

1 Introduction

Evaluation of the perturbation of atmospheric ¹⁴CO₂ by nuclear bomb tests in the middle of the last century has given very useful insight into carbon cycle dynamics (e.g. Levin and Hesshaimer, 2000). Today this artificial spike has almost equilibrated with the fast exchanging carbon reservoirs, and the currently observed global Δ¹⁴CO₂ trend (Δ¹⁴CO₂ being the relative deviation of the ¹⁴C∕C ratio in atmospheric carbon dioxide from standard material in per mill (‰); Stuiver and Polach, 1977) is almost exclusively due to the ongoing input of ¹⁴C-free fossil fuel CO₂ into the atmosphere (Levin et al., 2010; Graven, 2015). This long-term trend can potentially be used to estimate the global input of fossil fuel CO₂ into the atmosphere. However, the uncertainty in this estimate is still large (ca. 30 %; Levin et al., 2010) due to the uncertainty in the large ¹⁴CO₂ disequilibrium fluxes from biosphere and ocean, as well as artificial ¹⁴C sources. On the continental scale, however, atmospheric Δ¹⁴CO₂ measurements provide a powerful and the only direct and quantitative tool for estimating the regional fossil fuel component. The Δ¹⁴CO₂ measurements at a polluted station allow separating fossil-fuel-derived regional CO₂ enhancements relative to a clean reference level from those originating from biospheric fluxes if the Δ¹⁴CO₂ level at the reference site is also known (Levin et al., 2003; Turnbull et al., 2009). However, on that local to regional scale (several tens of kilometres), ¹⁴CO₂ emissions from nuclear facilities, such as boiling water reactors, can significantly contaminate atmospheric Δ¹⁴CO₂. The ¹⁴C signals from such point sources are well detectable in their immediate neighbourhood in atmospheric CO₂ (and CH₄) (e.g. Levin et al., 1992; Uchrin et al., 1998; Povinec et al., 2009) and also in plant samples (Levin et al., 1988). The ¹⁴CO₂ “plumes” from point sources normally quickly disperse at distances of some tens of kilometres (Pasquill, 1961; Turner, 1970). But if a sampling station is located in the catchment of such ¹⁴CO₂ point sources, special care is required to accurately quantify the Δ¹⁴CO₂ contamination and correct for it to estimate reliable fossil fuel CO₂ values (e.g. Levin et al., 2003).

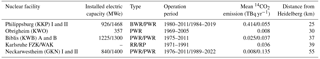
Table 1 Nuclear facilities in the surroundings of Heidelberg. Reactor type (BWR: boiling water reactor, PWR: pressurized water reactor), installed electrical power, and the average annual ¹⁴CO₂ emissions during their respective periods of operation up to 2014, as well as the distance to the Heidelberg sampling site are given. Different reactor blocks are separated by a slash. RR are research reactors and RP is the research reprocessing plant (WAK) of the Karlsruhe Research Center (FZK). After the operation period, further emissions occur during the decommissioning of the facilities (data taken from BfS 1986—2015).

Here we present results from HYSPLIT dispersion modelling (Draxler and Hess, 1998) of ¹⁴CO₂ emissions from five nuclear installations in the less than 60 km neighbourhood of our long-term Δ¹⁴CO₂ monitoring site in Heidelberg. We apply the HYSPLIT model for the period of 1986–2014 with available wind fields of 2.5^∘ × 2.5^∘, 1^∘ × 1^∘, and 0.5^∘ × 0.5^∘ resolution. Using reported ¹⁴CO₂ emission rates, these model estimates for the Heidelberg sampling site allow us to correct for the local ¹⁴CO₂ contaminations from nuclear facilities (Kuderer, 2016). Our model results, however, turn out to strongly depend on the resolution of the wind field used for the calculation. We discuss this important finding and present the currently most reliable corrections of our long-term Δ¹⁴CO₂ measurements.

2 Methods

2.1 Site description

The Heidelberg monitoring site is located on the university campus in the outskirts of Heidelberg, a medium sized city in the Upper Rhine valley in southwestern Germany (49^∘25^′ N, 8^∘41^′ E, 116 m a.s.l., see Fig. 1). From 1986–2001, CO₂ samples for Δ¹⁴C analysis have been collected from the roof of the former building of the Institut für Umweltphysik (INF 366), and from 2001 to present from the new building about 500 m to the east (INF 229). At both locations, air was sampled from about 25–30 m a.g.l. The small difference in location of the two sampling sites is not relevant when estimating the nuclear ¹⁴CO₂ contamination with HYSPLIT.

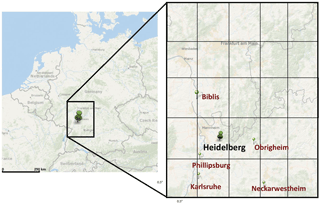
Figure 1 Map of the Heidelberg sampling site in southwest Germany. The locations of the five nearest nuclear facilities are shown in the enlargement. This enlargement corresponds to the size of the 2.5^∘ × 2.5^∘ wind field grid. The 0.5^∘ × 0.5^∘ wind field resolution is indicated by the grid in the enlargement.

Five nuclear installations with reported ¹⁴CO₂ emissions are found at distances between 25 and 55 km from the Heidelberg station. Figure 1 shows their locations; details of reactor type, installed electrical output, period of operation, distance from the Heidelberg station, and mean reported ¹⁴CO₂ emission during their operation up to 2014 are listed in Table 1. As the prevailing winds in the Upper Rhine valley are from the southwest, Philippsburg (KKP I and II) is the most important source of potential ¹⁴CO₂ contamination in Heidelberg. Philippsburg I is the only boiling water reactor (BWR) with its major ¹⁴C emissions being ¹⁴CO₂, whereas pressurized water reactors (PWR) emit ¹⁴C mainly as ¹⁴CH₄ (Kunz, 1985). All other nuclear installations except for Neckarwestheim II (GKN II) emit less than 15 % of Philippsburg I. Neckarwestheim, however, is located to the southeast of Heidelberg in the Neckar valley at a distance of 55 km, so that its relative contribution to the total ¹⁴CO₂ contamination is only less than 10 % (see Table 3).

2.2 ¹⁴CO₂ sampling and analysis

Two-week and, for limited periods, also one-week integrated large-volume samples of atmospheric CO₂ were collected from the roof of the institute's buildings by quantitative chemical absorption in basic sodium hydroxide (NaOH) solution, as described by Levin et al. (1980). Except for the first few years, samples were collected only during the night (from 19:00 to 07:00 CET, Central European Time) in order to avoid CO₂ contamination from local traffic. Moving the institute to a new building in the year 2000 required parallel CO₂ sampling at both the old and the new sampling locations on the Heidelberg University campus, in order to quantify possible differences and then allow combining the data sets from the two locations about 500 m apart. As the new building is located closer to the Heidelberg city centre, slightly lower Δ¹⁴C values (on average by 0.8 ‰) were found at the new location over the more than 1-year overlapping period from late 2000 to early 2002. The results obtained from samples collected until 2002 at INF 366 at about 25 m a.g.l. were adjusted accordingly, and are now comparable with those obtained at the current sampling location at INF 229 at about 30 m a.g.l. (for details of this comparison and correction, see Levin et al., 2008).

¹⁴CO₂ samples were processed in the Heidelberg ¹⁴C laboratory by acidification of the NaOH solution in a vacuum system. The extracted CO₂ was subsequently purified over charcoal. The ¹⁴C∕C ratio was then measured by low-level counting (Kromer and Münnich, 1992). All results are presented here as ¹³C-corrected Δ¹⁴C deviations from the international reference standard (oxalic acid) in per mill. They are corrected for decay to the date of CO₂ sampling (Stuiver and Polach, 1977). Note that Stuiver and Polach (1977) refer to this ¹⁴C notation as Δ not Δ¹⁴C; however, in order to be consistent with other atmospheric radiocarbon literature we stick to using Δ¹⁴C instead of Δ. The precision of Δ¹⁴C values was of the order of 4–5 ‰ in the 1980s and 1990s, 3–4 ‰ in the 2000s, and 2–3 ‰ thereafter.

2.3 Reported ¹⁴CO₂ emissions from nuclear facilities in the surroundings of Heidelberg

According to the German Atomic Energy Act (Strahlenschutzverordnung, 2001), emissions of radioactive substances from nuclear facilities with the exhaust air must be monitored and reported quarterly to regional and federal authorities. The Bundesamt für Strahlenschutz (BfS, German Federal Office for Radiation Protection), releases yearly reports on radioactive emissions from all German reactors and research facilities; here the ¹⁴CO₂ emissions are reported separately from other radioactive substances. These BfS reports are available for the years 1986–2014 (BfS, 1986–2015). For Philippsburg I and II higher resolution, i.e. monthly, emission data are available (Kernkraftwerk Philippsburg, personal communication, 2013); these monthly data were used in this work to estimate the ¹⁴CO₂ contamination in Heidelberg.

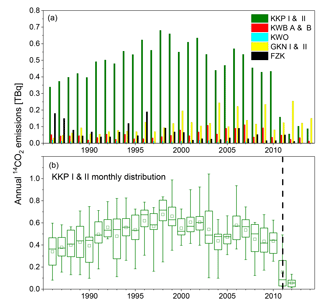
Figure 2 ¹⁴CO₂ emissions from nuclear facilities: annual mean emissions from all facilities (a) and box plots of the distribution of monthly values from Philippsburg (KKP I and II, b); the boxes include 50 % of all months of the year with the horizontal bar indicating the mean and the square indicating the median value of the year. The whiskers show the minimum and maximum monthly values of the individual years. The dashed line indicates the shutdown of Philippsburg I shortly after the Fukushima accident.

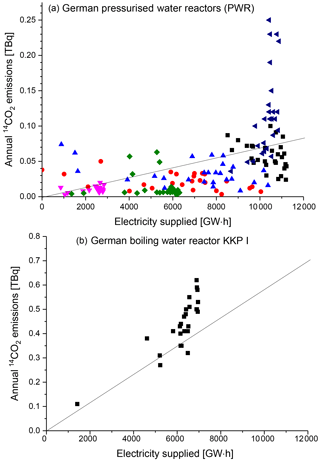
Figure 2a shows annual ¹⁴CO₂ emissions from 1986–2014 for all five facilities listed in Table 1, while Fig. 2b shows the distribution of monthly emissions from Philippsburg I and II for the years 1986–2012. Note the huge variability in monthly emissions, which can differ from month to month by more than a factor of 2. No seasonal variation nor any relation to particular maintenance activities was observed. Graven and Gruber (2011) estimated mean emission factors of 0.06 TBq ¹⁴CO₂ GWa⁻¹ for PWRs and 0.51 TBq ¹⁴CO₂ GWa⁻¹ for BWRs. However, from our emission data and corresponding power production reports, we do see large differences from these emission factors and for PWRs no correlation at all, as displayed in Fig. 3. Moreover, keeping in mind the huge month-to-month variability in ¹⁴CO₂ emissions from Philippsburg (Fig. 2b), this underlines the necessity for reliable high-resolution ¹⁴CO₂ emission data from nuclear installations if accurate corrections shall be applied to atmospheric Δ¹⁴CO₂ observations for fossil fuel CO₂ estimates.

Figure 3 Relationship between annual ¹⁴CO₂ emissions from Pressurized Water Reactors (a) and the Boiling Water Reactor Philippsburg I (b) and their annual electricity supplied. The solid lines show the specific emission factors reported by Graven and Gruber (2011).

2.4 The HYSPLIT model

The Hybrid Single-Particle Lagrangian Integrated Trajectory model (HYSPLIT) from NOAA offers a variety of services ranging from computing simple air parcel trajectories up to complex dispersion simulations (Draxler and Hess, 1998). During the simulations, virtual particles are emitted at the source location and advected to the new particle position, described by the position vector P, using the input wind velocity vector field V:

$$\begin{array}{ll}{\displaystyle }& {\displaystyle }\mathit{P}(t+\mathrm{\Delta }t)\mathit{\_}\mathrm{advection}=\mathit{P}\left(t\right)+\mathrm{0.5}\cdot \left[\mathbf{V}(\mathit{P},t)\right.\\ \text{(1)}& {\displaystyle }& {\displaystyle }\left.+\mathbf{V}\left({\mathit{P}}^{\mathbf{\prime }}(t+\mathrm{\Delta }t),t+\mathrm{\Delta }t\right)\right]\cdot \mathrm{\Delta }t.\end{array}$$

The advection equation is solved with a dynamic time step Δt, demanding that the advective displacement is smaller than the size of a grid cell (Draxler, 1999). Equation (1) is solved numerically by integrating the velocity vector over time, making use of the trapezoidal rule, i.e. averaging the velocity vectors at the initial position V(P,t) and first-guess position $\mathbf{V}\left({\mathit{P}}^{\prime }\right(t+\mathrm{\Delta }t)$, $t+\mathrm{\Delta }t)=\mathbf{V}\mathit{\{}\left(\mathit{P}\right(t)+\mathbf{V}(\mathit{P},t)\cdot \mathrm{\Delta }t),(t+\mathrm{\Delta }t)\mathit{\}}$ of the particle. To account for atmospheric dispersion, the particles are displaced stochastically (Eqs. 2a and b):

$$\begin{array}{ll}{\displaystyle }& {\displaystyle }X\mathit{\_}\text{final}(t+\mathrm{\Delta }t)=X(t+\mathrm{\Delta }t)\mathit{\_}\text{advection}\\ \text{(2a)}& {\displaystyle }& {\displaystyle }+U^{\prime }\mathit{\_}\text{dispersion}(t+\mathrm{\Delta }t)\cdot \mathrm{\Delta }t\end{array}$$

and

$$\begin{array}{ll}{\displaystyle }& {\displaystyle }Y\mathit{\_}\text{final}(t+\mathrm{\Delta }t)=Y(t+\mathrm{\Delta }t)\mathit{\_}\text{advection}\\ \text{(2b)}& {\displaystyle }& {\displaystyle }+W^{\prime }\mathit{\_}\text{dispersion}(t+\mathrm{\Delta }t)\cdot \mathrm{\Delta }t,\end{array}$$

where the turbulent velocity components U^′ and W^′ are estimated from the standard deviations σ of the horizontal or respective vertical velocity components (Fay et al., 1995). For more details, see Stein et al. (2015) and references therein.

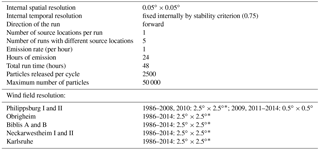
The HYSPLIT model was run here in the forward mode with an internal spatial resolution of 0.05^∘ × 0.05^∘ and an internal time step fixed by the stability ratio 0.75, i.e. the time step is chosen such that the maximal advective displacement is smaller than 0.75 times the grid size. For every nuclear facility location, a separate run has been conducted with a constant emission rate. Due to the small distance between ¹⁴C sources and the measurement station Heidelberg, simulations were limited to 48 h, where each run consisted of a 24 h period, with 2500 particles being emitted every hour, followed by 24 h of sole propagation of the particles. Thus, for each day, the simulated nuclear ¹⁴C activity included the actual emissions of this day arriving at the sampling site and the propagated emissions from the day before. This could potentially lead to loss of particles, which arrive at the measurement site more than 24–48 h after the release; but for an extended reference period, only a minor effect has been observed. Note that typical travel times from the nuclear power plants to Heidelberg are of the order of 6–12 h. The HYSPLIT model computes for every hour the particle concentration in every grid box, which gives a dilution factor f (see Eq. 3), describing how much the point source emissions are diluted over the respective grid. This dilution factor is strongly depending on the prevailing meteorological conditions. All relevant control parameters of the different runs are listed in Table 2.

Table 2 Control parameters of the HYSPLIT runs and used wind field data for ¹⁴CO₂ contamination estimates for the different nuclear facilities.

^* The HYSPLIT results obtained with 2.5^∘ × 2.5^∘ wind fields have been corrected with a factor of 0.43

Table 3 Relative average Δ¹⁴C_nuclear contribution in Heidelberg from 1986 to spring 2011 (shutdown of Philippsburg I).

2.5 Wind fields

Previous studies have shown that HYSPLIT calculations are sensitive to the meteorological input data (e.g. Cabello et al., 2008; Lin et al., 2015). Here we used three different wind velocity fields that have a horizontal resolution of 2.5^∘ × 2.5^∘, 1^∘ × 1^∘, and 0.5^∘ × 0.5^∘. The GDAS (Global Data Assimilation System) assimilates meteorological observations in numerical weather prediction models and archives the results. The one degree fields (GDAS1) are available since 2005 and the half degree fields (GDAS0p5) since 2008. GDAS1 and GDAS0p5 also differ, besides the horizontal, in the vertical resolution (Lin et al., 2015). The NCEP/NCAR (National Centers for Environmental Prediction/National Center for Atmospheric Research) reanalysis provides atmospheric analyses with a spatial resolution of 2.5^∘ × 2.5^∘, using historical data from 1948 onwards. All three wind fields are readily available at ftp://arlftp.arlhq.noaa.gov/pub/archives/, last access: 29 March 2016.

2.6 Estimation of Δ¹⁴C_nuclear

The ¹⁴C signal at the sampling site (Δ¹⁴C_nuclear) originating from ¹⁴CO₂ emissions from each nuclear facility is calculated by scaling the meteorological dilution factor f (s m⁻³) at the measurement station obtained from the HYSPLIT simulation with the time-varying emission strength Q (Bq s⁻¹) of the source. This specific ¹⁴C activity is converted (according to its definition from Stuiver and Polach, 1977) into Δ¹⁴C_nuclear in per mill according to Eq. (3)

$$\begin{array}{}\text{(3)}& {\mathrm{\Delta }}^{\mathrm{14}}{\mathrm{C}}_{\mathrm{nuclear}}=f\cdot Q\cdot X_{\mathrm{CO}{}_{\mathrm{2}}}/(M_{\mathrm{C}}\cdot V_{\mathrm{m}}\cdot a)(\cdot \mathrm{1000}\mathrm{‰}),\end{array}$$

with the molar volume at standard atmospheric temperature and pressure (STP) V_m=24.465 mole m⁻³, molar mass of carbon M_C=12 g mole⁻¹, mole fraction of CO₂, $X_{{\mathrm{CO}}_{\mathrm{2}}}$, and specific activity of the ¹⁴C standard a=0.238 Bq gC⁻¹.

Figure 4 (a) Calculated Δ¹⁴C_nuclear contributions from Philippsburg with assumed constant ¹⁴CO₂ emissions using the three wind fields with different resolution. (b) Same as (a), showing the contributions from Neckarwestheim.

3 Results

3.1 Δ¹⁴C_nuclear estimates using wind fields of different resolutions

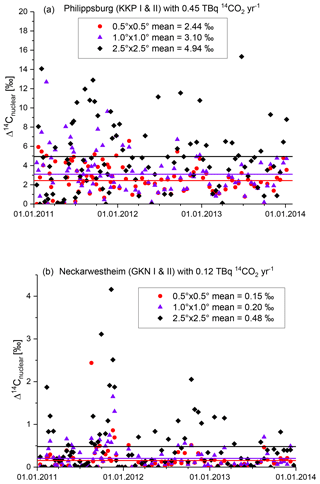
Figure 4a shows 2-weekly (i.e. sampling period) integrated HYSPLIT-estimated Δ¹⁴C_nuclear contributions in Heidelberg for 2011–2013, originating from assumed constant ¹⁴CO₂ emissions from Philippsburg of 0.45 TBq yr⁻¹ (corresponding to the long-term average emission from this facility). The different symbols distinguish the results when using the three different wind fields, i.e. with resolution of 2.5^∘ × 2.5^∘ (black diamonds), 1^∘ × 1^∘ (blue triangles), and the highest resolution of 0.5^∘ × 0.5^∘ (red circles). The 2-week integrated Δ¹⁴C_nuclear signals vary between 0 and 16 ‰ for the coarse resolution wind field, and show on average lower signals when using the higher resolved wind fields. There are, however, also situations when we obtain lower contamination signals with the coarse resolution wind field than with the higher resolved fields. The 1^∘ × 1^∘ wind field also yields, on average, slightly higher Δ¹⁴C_nuclear signals from Philippsburg than the highest resolution (0.5^∘ × 0.5^∘) wind field, but the differences between those two are often only marginal. Looking at the contributions from the Neckarwestheim reactors (GKN I and II; Fig. 4b), we also estimate the largest Δ¹⁴C_nuclear signals with the low-resolution wind field, while the highest resolution wind field yields the smallest signals. The mean ratio between the contamination signals estimated with the highest resolution wind field and those estimated with the 2.5^∘ × 2.5^∘ resolution field is 0.43. We consider the results from the higher resolution wind fields more reliable to calculate Δ¹⁴C_nuclear than those with the coarse resolution field (see discussion below). We can further see that the contributions from Neckarwestheim ¹⁴CO₂ emissions on the Heidelberg Δ¹⁴CO₂ signal are, on average, about 1 order of magnitude smaller than those from Philippsburg and, thus, with an average Δ¹⁴C_nuclear of less than 0.2 ‰, almost negligible.

3.2 Estimation of Δ¹⁴C_nuclear in Heidelberg from all five nuclear installations

Owing to its source strength and proximity to Heidelberg, Philippsburg I is the dominant contributor to the nuclear contamination at our sampling site. Therefore, and considering the high month-to-month variability in emissions (Fig. 2b), it is important to use monthly-resolved emission data to estimate the Δ¹⁴C_nuclear signals originating from this facility. The other four nuclear installations are secondary contributors permitting the use of annual average ¹⁴CO₂ emission rates in absence of higher temporally resolved emission data. For each source location, the HYSPLIT model was run for every calendar day separately covering the period 1986–2014.

For the Philippsburg reactor site, the following meteorological data has been used (Table 2): For 1986–2008 and 2010, we used the 2.5^∘ × 2.5^∘ fields, for 2009 and 2011–2014 the 0.5^∘ × 0.5^∘ fields. For the other four source locations (Obrigheim, Biblis A and B, Neckarwestheim 1 and 2, and Karlsruhe), the 2.5^∘ × 2.5^∘ wind field data have been used for the entire period 1986–2014 in order to save computing time. All coarse grid dilution factors were then corrected with a factor of 0.43 as an attempt to account for the effect of underestimating atmospheric dispersion in coarse grid simulations. This factor was obtained from the comparison made for the 3-year period 2011–2013 at Philippsburg and Neckarwestheim (Fig. 4). The average relative contributions to the total Δ¹⁴C_nuclear signal for all facilities are listed in Table 3. The largest correction terms for a 2-week sampling period originating from Philippsburg I and II were 15.2 ‰, from Neckarwestheim I and II it was 3.3 ‰, and from Biblis A and B it was 1.1 ‰. From the other two facilities, they were always smaller than 1 ‰.

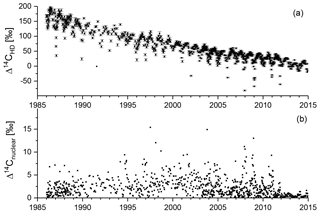
The individual uncorrected Heidelberg Δ¹⁴CO₂ data are displayed in Fig. 5a together with the individual total Δ¹⁴C_nuclear corrections Fig. 5b. In the years before the Philippsburg I shutdown, about 1 % of all corrections were above 10 ‰ and less than 2 % above 5 ‰. The mean correction was 2.3 ‰ with a standard deviation of 2.1 ‰. After the shutdown of the BWR Philippsburg I, the largest ¹⁴CO₂ source before 2011, Δ¹⁴C_nuclear decreased to less than 2 ‰, with a mean value of 0.44 ± 0.32 ‰ from 2012 to 2014. It is therefore feasible to only apply an average correction of this size to the Heidelberg measurements of all subsequent years.

Figure 5 (a) Results of Δ¹⁴CO₂ measurements in Heidelberg (uncorrected); (b) nuclear contribution from all installations in Heidelberg (note expanded Δ¹⁴C scale).

3.3 Uncertainty in estimated Δ¹⁴C_nuclear

The uncertainty in our Δ¹⁴C_nuclear estimates originates from uncertainties in emission data and uncertainties in the HYSPLIT model transport. From comparison with results based on the differently resolved wind fields (Fig. 4), we find the largest deviations between the 2.5^∘ × 2.5^∘ and the 1^∘ × 1^∘ fields while the average differences between the two finer resolved wind fields are of the order of 30 %, they can, however, be as large as a factor of 2 for individual 2-week periods. The uncertainty in the measured monthly emission data is probably less than 10–20 % and thus small if compared to the uncertainty in the model transport (although sub-monthly variability in the emissions may also contribute to the uncertainty in the Δ¹⁴C_nuclear estimates). For the contributions from nuclear installations where only annual average emission data were available to us, the uncertainty in emissions is estimated to be 30 %. As the contribution from all four installations except Philippsburg contribute on average only 12 % (Table 3), this uncertainty is small compared to the transport uncertainty in the contributions from Philippsburg. We, therefore, estimate the typical uncertainty in individual total Δ¹⁴C_nuclear signals to be less than 35 %. It is worth noting from Fig. 4a and b that the variability of Δ¹⁴C_nuclear is larger for the 2.5^∘ × 2.5^∘ wind field calculations than would be expected from the mean differences between the fine and the coarse resolution wind field simulations. Therefore, applying a simple correction factor of 0.43 on all values estimated for the years 1986–2008 and 2010 with the 2.5^∘ × 2.5^∘ wind field adds variability and uncertainty to the Δ¹⁴C_nuclear corrections, which is, however, not possible to quantify with the currently available information.

4 Discussion and conclusions

Our HYSPLIT estimates of ¹⁴CO₂ contaminations from nuclear facilities in the catchment area of Heidelberg showed large differences when using wind fields of different resolution. The calculated mean contamination was approximately twice as large when using the coarse resolution 2.5^∘ × 2.5^∘ wind field compared to the two higher resolution fields. Previous studies have shown that meteorological coarse grid reanalyses can be well suited to capture synoptic-scale dynamical processes, but biases in surface wind speeds may be introduced as reanalysis data are not well adapted to reproduce transient strong wind events occurring at the mesoscale and generating a large sub-grid scale variability (Largeron et al., 2015). These can arise in HYSPLIT trajectory calculations, which are the basis for concentration simulations, when the air mass passes through areas with complicated topography and meteorological patterns that are on a smaller scale than the data resolution (Su et al., 2015). Another and possibly more important factor is that atmospheric dispersion is included in the model by using the standard deviation of the interpolated velocity field. Linearly interpolating the coarse wind field to the internal HYSPLIT grid (here 0.05^∘ × 0.05^∘) leads to a less variable velocity field compared to initially starting with a fine grid. This generates more distinct plume shapes in coarse grid simulations (Kuderer, 2016). Therefore, using the coarse wind field may underestimate the effect of atmospheric dispersion, leading to high values when the plume directly passes the measurement point. We expect this to occur frequently in the case of the Philippsburg ¹⁴CO₂ plume, where the source lies in the main wind direction at rather short distance from the measurement point. This effect may explain the occasionally high Δ¹⁴C_nuclear values estimated for a number of sampling periods before 2009 (Fig. 5b), which are not seen in the measured uncorrected data (Fig. 5a). In the case of Neckarwestheim, this explanation does not hold. However, here we also consider the results obtained with the finest resolution wind field as more accurate. Neckarwestheim lies in the hilly Neckar valley with a complex topography, which is probably better represented by the finer resolution wind fields. Overall, we expect the HYSPLIT estimates that are based on higher resolution wind fields to provide more realistic results, in particular as the topography around Heidelberg is not flat. We therefore correct the HYSPLIT results obtained with the 2.5^∘ × 2.5^∘ wind fields for the earlier years when high-resolution wind fields (0.5^∘ × 0.5^∘) are not available. Note, however, that this first rough correction comes with additional uncertainty and variability (see above).

In an earlier study by Levin et al. (2003), Philippsburg I and II were considered as the sole sources for the nuclear contamination at the Heidelberg sampling site. A Gaussian plume model (Turner, 1970) with a constant mean dispersion factor had been applied there to calculate Δ¹⁴C_nuclear as a first approximation, but using the same monthly ¹⁴CO₂ emissions as in the present study. The mean nuclear signal estimated by Levin et al. (2003) was Δ¹⁴C_nuclear $=\mathrm{4.8}\pm \mathrm{2.0}$ ‰ ranging from 0.2 to 10 ‰ for monthly mean values. This earlier estimate of ¹⁴CO₂ contamination is approximately twice the value obtained with the HYSPLIT model and the high-resolution wind fields. Graven and Gruber (2011) used the TM3 model with a spatial resolution of 1.8^∘ × 1.8^∘ and estimated for 2005 a total Δ¹⁴C_nuclear of 2.1 (1.1–3.7) ‰ for the Heidelberg grid cell. Their estimate is in agreement with our results for that year (2.1 ± 1.6 ‰) obtained with the 2.5^∘ × 2.5^∘ resolution wind field corrected by the factor of 0.43. As in the present study, Graven and Gruber (2011) also included ¹⁴C contributions from other nuclear installations in their estimates. However, their assumed emissions from the Philippsburg I reactor were estimated with the average emission factor for BWR, which is about 20 % smaller than the measured value for 2005 used in our estimate. They also mention that their Eulerian model may have underestimated the true contamination due to its coarse resolution, which would dilute point source emissions over a large grid in an Eulerian approach.

These comparisons with earlier studies indicate that more work and higher resolution models and wind fields are needed to reduce the uncertainty in the ¹⁴CO₂ contamination estimates from nuclear installations at measurement sites where Δ¹⁴CO₂ observations shall be used to precisely determine the regional fossil fuel CO₂ component. Currently, we have to take into account a model transport uncertainty of about 1–2 ‰ in the estimated Δ¹⁴C_nuclear contamination, if the measurement site is located closer than about 30 km downwind from a nuclear facility, which has a ¹⁴CO₂ emission rate of about 0.5 TBq yr⁻¹ similar to the Philippsburg I boiling water reactor with 1 MWe power production. Other reactor types, such as the Canadian CANDU reactors may have significantly larger emission rates (Graven and Gruber, 2011; Vogel et al., 2013); the uncertainty in corresponding Δ¹⁴C_nuclear estimates in their close neighbourhood may then be considerably larger.

The limited temporal resolution of ¹⁴CO₂ emission rates from nuclear installations cause additional uncertainty in the Δ¹⁴C_nuclear estimates, as generally only annual mean emissions are reported. Graven and Gruber (2011) assume that ¹⁴CO₂ emissions are proportional to the annual power production. However, the present study on the influence from German reactors on the Heidelberg measurement site does not fully support this finding. Figure 3 does not show significant correlations between annual ¹⁴CO₂ emissions and corresponding electricity supply. Therefore, assuming emission factors as suggested by Graven and Gruber (2011) will add considerable uncertainty to the Δ¹⁴C_nuclear estimates, which may be as large as the uncertainties estimated here for the wind-field-based model transport error.

Overall, we conclude that careful investigation of potential ¹⁴CO₂ emissions in the catchment of sampling sites is required when using Δ¹⁴CO₂ observations for fossil fuel CO₂ estimates. The differences in our HYSPLIT modelling results, when based on differently resolved wind fields together with the findings from earlier studies, suggest that current Δ¹⁴C_nuclear estimates may be wrong by a factor of 2. Therefore, careful investigations with high-resolution models must be performed at all stations where ¹⁴C-based fossil fuel CO₂ measurements are conducted. Based on our simulations, the shutdown of Philippsburg I in 2011, if not accounted for in the Δ¹⁴C_nuclear correction, would have masked a fossil fuel CO₂ signal of 1 ppm, corresponding to 10 % of the average total fossil fuel CO₂ signal in Heidelberg. Therefore, we plan similar studies for the European ICOS atmospheric station network (https://www.icos-ri.eu/icos-stations-network, last access: 5 June 2018). The basis must be high-resolution ¹⁴CO₂ emissions data from nuclear facilities, which need to be made available for these investigations if contamination estimates are to be accurate.