Rate coefficients for the reactions of OH radicals with C₃–C₁₁ alkanes determined by the relative-rate technique

Rate coefficients for the reactions of OH radicals with C₃–C₁₁ alkanes were determined using the multivariate relative-rate technique. A total of 25 relative-rate coefficients at room temperature and 24 Arrhenius expressions in the temperature range of 273–323 K were obtained. Notably, a new room temperature relative-rate coefficient for 3-methylheptane that had not been previously reported was determined, and the obtained k_OH value (in units of 10⁻¹² cm³ molec.⁻¹ s⁻¹) was 7.71 ± 0.35. Interestingly, whilst results for n-alkanes agreed well with available structure–activity relationship (SAR) calculations of Kwok and Atkinson (1995), Neeb (2000), Wilson et al. (2006), Jenkin et al. (2018), and McGillen et al. (2020), the three cycloalkanes (cyclopentane, methylcyclopentane, cyclohexane) and one branched alkane (2,2,4-trimethylpentane) were found to be less reactive than predicted by the SAR approach. Conversely, the SAR estimates for 2,3-dimethylbutane were approximately 25 % lower than the experimental values, with the exception of those estimated by the Wilson group, highlighting that there may be additional factors that govern the reactivity of highly branched alkanes that are not captured by current SAR techniques. Arrhenius expressions (in units of cm³ molec.⁻¹ s⁻¹) for the reactions of various branched alkanes with OH radicals were determined for the first time: 2-methylheptane, $\left(\mathrm{1.37}\pm \mathrm{0.48}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{209}\pm \mathrm{100}\right)/T\right]$, and 3-methylheptane, $\left(\mathrm{3.54}\pm \mathrm{0.45}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{374}\pm \mathrm{49}\right)/T\right]$. The reactivity relation of saturated alkanes with OH radicals and chlorine atoms was obtained: ${\log }_{\mathrm{10}}\left(k_{(\mathrm{Cl}+\mathrm{alkanes})}\right)=\mathrm{0.569}\times {\log }_{\mathrm{10}}\left(k_{(\mathrm{OH}+\mathrm{alkanes})}\right)-\mathrm{3.111}$ (R² = 0.86). In addition, the rate coefficients for the 24 previously studied OH + alkanes reactions were consistent with existing literature values, demonstrating the reliability and efficiency of this method for the simultaneous investigation of gas-phase reaction kinetics.

1 Introduction

Volatile organic compounds (VOCs), a category of compounds found ubiquitously in the atmosphere, primarily consist of alkanes, alkenes, aromatics, and oxygenated volatile organic compounds (OVOCs) (Lewis et al., 2000; Goldstein and Galbally, 2007; Anderson et al., 2004). Research has shown that alkanes, including straight-chain, branched-chain, and cyclic alkanes within the C₃–C₁₁ range, often constitute a significant portion of VOCs (Liang et al., 2023; Dunmore et al., 2015), and they could be emitted into the atmospheric environment through natural and anthropogenic sources, e.g., C₅ alkanes emitted from gasoline usage and C₆ alkanes and higher homologous VOCs emitted as a consequence of their usage as solvents and from fuel evaporation (Atkinson, 2000; Guenther, 2002; Atkinson and Arey, 2003). In the troposphere, the alkanes are much less reactive with NO₃ and with ozone, and thus they are degraded and removed from the atmosphere via gas-phase oxidation reactions with OH radicals and chlorine atoms (Atkinson and Arey, 2003; Shi et al., 2019; Finlayson-Pitts and Pitts, 1997; Atkinson, 2000). These oxidation processes can lead to photochemical smog in the presence of NO_x and light, causing regional photochemical pollution (Fiore et al., 2005; Ling and Guo, 2014). Additionally, degradation products produced by the oxidation of alkanes can form secondary organic aerosol (SOA) through homogeneous nucleation or condensation onto existing primary particles (Sun et al., 2016).

Numerous laboratories have conducted research on the kinetics of the reaction between alkanes and OH radicals using both absolute- and relative-rate methods. The absolute-rate method (such as flash photolysis and emission flow) involves calculating the reaction kinetics parameter k_OH for organic compounds with OH radicals during the experimental process by directly measuring changes in OH radical concentration or the concentration of the target compound. Greiner (1967) measured the first kinetic data for the reaction of OH radicals with three alkanes at 300 K and 28–149 Pa in the Ar system using the flash photolysis–resonance fluorescence technique. Over the next decade, Gorse and Volman (1974), Overend et al. (1975), and Darnall et al. (1978) obtained kinetic data for the reaction of OH radicals with selected alkanes in carbon monoxide, He, and N₂ systems, respectively. Unlike the absolute-rate method, the relative-rate method relies on the recommended rate coefficient for the reaction of a reference compound with OH radicals, with the reference reaction rate coefficient needing to be similar to that of the compound under study to enhance measurement sensitivity. By monitoring the simultaneous decay of the target and reference compounds in the presence of OH radicals due to competitive response mechanisms, the rate coefficient for the reaction of OH radicals with the target compound can be determined (Atkinson and Arey, 2003; Shaw et al., 2018). From the 1980s to the early 2020s, dozens of papers about the rate coefficients of alkanes with OH radicals measured by the relative-rate method were published. For example, Shaw et al. (2018) and Phan and Li (2017) obtained rate coefficients of a series of alkanes. Anderson et al. (2004) obtained the k_OH of C₂–C₈ of several n-alkanes and cyclic alkanes using the relative technique at 296 ± 4 K. However, the majority of experiments were limited to C₂–C₆ alkanes, and, in general, more complex and multifunctional alkanes are often poorly constrained or unmeasured.

Temperature has an important influence on the reaction rate coefficients of alkanes and OH radicals. The reaction rate coefficients of several n-alkanes with OH radicals measured by Greiner (1970) increased by about 70 % in the range of 300–500 K; Perry et al. (1976) found that the rate coefficients of n-butane increased by 72 % as the temperature rose from 297 to 420 K. The rate coefficients of 10 n-alkanes and cycloalkanes obtained by Donahue et al. (1998) also increased, with the temperature varying in the range of 300–390 K. However, most reported experimental studies on the reactivity of OH radicals with a series of alkanes focus on temperatures ≥ 290 K (Greiner, 1970; Perry et al., 1976; Finlayson-Pitts et al., 1993; Donahue et al., 1998; Atkinson, 2003; Badra and Farooq, 2015), with relatively few studies at low temperatures (DeMore and Bayes, 1999; Li et al., 2006; Wilson et al., 2006; Sprengnether et al., 2009; Crawford et al., 2011). In addition, there is another alkane (e.g., 3-methylheptane) for which only two or fewer measurements of OH radical rate coefficients have been reported in the above temperature range, and it is unclear whether the rate coefficients for the reactions of OH radicals with alkanes differ in a mixed system containing oxygen compared to an inert gas system. Therefore, further investigations are required to explore the variations in the rate coefficients for different types of alkanes at various temperatures.

In this study, the rate coefficients for the reactions of 25 different C₃–C₁₁ alkanes with OH radicals were determined using the multivariate relative-rate method, including linear alkanes, cycloalkanes, and methyl alkanes. To validate the rate coefficients for the reaction between alkanes and OH radicals, multiple comparisons were made with the previous literature and values estimated from structure–activity relationships (SARs). Additionally, the rate coefficients of certain straight-chain, branched-chain, and methyl cycloalkanes were measured at 273–323 K.

2 Methods

2.1 Experiment

2.1.1 Atmospheric simulation chamber

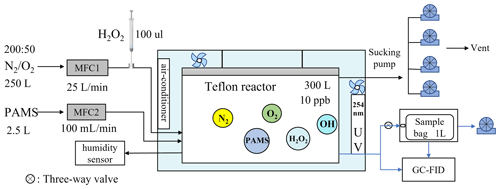
As shown in Fig. 1, the chamber experiments were performed at atmospheric pressure in a climate-controlled box with a temperature range of 263–333 K (accuracy of ± 0.1 K). A 300 L Teflon airbag was suspended in the climate-controlled box to serve as the reaction system. The box was equipped with two Teflon-coated fans for rapid chemical mixing and a 254 nm ultraviolet lamp for photolysis of hydrogen peroxide (H₂O₂) to produce OH radicals. The inner walls of the climate-controlled box were constructed with reflective steel plates to enhance ultraviolet light utilization. Bath gas (N₂ or O₂) and non-methane hydrocarbons (NMHCs) were introduced into the Teflon bag through mass flow controllers with flow rates of 25 L min⁻¹ and 100 mL min⁻¹, respectively, while excess H₂O₂ with respect to VOCs was injected through a three-way valve using a micro-syringe. Initial conditions of the different species introduced into the reactor for each experiment are outlined in Table S1 in the Supplement. By varying the presence of H₂O₂ through turning on and off the light, a series of observations were generated such as N₂+NMHCs + dark reaction, ${\mathrm{N}}_{\mathrm{2}}+\text{NMHCs}+h\mathit{\nu }$ (254 nm), and ${\mathrm{N}}_{\mathrm{2}}+\text{NMHCs}+{\mathrm{H}}_{\mathrm{2}}{\mathrm{O}}_{\mathrm{2}}+$ dark reaction to ensure no wall loss of NMHCs under dark conditions and their stability when exposed to 254 nm light.

Figure 1 A schematic of the experimental device (MFC, mass flow controller; UV, ultraviolet lamp).

2.1.2 Gas sampling and analysis

An NMHC analyzer (gas chromatography–flame ionization detection, GC-FID) with a time resolution of 1 h independently developed by the Research Center for Eco-Environmental Sciences (RCEES) was used to analyze 25 C₃–C₁₁ alkanes. The sample gas was enriched by a 60–80-mesh Carbopack B adsorption tube under a temperature condition of 183.15 K, and then the adsorption tube was rapidly heated to 453.15 K. The 25 alkanes were detected by FID at 523.15 K after programmed heating in 30 min (Liu et al., 2016).

Figure S1a in the Supplement reveals that the mixed gas diluted with N₂ underwent a 14 h reaction in a Teflon reactor without light. The k_d values ranged from 1.3 to 4.8 (the units are $\times {\mathrm{10}}^{-\mathrm{4}}$ ppbv h⁻¹), implying a negligible influence of factors such as alkane loss from reactor walls, self-consumption, or airbag leakage. Figure S1b illustrates that the peak height variation for 25 alkanes +50 µL of H₂O₂ within 15 h was less than 3 %, indicating the insignificance of dark reactions between H₂O₂ and alkanes. When the same concentration of mixed gas was irradiated for 7 h without H₂O₂, alkane concentration changes were measured, as depicted in Fig. S2. The results indicated a minimal impact of alkane photolysis on OH radical reaction rate coefficient determination.

To obtain the reaction rate coefficients of alkanes with OH radicals in 1–2 h, the alkane mixture exiting the reactor was collected in more than 10 polyvinyl fluoride (PVF) sampling bags (1.0 L) using a transparent vacuum sampling device for GC-FID. Prior to use, the empty sampling bag was flushed with high-purity nitrogen three times and placed within the vacuum sampler – a system utilizing an oil-free diaphragm air pump to create a vacuum. The initial concentrations of the alkane sample were collected before the lamp was switched on, and the following sampling process occurred every 10 min. Collected samples were subsequently analyzed using a self-developed automated injection system for the PVF bags.

2.1.3 Relative-rate technique

The rate coefficients were measured by the relative-rate method (Atkinson, 1986). Its basic principle is that the rate coefficient for the reaction of a reference compound with OH needs to be well established; then, the rate coefficient for the target compound can be determined by monitoring the simultaneous decay of the target and reference compounds in the presence of OH radicals due to the competitive response mechanism. Additionally, the reference rate coefficient, which is an important criterion for the selection of reference compounds, needs to be similar to the one under study in order to improve sensitivity. The research method of this work is based on the multivariate relative-rate method published by Shaw et al. (2018), taking the mixed system as the research object and broadening the range of compounds that can be examined.

Taking R (reference compounds) and X (target compounds) as examples, the reaction of OH radicals can be described as follows:

$$\begin{array}{}\text{(R1)}& {\displaystyle }\text{R}+\mathrm{OH}\to \text{products}\left(k_{\mathrm{R}}\right),\text{(R2)}& {\displaystyle }\text{X}+\mathrm{OH}\to \text{products}\left(k_{\mathrm{X}}\right),\text{(R3)}& {\displaystyle \frac{\mathrm{d}\left[\text{R}\right]}{\mathrm{d}t}}=k_{\mathrm{R}}\left[\mathrm{OH}\right]\left[\text{R}\right],\text{(R4)}& {\displaystyle \frac{\mathrm{d}\left[\text{X}\right]}{\mathrm{d}t}}=k_{\mathrm{X}}\left[\mathrm{OH}\right]\left[\text{X}\right],\text{(R5)}& {\displaystyle }\ln \left({\displaystyle \frac{{\left[\text{R}\right]}_{\mathrm{0}}}{{\left[\text{R}\right]}_t}}\right)=k_{\mathrm{R}}\cdot \int \left[\mathrm{OH}\right]\mathrm{d}t,\text{(R6)}& {\displaystyle }\ln \left({\displaystyle \frac{{\left[\text{X}\right]}_{\mathrm{0}}}{{\left[\text{X}\right]}_t}}\right)=k_{\mathrm{X}}\cdot \int \left[\mathrm{OH}\right]\mathrm{d}t,\text{(R7)}& {\displaystyle }\ln \left({\displaystyle \frac{{\left[\text{X}\right]}_{\mathrm{0}}}{{\left[\text{X}\right]}_t}}\right)={\displaystyle \frac{k_{\mathrm{X}}}{k_{\mathrm{R}}}}\cdot \ln \left({\displaystyle \frac{{\left[\text{R}\right]}_{\mathrm{0}}}{{\left[\text{R}\right]}_t}}\right),\end{array}$$

where [R]₀ and [X]₀ are the concentrations of reference compounds and target compounds before turning on the light and [R]_t and [X]_t are the corresponding concentrations after turning on the light for time t. k_R and k_X refer to the second-order rate coefficients for the reaction of the reference compounds and target compounds with OH radicals.

2.1.4 Choice of reference k values

It is critical to choose appropriate reference compounds in a kinetics study using the relative-rate technique. Some reported values of the rate coefficients for reactions of C₃–C₁₁ alkanes with OH radicals have been measured by different methods in different laboratories, and these measurement results may be quite different. When these rate coefficients are measured by the relative-rate technique, choosing different reference values will lead to a change in the final experimental target rate coefficients. In this work, three reference compounds (n-hexane, cyclohexane, and n-octane) were used to determine the rate coefficients for each reaction at room temperature and thereby check the consistency of kinetic results. The selection of k values for reference compounds and the literature data assessment and comparison gives priority to the available expert-evaluated rate coefficients wherever possible. Here we used the recommended evaluated data of Version 2.1.0 of the Database for the Kinetics of the Gas-Phase Atmospheric Reactions of Organic Compounds (McGillen et al., 2020, Eurochamp Data Center), which is relatively comprehensive and provides rigorously evaluated rate coefficients for many species. Among them, at 298 ± 1 K, the k values (in units of cm³ molec.⁻¹ s⁻¹) of the three reference compounds selected are evaluated rate coefficients: k_OH+n-hexane = $\mathrm{4.97}\times {\mathrm{10}}^{-\mathrm{12}}$, k_{OH+cyclohexane} = $\mathrm{6.69}\times {\mathrm{10}}^{-\mathrm{12}}$, and k_OH+n-octane = $\mathrm{8.48}\times {\mathrm{10}}^{-\mathrm{12}}$. These are derived from fitted or manually entered data from multiple sources. A detailed explanation of the impact of different temperatures is presented in Sect. 3.3.

2.1.5 Materials

The air bath gas was obtained by a mix of nitrogen (200 L) and oxygen (50 L). H₂O₂ (30 %) was provided by Sinopharm Chemical Reagent Co., Ltd. The standard gas (PAMS) is a mixed standard sample of 57 kinds of NMHCs produced by Linde SPECTRA (Alpha, NJ, USA). Sampling bags (PVF, 1 L) were provided by Dalian Delin Gas Packing Co., Ltd. The pump is the NMP 830 KNDC model produced by KNF, Germany, with a maximum air sampling rate of 23 L min⁻¹. The climate-controlled box (ZRG-1000D-C0203) was provided by Shanghai Binglin Electronic Technology Co., Ltd.

2.2 Estimation of the rate coefficient at 298 K (SAR)

In the past few decades, researchers have been devoted to finding a reasonable theoretical estimation method for the kinetic rate coefficients (Cohen, 1991). The structure–activity relationship (SAR) established and developed by Kwok and Atkinson (1995) is the most widely used estimation method of rate coefficients. Based on the relationship between the structure and the reaction activity of the compounds, this method assumes that the hydrogen extraction reaction mainly occurs in the saturated compounds and the addition reaction mainly occurs in the unsaturated compounds, which is used to estimate the gaseous rate coefficients for the reactions of most VOCs with OH radicals. An advantage of the rate coefficient estimation is that it gives a measure of the rates of attack at different sites in the molecule, which is then useful in predicting the overall temperature dependence. The rate coefficient estimated by the SAR method is in good agreement with the experimental data. In this relationship, the calculation of the rate coefficient of the hydrogen atom on the C–H bond is based on the evaluation of the rate coefficient of the −CH₃, $-{\mathrm{CH}}_{\mathrm{2}}-$, $>\mathrm{CH}-$ groups. The relationship between the group structure and the rate coefficient is as follows:

$$\begin{array}{c}{\displaystyle }k\left({\mathrm{CH}}_{\mathrm{3}}-X\right)=k_{\mathrm{prim}}^{\mathrm{0}}F\left(X\right),\\ {\displaystyle }k\left(X-{\mathrm{CH}}_{\mathrm{2}}-Y\right)=k_{\sec }^{\mathrm{0}}F\left(X\right)F\left(Y\right),\\ {\displaystyle }k\left(X-\mathrm{CH}\left(Y\right)Z\right)=k_{\mathrm{tert}}^{\mathrm{0}}F\left(X\right)F\left(Y\right)F\left(Z\right),\\ {\displaystyle }{k}_{\mathrm{tot}}=\sum \left[k\left({\mathrm{CH}}_{\mathrm{3}}-X\right)+k\left(X-{\mathrm{CH}}_{\mathrm{2}}-Y\right)+k\left(X-\mathrm{CH}\left(Y\right)Z\right)\right]\text{A},\end{array}$$

where k_tot represents the rate coefficient of each target compound. $k_{\mathrm{prim}}^{\mathrm{0}}$, $k_{\sec }^{\mathrm{0}}$, and $k_{\mathrm{tert}}^{\mathrm{0}}$ represent the rate coefficients of each −CH₃, $-{\mathrm{CH}}_{\mathrm{2}}-$, and $>\mathrm{CH}-$ atom. For standard substituent groups such as CH₃, F(−CH₃) = 1.00. X, Y, and Z represent substituent groups, and F(X), F(Y), and F(Z) refer to the activity coefficient of substituents (X, Y, Z) at different positions on carbon groups. At room temperature, $F\left(-{\mathrm{CH}}_{\mathrm{2}}-\right)$ = 1.23 and $F\left(>\mathrm{CH}-\right)$ = 1.23. Based on an extensive review of kinetic literature values for linear alkanes at room temperature, Kwok and Atkinson (1995) derived the values of $k_{\mathrm{prim}}^{\mathrm{0}}$, $k_{\sec }^{\mathrm{0}}$, and $k_{\mathrm{tert}}^{\mathrm{0}}$ at room temperature: $k_{\mathrm{prim}}^{\mathrm{0}}=\mathrm{0.136}\times {\mathrm{10}}^{-\mathrm{12}}$, $k_{\sec }^{\mathrm{0}}=\mathrm{0.934}\times {\mathrm{10}}^{-\mathrm{12}}$ and $k_{\mathrm{tert}}^{\mathrm{0}}=\mathrm{1.94}\times {\mathrm{10}}^{-\mathrm{12}}$ (the unit is cm³ molec.⁻¹ s⁻¹). After that, many researchers continued to update and modify some parameters based on the method of Kwok and Atkinson (1995) and obtained the new base rate coefficients for different positional groups, as well as developing independent methods for rate coefficient estimation. Some examples include Neeb (2000), Wilson et al. (2006), Jenkin et al. (2018), and McGillen et al. (2020).

3 Result and discussion

3.1 Results from relative-rate experiments at 298 K

The rate coefficients for the reactions involving OH radicals with C₃–C₁₁ alkanes in the mixed system were determined at 298 ± 1 K. The concentration curves of target alkanes and the reference compound (n-hexane) are plotted in Fig. 2. As shown in Fig. 2, the decay of both target and reference compounds correlated well with Reaction (R7); the intercepts of the linear fits were close to 0; and high correlation coefficients (R²) were observed for most alkanes, exceeding 0.99. Tables 1 and S2 list the k_OH values obtained for C₃–C₁₁ alkanes using the related reference compounds. The error margins (1σ) in Table 1 account for reference rate coefficient uncertainty and experimental parameter uncertainties (pressure, temperature, flow rate, reactant concentration). The results indicate strong agreement (within < 15 %) between rate coefficients for 25 C₃–C₁₁ straight-chain, branched-chain, and cyclic alkanes, using different reference compounds. For example, the k_OH values obtained for propane with n-hexane, cyclohexane, and n-octane as the reference compound were (1.38 ± 0.01) × 10⁻¹², (1.25 ± 0.03) × 10⁻¹², and (1.34 ± 0.04) × 10⁻¹² (the units are cm³ molec.⁻¹ s⁻¹), respectively (within 10 %). This suggests that reference compound variation minimally affects results, indicating reliable experimental methods and data. Notably, the rate coefficient for 3-methylheptane's reaction with OH radicals at room temperature was determined for the first time. As shown in Fig. 3, for the different bath gases, the k_OH values obtained for C₃–C₁₁ alkanes showed high agreement, errors (dY) between 0.12 and 0.41 (the units are 10⁻¹² cm³ molec.⁻¹ s⁻¹). Meanwhile, it can also be observed in the figure that most of the rate coefficients obtained are very similar to the expert-evaluated values of the database of McGillen et al. (2020). However, 2,4-dimethylpentane is an exception: the k_OH value obtained in this study is about 20 % lower than the recommended value, but it is similar to expert-evaluated value of Atkinson and Arey (2003). Additionally, it can be clearly seen in the figure that the reactivity of linear alkanes (R₁CH₂R₂) with OH radicals increases as the number of carbon atoms in the hydrocarbon molecules increases, indicating that the increase in the R terminal alkyl chain length will provide additional hydrogen extraction sites. For each additional CH₂ group from C₃–C₁₁, the reaction rate coefficient increases by about 0.95–1.81 (the unit is 10⁻¹² cm³ molec.⁻¹ s⁻¹), reflecting the fact that the main way is extract the H atom is from the secondary C–H bond. For branched alkanes, for example, 2,2-dimethylbutane and 2,3-dimethylbutane, it is obvious that the addition of the CH group increases the reaction rate coefficients with OH radicals to a great extent. For cyclic alkanes, such as cyclopentane, methylcyclopentane, cyclohexane, and methylcyclohexane, it can also be seen that the reactivity increases with the increase in the cycle size. By comparing the reaction rate coefficients of cyclopentane and cyclohexane (methylcyclopentane and methylcyclohexane), it is found that for cyclic alkanes, each CH₂ group reaction rate increases by about $\mathrm{2.37}\times {\mathrm{10}}^{-\mathrm{12}}$ cm³ molec.⁻¹ s⁻¹. It can be seen from the reaction rate coefficients of cyclopentane and methylcyclopentane (cyclohexane and methylcyclohexane) that the reaction rate coefficient increases by about $\mathrm{2.06}\times {\mathrm{10}}^{-\mathrm{12}}$ cm³ molec.⁻¹ s⁻¹ for cycloalkanes with the addition of methyl.

Table 1 Comparison of experimental results in this work with results reported in the literature at 298 ± 1 K.

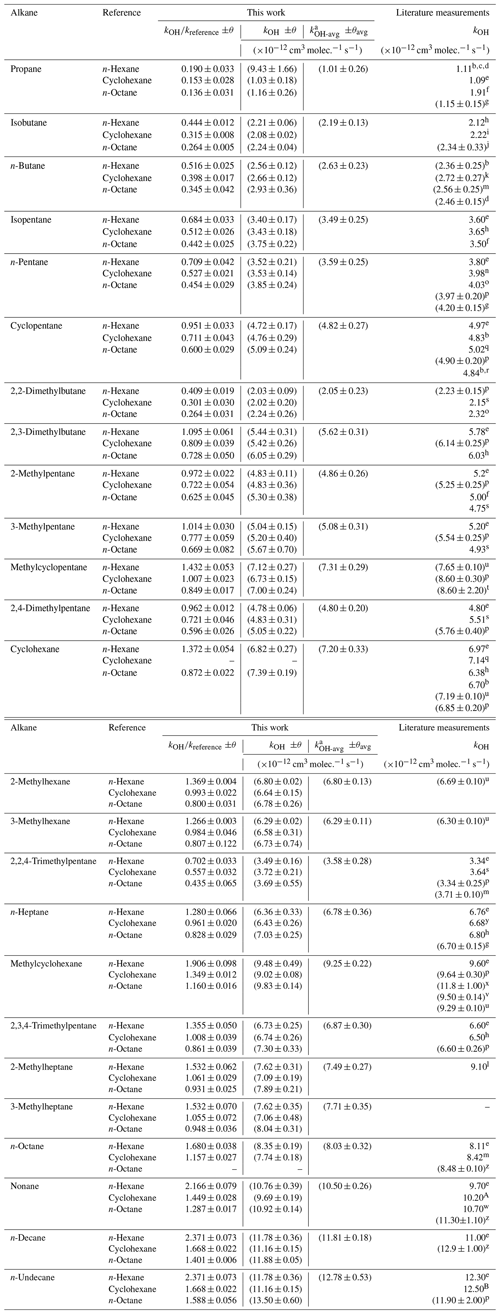
^a Weighted average $k_{\mathrm{OH}\text{-avg}}=\left(w_{\mathrm{ref}\mathrm{1}}{k}_{\mathrm{ref}\mathrm{1}}+w_{\mathrm{ref}\mathrm{2}}{k}_{\mathrm{ref}\mathrm{2}}+\mathrm{\dots }\right)/\left(w_{\mathrm{ref}\mathrm{1}}+w_{\mathrm{ref}\mathrm{2}}+\mathrm{\dots },\text{etc.}\right)$, where $w_{\mathrm{ref}\mathrm{1}}=\mathrm{1}/{\mathit{\theta }}_{\mathrm{ref}\mathrm{1}}^{\mathrm{2}}$, $w_{\mathrm{ref}\mathrm{2}}=\mathrm{1}/{\mathit{\theta }}_{\mathrm{ref}\mathrm{2}}^{\mathrm{2}}$, etc. The error is ${\mathit{\theta }}_{\mathrm{ref}}=\sqrt{\frac{\sum {\left(x_i-\stackrel{\mathrm{‾}}{x}\right)}^{\mathrm{2}}}{n}}$ and ${\mathit{\theta }}_{\mathrm{avg}}={\left(\mathrm{1}/{\mathit{\theta }}_{\mathrm{ref}\mathrm{1}}+\mathrm{1}/{\mathit{\theta }}_{\mathrm{ref}\mathrm{2}}+\mathrm{\dots },\text{etc.}\right)}^{-\mathrm{0.5}}$ . ^b DeMore and Bayes (1999). ^c Mellouki et al. (1994). ^d Talukdar et al. (1994). ^e Atkinson and Arey (2003). ^f Cox et al. (1980). ^g Morin et al. (2015). ^h Wilson et al. (2006). ⁱ Tully et al. (1986). ^j Edney et al. (1986). ^k Perry et al. (1976). ^l Shaw et al. (2020). ^m Greiner (1970). ⁿ Donahue et al. (1998). ^o Harris and Kerr (1988). ^p Calvert et al. (2015). ^q Droege and Tully (1987). ^r Singh et al. (2013). ^s Badra and Farooq (2015). ^t Anderson et al. (2004). ^u Sprengnether et al. (2009). ^v Bejan et al. (2018). ^w Atkinson et al. (1982). ^x Ballesteros et al. (2015). ^y Crawford et al. (2011). ^z Li et al. (2006). ^A Ferrari et al. (1996). ^B Sivaramakrishnan and Michael (2009).

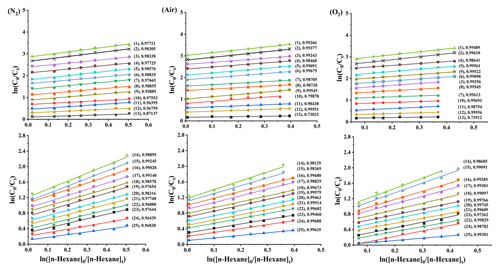
Figure 2 Typical kinetic data as acquired with the multivariate relative-rate technique at 298 K and a fixed reaction time of 70 min for the reaction of C₃–C₁₁ alkanes with the OH radicals using n-hexane as the reference compound in different bath gases (N₂, air, O₂). The numbers in parentheses correspond to each substance and are followed by the correlation coefficient R². The following data have been displaced for reasons of clarity: for N₂, (1) methylcyclopentane, (2) cyclohexane, (3) cyclopentane, (4) 2-methylpentane, (5) 2,3-dimethylbutane, (6) 2,4-dimethylpentane, (7) isopentane, (8) n-pentane, (9) 3-methylpentane, (10) isobutane, (11) n-butane, (12) 2,2-dimethylbutane, (13) propane, (14) n-undecane, (15) n-decane, (16) nonane, (17) methylcyclohexane, (18) n-octane, (19) 3-methylheptane, (20) 2-methylheptane, (21) 2,3,4-trimethylpentane, (22) n-heptane, (23) 2-methylhexane, (24) 3-methylhexane, and (25) 2,2,4-trimethylpentane were vertically displaced by 2.8, 2.6, 2.4, 2.1, 1.8, 1.6, 1.4, 1.1, 0.9, 0.7, 0.5, 0.3, 0.1, 1.2, 1.1, 1, 0.9, 0.8, 0.7, 0.6, 0.5, 0.4, 0.3, 0.25, and 0.1 units, respectively; for air, each alkane (in the above order) was vertically displaced by 3, 2.8, 2.6, 2.4, 2.1, 1.9, 1.6, 1.4, 1, 0.8, 0.6, 0.4, 0.1, 1.2, 1.1, 1, 0.9, 0.8, 0.7, 0.6, 0.5, 0.4, 0.3, 0.2, and 0.1 units, respectively; for O₂, each alkane (in the above order) was vertically displaced by 2.8, 2.6, 1.5, 2.4, 2.1, 1.7, 1, 1.3, 1.9, 0.8, 0.5, 0.3, 0.1, 1, 0.9, 0.8, 0.7, 0.6, 0.5, 0.4, 0.3, 0.1, 0.2, 0, and 0 units, respectively.

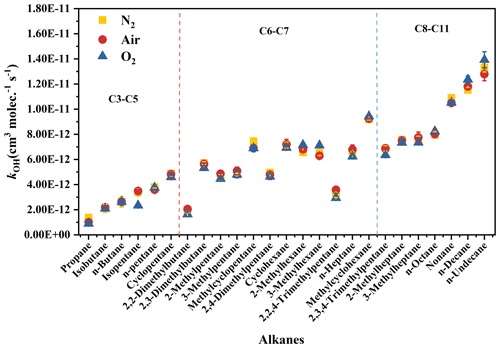
Figure 3 Comparison of rate coefficients of C₃–C₁₁ alkanes in different bath gases (N₂, air, O₂) with evaluated data at 298 ± 1 K. The error bar represents 1σ.

The obtained k_OH values for C₃–C₁₁ alkanes in this work were compared with literature-reported values (Table 1). For several n-alkanes, the average rate coefficients obtained are consistent with literature values. For example, the result of n-butane (2.63 ± 0.23) (all units in this paragraph are 10⁻¹² cm³ molec.⁻¹ s⁻¹) is highly consistent with the value (2.56 ± 0.25) obtained by Greiner (1970) and the value (2.72 ± 0.27) obtained by Perry et al. (1976), with a consistency of 3 % or better. Although slightly higher by 7 % compared to Talukdar et al. (1994) using absolute techniques (2.46 ± 0.15), when considering the errors and taking into account the experimental uncertainties, the value of this work and that of Talukdar et al. (1994) still exhibit consistency. Compared to the value obtained by DeMore and Bayes (1999) using the relative-rate method (2.36 ± 0.25) and the evaluated data (2.36) of the database of McGillen et al. (2020), our value is higher by 11 %.

n-Pentane (n-heptane). As in the n-butane case, the derived rate coefficients for n-pentane and n-heptane are in excellent agreement (4 % or better at 298 K) with previous studies (Donahue et al., 1998; Atkinson, 2003; Atkinson and Arey, 2003; Wilson et al., 2006; Crawford et al., 2011; Calvert et al., 2015; Morin et al., 2015).

n-Octane (nonane). The reaction rate coefficients of n-octane and OH radicals are in extremely good agreement with the values reported in the literature (within 5 %) (Greiner, 1970). The same applies to nonane, for which consistency with previous studies is less than 8 % (Atkinson et al., 1982; Ferrari et al., 1996; Atkinson and Arey, 2003; Li et al., 2006).

n-Decane. The obtained average k_OH for n-decane in the air system was 1.18 ± 0.02 (the unit is 10⁻¹¹ cm³ molec.⁻¹ s⁻¹). When considering experimental error, these results are consistent with the relative value (1.29 ± 0.10) obtained by Li et al. (2006) and the reviewed value (1.10) of Atkinson and Arey (2003), with a consistency of about 6 %–9 %.

n-Undecane. The obtained average k_OH for n-undecane in the air system was 1.33 ± 0.16 (the unit is 10⁻¹¹ cm³ molec.⁻¹ s⁻¹). This is about 8 % higher than the values of previous research (Atkinson and Arey, 2003; Sivaramakrishnan and Michael, 2009; Calvert et al., 2015).

For the cycloalkanes, like cyclopentane, the average rate coefficient is 4.82 ± 0.27 (the unit is 10⁻¹² cm³ molec.⁻¹ s⁻¹). The results are in excellent agreement (8 % or better) with the reviewed value (4.97) of Atkinson and Arey (2003), the relative values (4.83, 4.84) of DeMore and Bayes (1999) and Singh et al. (2013), and the absolute value (5.02) of Droege and Tully (1987). The obtained k_OH values for cyclohexane are highly consistent (3 % or better) with the absolute values (7.14 × 10⁻¹², 7.19 × 10⁻¹²) obtained by Droege and Tully (1987) and Sprengnether et al. (2009). However, these results are slightly higher than the relative value by about 5 %–16 %. For example, the relative values measured by DeMore and Bayes (1999) or Wilson et al. (2006) were 6.70 × 10⁻¹² and 6.38 × 10⁻¹², respectively. It worth noting that the k_OH value for methylcyclopentane in this work is highly consistent (within 3 % to 5 %) with the absolute data reported by Sprengnether et al. (2009). However, it is lower by approximately 15 % to 18 % compared to the relative data obtained by Andersen et al. (2003). The k_OH values for methylcyclohexane are in excellent agreement (3 % or better) with other values reported by Atkinson and Arey (2003) and Calvert et al. (2015).

Furthermore, for several less studied branched alkanes, such as 2-methylhexane, 3-methylhexane, and 2-methylheptane, there has only been one study reported so far. Sprengnether et al. (2009) conducted a study on 2-methylhexane and 3-methylhexane and obtained k_OH values at room temperature for the first time, which were 6.69 × 10⁻¹² and 6.30 × 10⁻¹² (the unit is cm³ molec.⁻¹ s⁻¹), respectively. The rate coefficients of 2-methylhexane and 3-methylhexane obtained in this work are (6.80 ± 0.13) × 10⁻¹² and (6.29 ± 0.11) × 10⁻¹², respectively, which are consistent with the values obtained by Sprengnether et al. (2009). However, the data for 2-methylheptane in this work are lower by about 17 % compared to the value reported by Shaw et al. (2018).

3.2 Comparisons to structure–activity relationships

To assess the accuracy of the estimation technique, multiple comparisons were made between the obtained reaction rate coefficients and the SAR values of different experimental groups (Fig. 4). As shown in Fig. 4, most n-alkanes fall into the shaded region, indicating a high level of agreement for k_OH rate coefficients of most n-alkanes (experimental values) with the SAR values, particularly for C₃–C₁₁ n-alkanes (within about 10 %). Although the measured values of n-butane and n-pentane were lower than the estimated values of Neeb (2000), a similar trend was observed when comparing our experimental data with the SAR values of Kwok and Atkinson (1995), Jenkin et al. (2018), and Wilson et al. (2006) (refer to Fig. 4a, c, and d), suggesting a certain level of reliability in our results.

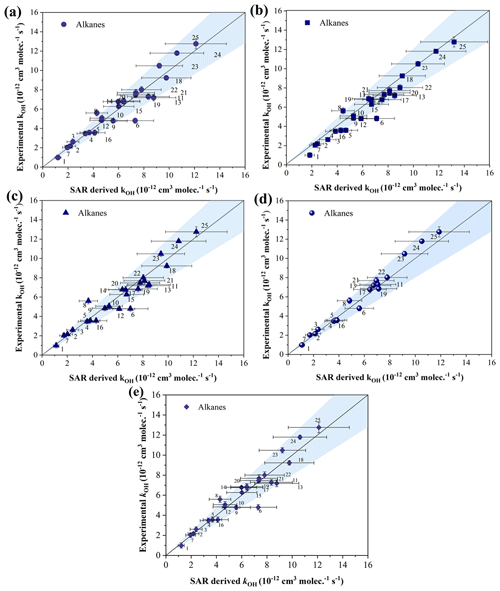
Figure 4 Measured alkanes + OH rate coefficients plotted against SAR-derived rate coefficients for all compounds (a: Kwok and Atkinson, 1995; b: Neeb, 2000; c: Jenkin et al., 2018; d: Wilson et al., 2006; e: McGillen et al., 2020). The shaded area demonstrates a 20 % uncertainty around the 1:1 black gradient line. The alkanes represented by serial numbers can be identified as follows: (1) propane, (2) isobutane, (3) n-butane, (4) isopentane, (5) n-pentane, (6) cyclopentane, (7) 2,2-dimethylbutane, (8) 2,3-dimethylbutane, (9) 2-methylpentane, (10) 3-methylpentane, (11) methylcyclopentane, (12) 2,4-dimethylpentane, (13) cyclohexane, (14) 2-methylhexane, (15) 3-methylhexane, (16) 2,2,4-trimethylpentane, (17) n-heptane, (18) methylcyclohexane, (19) 2,3,4-trimethylpentane, (20) 2-methylheptane, (21) 3-methylheptane, (22) n-octane, (23) n-nonane, (24) n-decane, and (25) n-undecane.

For branched alkanes, such as monomethyl branched alkanes (2-methylpentane, 3-methylpentane, 2-methylhexane, 3-methylhexane, 2-methylheptane, and 3-methylheptane), the obtained k_OH values all fall within the shaded area. The results indicate a relatively consistent alignment between our experimental data and the SAR-estimated data within a certain margin of error, particularly for the SAR values of Neeb (2000) and Jenkin et al. (2018) (within 8 %). Nevertheless, there seemed to be something different for polymethyl branched alkanes, like 2,3-dimethylbutane, where the experimental data were about 25 % higher than the estimated SAR values of Kwok and Atkinson (1995), and Neeb (2000) and, notably, 53% higher than that of Jenkin et al. (2018). The k_OH of this compound (at 298 K) could not be accurately estimated by Wilson et al. (2006) for unknown reasons. Furthermore, compared with the SAR values of Kwok and Atkinson (1995), the obtained data of 2,2-dimethylbutane and 2,4-dimethylpentane were relatively consistent, while compared with the estimated data of Neeb (2000), Jenkin et al. (2018), and Wilson et al. (2006), our results were higher or lower by about 18 % and 22 %. It is worth noting that the obtained k_OH value of 2,2,4-trimethylpentane was about 23 %, 16 %, and 17 % lower than the corresponding SAR values of Kwok and Atkinson (1995), Neeb (2000), and Jenkin et al. (2018), respectively. The results indicate that our understanding of the oxidation chemistry of these compounds is still limited and that further data and analysis for alkanes with this structure are needed.

For cyclopentane and cyclohexane, as examples of cyclic alkanes in general, the obtained k_OH values in this study were approximately 32 % and 15 % lower, respectively, than the SAR values of Kwok and Atkinson (1995), Fig. 4a; Neeb (2000), Fig. 4b; and Jenkin et al. (2018), Fig. 4c. On the other hand, the obtained experimental values for methylcyclopentane and methylcyclohexane were similar to SAR values of Neeb (2000) and Wilson et al. (2006) (within 5 %). However, compared with the SAR values of Kwok and Atkinson (1995) and Jenkin et al. (2018), this result is about 15 % and 8 % lower, respectively. The result suggests that the reaction activity of these cyclic alkanes estimated with SAR methods (Kwok and Atkinson, 1995; Jenkin et al., 2018) might be overestimated to varying degrees.

In addition, there are a number of SAR methods that are quite different in their estimation from those of Kwok and Atkinson (1995), Wilson et al. (2006), and Neeb (2000), for instance, the method of McGillen et al. (2020). Figure 4e shows a comparison of our measurements with the SAR estimates of McGillen et al. (2020). Similarly to the results of Kwok and Atkinson (1995), Neeb (2000), and Jenkin et al. (2018), the obtained k_OH values of cyclopentane and 2,3-dimethylbutane in this study exceed the shaded area. This further illustrates that there is still a large discrepancy between the experimental values and the SAR estimates for both substances. For cycloalkanes, the SAR estimates of McGillen et al. (2020) are still overestimated to varying degrees compared to our measurements, especially for cyclopentane, where the experimentally measured k_OH in this work is still about 34 % lower than the SAR estimate. Additionally the k_OH values for cyclohexane, methylcyclopentane, and methylcyclohexane were also lower than the estimated values by about 18 %, 12 %, and 5 %, respectively. For the branched alkanes, again the k_OH of 2,3-dimethylbutane is higher than the SAR estimate by 32 % or so. Similarly to the comparison with the Neeb (2000) and Jenkin et al. (2018) SAR estimates, the experimental measurements we obtained for 2,2,4-trimethylpentane are also lower than the McGillen et al. (2020) estimates by about 14 %. By comparing the reaction rate coefficients of cyclopentane and cyclohexane, it is found that for the cyclic alkanes of Kwok and Atkinson (1995), Neeb (2000), Jenkin et al. (2018), and McGillen et al. (2020), the increase in cycle size increases k by about 1.41 × 10⁻¹² cm³ molec.⁻¹ s⁻¹. However, for the SAR estimate of Wilson et al. (2006), the increase is about 1.12 × 10⁻¹² cm³ molec.⁻¹ s⁻¹. It is worth considering that the presence of ring strain can influence the kinetics of H-atom abstraction in cyclic alkanes, leading to an overestimation of reaction rate coefficients when using unadjusted $F\left(-\mathrm{CH}\mathrm{2}-\right)$. Hence, further data and analysis of $F\left(-\mathrm{CH}\mathrm{2}-\right)$ with these cyclic alkanes are needed.

3.3 Temperature dependence (273–323 K)

In order to investigate the relationship between temperature and the reaction rate coefficient, experiments were carried out in this study at temperatures relevant to the troposphere (273–323 K), and the Arrhenius formula was obtained for this temperature range. Also, our data were combined with the literature data (the expert-recommended data from Version 2.1.0 of the Database for the Kinetics of the Gas-Phase Atmospheric Reactions of Organic Compounds; McGillen et al., 2020) to study the kinetic temperature dependence of several alkanes in a wide temperature range. n-Hexane (Arrhenius expression: k(T) = (2.43 ± 0.52) × ${\mathrm{10}}^{-\mathrm{11}}\exp [-(\mathrm{481.2}\pm \mathrm{60})/T]$ at 240–340 K) was used as the reference compound. Since the research results at room temperature show that different bath gases have little effect on the reaction rate coefficient, only the temperature dependence of the reaction rate coefficient under the air system is considered here. Measured values for 24 C₃–C₁₀ alkanes are provided at different temperatures (273–323 K) in Table S3. The pre-exponential factor A and activation energy $E_{\mathrm{a}}/R$ obtained by linear regression and non-linear curve fits along with the values of the literature are listed in Table 2. The value of the pre-exponential factor A increases with the increase in the number of carbon atoms, which is consistent with the law of its reactivity. Additionally, Arrhenius plots were linearly fitted using these data along with literature data. The following is a detailed analysis of several components that are important or of temperature-dependence data that have been less or not studied; the Arrhenius plots are shown in Figs. 4–5, and other components are listed in the Supplement (Figs. S9–S21). In addition, typical R–R plots for some alkanes at different temperatures are also given in the Supplement (Figs. S3–S8).

Table 2 Summary of the Arrhenius expression of the reaction of OH radicals with C₃–C₁₁ alkanes in this work and other studies.

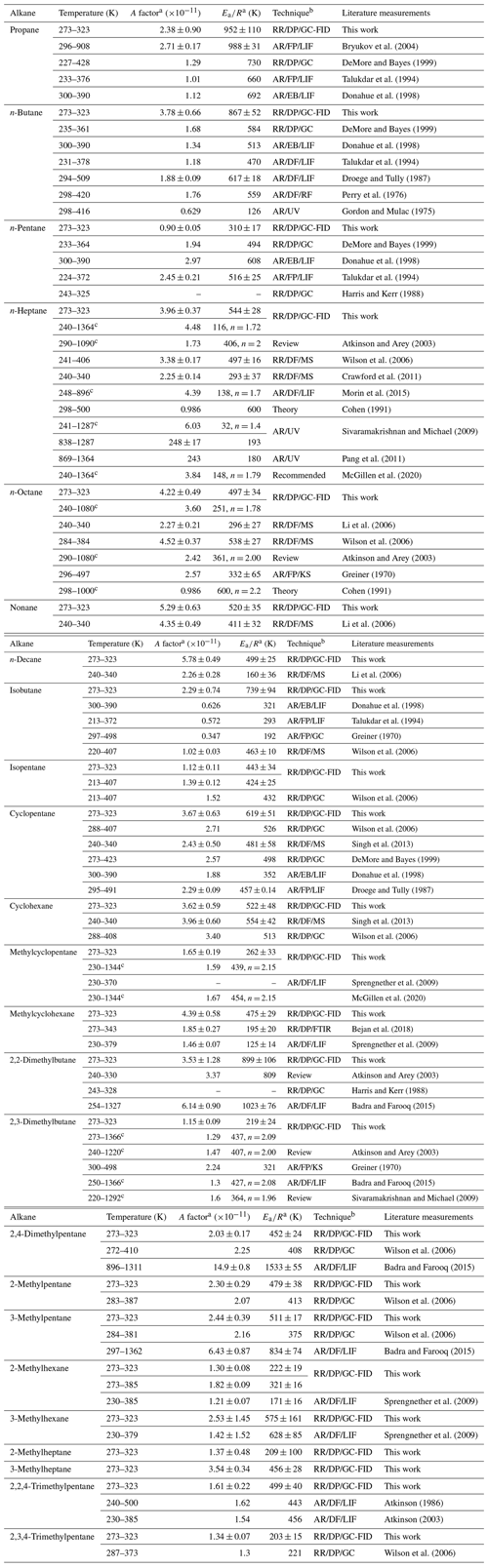
^a The error margin is 1σ. ^b RR: relative rate; AR: absolute rate; DF: discharge flow; DP: direct photolysis; FP: flash photolysis; EB: electron beam; UV: ultraviolet; GC: gas chromatography; FID: flame ionization detection; LIF: laser-induced fluorescence; FTIR: Fourier transform infrared spectrometer; RF: resonance fluorescence; MS: mass spectrometry; KS: kinetic spectroscopy. ^c The expression takes the form of k(T) = $A\times \exp (E_{\mathrm{a}}/RT)\times (T/\mathrm{300}{)}^n$.

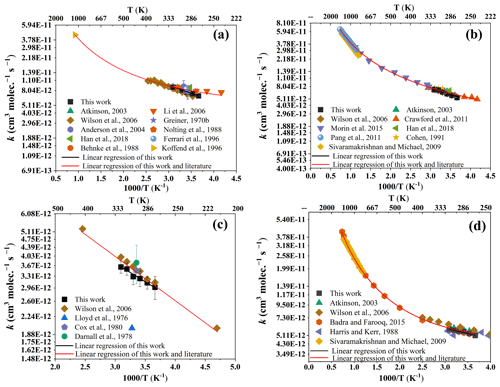
Figure 5 Arrhenius plots for the reaction of n-octane (a), n-heptane (b), isopentane (c), and 2,3-dimethylbutane (d) with OH radicals in a wide temperature range along with available literature data. The error bar represents 2σ. The expression for the non-linear curve fits takes the form of k(T) = A $\times \exp (E_{\mathrm{a}}/RT)\times (T/\mathrm{300}{)}^n$, and the expression for linear regression takes the form of k(T) = A $\times \exp (E_{\mathrm{a}}/RT)$.

3.3.1 OH + n-octane (Fig. 5a)

Figure 5a exhibits the Arrhenius plot for the reaction between n-octane and OH radicals, covering a temperature range of 240 to 1080 K. Within the experimental temperature range (273–323 K), our data align well with previous studies. The derived Arrhenius expression is as follows: $k_{n\text{-octane}}\left(T\right)=\left(\mathrm{4.22}\pm \mathrm{0.49}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{497}\pm \mathrm{34}\right)/T\right]$ (T = 273 to 323 K). The results agree well with the Arrhenius expression of (4.52 ± 0.37) $\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{538}\pm \mathrm{27}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹ reported by Wilson et al. (2006) between 284 and 384 K but contrast the expressions of $\left(\mathrm{2.27}\pm \mathrm{0.21}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{296}\pm \mathrm{27}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹ reported by Li et al. (2006) between 240 and 340 K and $\left(\mathrm{2.57}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{332}\pm \mathrm{65}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹ reported by Greiner (1970) between 296 and 497 K. Fitting our data with evaluated data (manually entered data from multiple sources), the derived Arrhenius expression at 240–1080 K is as follows: $k_{n\text{-octane}}\left(T\right)=\mathrm{3.60}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{251}/T)\times (T/\mathrm{300}{)}^{\mathrm{1.78}}$ cm³ molec.⁻¹ s⁻¹. This result is reasonably consistent with the recommended expression of ($k_{n\text{-octane}}\left(T\right)=\mathrm{2.42}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{361}/T)\times (T/\mathrm{300}{)}^{\mathrm{2.00}}$ (Atkinson and Arey, 2003). By comparison, our data are highly consistent with the data recommended by experts. The obtained Arrhenius expression more accurately represents the relationship between the reaction rate coefficient of octane and OH radicals, with temperature at 273–323 K and a wide temperature range, which has certain reference significance. Further investigations are necessary to understand the discrepancies amongst the studies mentioned here. Also, the experimental values of n-octane obtained at different temperatures are in high agreement with the SAR estimates.

3.3.2 OH + n-heptane (Fig. 5b)

The Arrhenius plot in Fig. 5b displays the reaction between n-heptane and OH radicals in the air system, covering a temperature range of 240 to 896 K. As shown in the figure, within the experimental temperature range (273–323 K), our data are highly similar to those of previous studies. The Arrhenius expression obtained is $k_{n\text{-heptane}}\left(T\right)=\left(\mathrm{3.96}\pm \mathrm{0.38}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{544}\pm \mathrm{28}\right)/T\right]$. This result agrees well with the Arrhenius expression of $\left(\mathrm{3.38}\pm \mathrm{0.17}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{497}\pm \mathrm{16}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹ reported by Wilson et al. (2006) between 241 and 406 K. By fitting our data and recommended data from multiple sources to the Arrhenius equation, the resulting Arrhenius expression at 240–1364 K is as follows: $k_{n\text{-heptane}}\left(T\right)=\mathrm{4.48}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{116}/T)\times (T/\mathrm{300}{)}^{\mathrm{1.72}}$ cm³ molec.⁻¹ s⁻¹. This result is in good agreement with the expression ($k_{n\text{-heptane}}\left(T\right)=\mathrm{4.39}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{138}/T)\times (T/\mathrm{300}{)}^{\mathrm{1.70}}$) obtained by Morin et al. (2015) at 248–896 K. Compared to the database of McGillen et al. (2020) at 240–1464 K, the activation energy ($E_{\mathrm{a}}/R$) obtained in this work is similar; however, the A factor obtained (4.48) is about 17 % higher.

3.3.3 OH + isopentane (Fig. 5c)

Presented in Fig. 5c, isopentane was extensively studied over a temperature range (213–407 K). As far as we know, at present, only Wilson et al. (2006) have reported this compound in the range of 213–407 K. Within the experimental temperature range (273–323 K), our data are consistent with those of Wilson et al. (2006) (273–323 K), especially in the low-temperature range. The Arrhenius expression obtained at 273–323 K is $k_{\mathrm{isopentane}}\left(T\right)=\left(\mathrm{1.12}\pm \mathrm{0.11}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{443}\pm \mathrm{34}\right)/T\right]$. The Arrhenius expression at 213–407 K obtained by fitting our data and those of Wilson et al. (2006) is as follows: $k_{\mathrm{isopentane}}\left(T\right)=\left(\mathrm{1.39}\pm \mathrm{0.12}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{424}\pm \mathrm{25}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹. The results are similar to the relative experimental results of Wilson et al. (2006): $\left(\mathrm{1.52}\pm \mathrm{0.21}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{432}\pm \mathrm{27}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹.

3.3.4 OH + 2,3-dimethylbutane (Fig. 5d)

Figure 5d shows the Arrhenius plot for the reaction of 2,3-dimethylbutane with OH radicals over the temperature range of 273 to 1366 K. The temperature-dependent values obtained in this study at high temperatures (313–323 K) align closely with those reported by Badra and Farooq (2015), who used the absolute-rate technique, as well as the work of Sivaramakrishnan and Michael (2009) with a three-parameter fit. However, the data obtained at 273–293 K in this work are highly consistent with the reviewed data from Atkinson and Arey (2003). In the temperature range studied (273–323 K), the Arrhenius expression obtained in this work is $k_{\text{2,3-dimethylbutane}}\left(T\right)=\left(\mathrm{1.15}\pm \mathrm{0.09}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{219}\pm \mathrm{24}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹. Linear regression applied to our data and high-temperature data in the literature (at 273–1366 K) yields the Arrhenius expression as follows: $k_{\text{2,3-dimethylbutane}}\left(T\right)=\mathrm{1.29}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{437}/T)\times (T/\mathrm{300}{)}^{\mathrm{2.09}}$ cm³ molec.⁻¹ s⁻¹. This result agrees well with the Arrhenius expression of $k_{\text{2,3-dimethylbutane}}\left(T\right)=\mathrm{1.30}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{427}/T)\times (T/\mathrm{300}{)}^{\mathrm{2.08}}$ cm³ molec.⁻¹ s⁻¹ at 250–1366 K reported by Badra and Farooq (2015).

3.3.5 OH + methylcyclopentane and OH + 2-methylhexane (Fig. 6a and b)

Figure 6a and b illustrate the Arrhenius plot for the reaction of methylcyclopentane (230–1344 K) and 2-methylhexane (273–385 K) with OH radicals. Literature data from Sprengnether et al. (2009) and Anderson et al. (2004) are available for comparison purposes. The rate coefficients of methylcyclopentane at 273–323 K in this work were obtained. Notably, for methylcyclopentane, Anderson et al. (2004) reported absolute data that are 26 % higher than the relative data obtained in this study at 298 K. However, this difference falls within the margin of error. The absolute data from Sprengnether et al. (2009) are slightly higher, ranging from 10 % to 20 %, compared to this study. Fitting our data at 273–323 K yields the Arrhenius expression of $k_{\mathrm{methylcyclopentane}}\left(T\right)=\left(\mathrm{1.65}\pm \mathrm{0.19}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{262}\pm \mathrm{33}\right)/T\right]$. Additionally, Sprengnether et al. (2009) derived an alternative Arrhenius expression to accommodate the curved behavior of the rate coefficient between 230 and 370 K, making it difficult to directly compare it with our Arrhenius expression. Besides, in order to obtain temperature-dependent relationships over a wide temperature range, the experimental data obtained at 273–323 K are fitted with multi-party literature data, especially the data from Sivaramakrishnan and Michael (2009) at high temperatures (859–1344 K), and the resulting Arrhenius expression is as follows: $k_{\mathrm{methylcyclopentane}}\left(T\right)=\mathrm{1.59}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{439}/T)\times (T/\mathrm{300}{)}^{\mathrm{2.15}}$ cm³ molec.⁻¹ s⁻¹. The result is highly consistent with the expert-evaluated Arrhenius expression of methylcyclopentane ($k_{\mathrm{methylcyclopentane}}\left(T\right)=\mathrm{1.67}\times {\mathrm{10}}^{-\mathrm{12}}\times \exp (\mathrm{454}/T)\times (T/\mathrm{300}{)}^{\mathrm{2.15}}$ cm³ molec.⁻¹ s⁻¹), indicating that the data obtained have a certain degree of reliability.

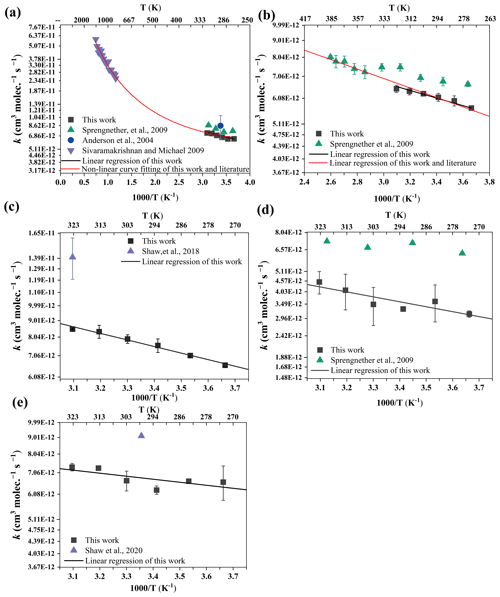
Figure 6 Arrhenius plots for the reaction of methylcyclopentane (a), 2-methylhexane (b), 3-methylheptane (c), 3-methylhexane (d), and 2-methylheptane (e) with OH radicals along with available literature data. The error bar represents 2σ. The expression for the non-linear curve fits takes the form of k(T) = A $\times \exp \left(E_{\mathrm{a}}/RT\right)\times (T/\mathrm{300}{)}^n$, and the expression for linear regression takes the form of k(T) = A $\times \exp (E_{\mathrm{a}}/RT)$.

Fitting our data at 273–323 K yields the Arrhenius expression of $k_{\text{2-methylhexane}}\left(T\right)=\left(\mathrm{1.30}\pm \mathrm{0.08}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{222}\pm \mathrm{19}\right)/T\right]$. At present, research on the temperature dependence of 2-methylhexane only includes the measured reaction rate coefficient with OH radicals of Sprengnether et al. (2009) using the absolute-rate technique at 230–385 K. The Arrhenius expression obtained by fitting our data with the data of Sprengnether et al. (2009) at 230–385 K is as follows: $k_{\text{2-methylhexane}}\left(T\right)=\left(\mathrm{1.82}\pm \mathrm{0.09}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{321}\pm \mathrm{16}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹. The expert-evaluated Arrhenius expression is $k_{\text{2-methylhexane}}\left(T\right)=\left(\mathrm{1.21}\pm \mathrm{0.07}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{171}\pm \mathrm{16}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹. Through comparison, it can be clearly seen that the two are consistent, indicating that the obtained Arrhenius expression is reliable. To the best of our knowledge, this is the first investigation of the temperature-dependent kinetics for the reaction of methylcyclopentane and 2-methylhexane with OH radicals utilizing the relative-rate technique.

3.3.6 OH + 3-methylheptane (Fig. 6c)

In Fig. 6c, the Arrhenius plot presents the reaction between 3-methylheptane and OH radicals, spanning a temperature range of 273 to 323 K. A linear regression analysis of our data yields the following Arrhenius expression: $k_{\text{3-methylheptane}}\left(T\right)=\left(\mathrm{2.72}\pm \mathrm{0.34}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{456}\pm \mathrm{28})\right./T\right]$ cm³ molec.⁻¹ s⁻¹. We believe this study to be the first investigation of the temperature-dependent kinetics for the reaction between 3-methylheptane and OH radicals. The only previous study on this reaction, reported by Shaw et al. (2020) utilizing the relative-rate method in nitrogen at 323 K, demonstrates significantly higher data (> 65 %) compared to our results. Possible explanations for this discrepancy lie in the different reference compounds used and potential sample loss during sampling in the enrichment tube in Shaw et al. (2020).

3.3.7 OH + 3-methylhexane (Fig. 6d)

We present the first temperature-dependence relative data for this compound. It can be seen from Fig. 6d that these data are significantly lower by approximately 80 % compared to the absolute data. The Arrhenius expression at 273–323 K is as follows: $k_{\text{3-methylhexane}}\left(T\right)=\left(\mathrm{2.53}\pm \mathrm{1.45}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{575}\pm \mathrm{161}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹.

3.3.8 OH + 2-methylheptane (Fig. 6e)

There have been no previous temperature-dependence data on this compound. Similarly to 3-methylhexane, these data are lower by approximately 37 % compared to Shaw et al. (2020) at room temperature. Within the range of 273–323 K, the obtained Arrhenius expression is as follows: $k_{\text{2-methylheptane}}\left(T\right)=\left(\mathrm{1.37}\pm \mathrm{0.48}\right)\times {\mathrm{10}}^{-\mathrm{11}}\exp \left[-\left(\mathrm{209}\pm \mathrm{100}\right)/T\right]$ cm³ molec.⁻¹ s⁻¹.

3.4 Correlation between the rate coefficients of the reaction of alkanes with OH radicals and chlorine atoms

Figure 7 presents a log–log correlation plot between the Cl atoms and OH radical rate coefficients with the series of C₃–C₁₁ discussed above. A very clear correlation (R² = 0.86) described by the relation of ${\log }_{\mathrm{10}}\left(k_{(\mathrm{Cl}+\mathrm{alkanes})}\right)=\mathrm{0.569}\times {\log }_{\mathrm{10}}\left(k_{(\mathrm{OH}+\mathrm{alkanes})}\right)-\mathrm{3.111}$ was obtained. Although the correlation between propane and isobutane is relatively weak, the reactivity of saturated alkanes with OH radicals and chlorine atoms is still clearly related. In addition, the log–log correlation for the series of saturated alkanes with these two oxidants presented by Calvert et al. (2011) and described by the relation of ${\log }_{\mathrm{10}}\left(k_{(\mathrm{Cl}+\mathrm{alkanes})}\right)=\mathrm{0.521}\times {\log }_{\mathrm{10}}\left(k_{(\mathrm{OH}+\mathrm{alkanes})}\right)-\mathrm{3.670}$ with R² = 0.85 is in better agreement with the log–log correlations obtained in this study for saturated alkanes. This correlation can be utilized to predict rate coefficients for unmeasured reactions, such as the reaction of 2,2,3-trimethylpentane with chlorine atoms. It is currently known that the rate coefficient for the reaction of 2,2,3-trimethylpentane with OH radicals at room temperature is 4.84 × 10⁻¹² cm³ molec.⁻¹ s⁻¹; according to the above correlation equation, it can be inferred that the rate coefficient with Cl atoms is 2.72 × 10⁻¹⁰ cm³ molec.⁻¹ s⁻¹.

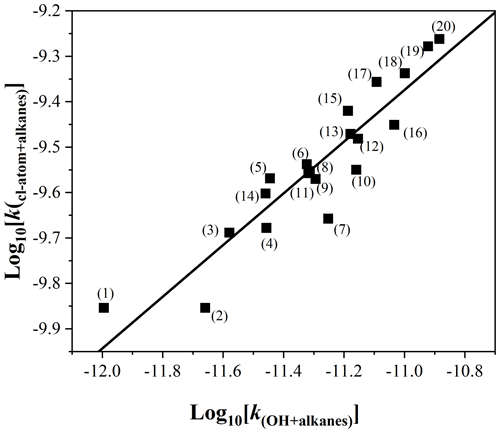
Figure 7 Log–log plot of the rate coefficients for the reaction of Cl atoms versus the reaction of OH radicals with saturated alkanes (C₃–C₁₁ alkanes discussed in the text). The solid line represents the unweighted least-squares fit to the data. The alkanes represented by serial numbers can be identified as follows: (1) propane, (2) isobutane, (3) n-butane, (4) isopentane, (5) n-pentane, (6) cyclopentane, (7) 2,3-dimethylbutane, (8) 2-methylpentane, (9) 3-methylpentane, (10) methylcyclopentane, (11) 2,4-dimethylpentane, (12) cyclohexane, (13) 2-methylhexane, (14) 2,2,4-trimethylpentane, (15) n-heptane, (16) methylcyclohexane, (17) n-octane, (18) n-nonane, (19) n-decane, and (20) n-undecane.

3.5 Atmospheric lifetime and implications

The atmospheric lifetime of alkanes in the troposphere, due to their reaction with OH radicals, can be estimated using the following formula:

$${\mathit{\tau }}_{\mathrm{alkane}}=\mathrm{1}/\left(k_{\mathrm{alkane}+\mathrm{OH}}\left[\mathrm{OH}\right]\right),$$

where τ_alkane is the atmospheric lifetime of the alkane due to OH removal, k_alkane+OH is the rate coefficient for the reaction of the alkane with OH radicals at the typical tropospheric temperature of 298 K, and [OH] is the atmospheric concentration of the hydroxyl radicals. The average tropospheric hydroxyl radical concentration has been previously reported in the literature as 1 × 10⁶ molec. cm⁻³ (Lawrence et al., 2001). Using the k_alkane+OH (298 K) values determined in the present work, the atmospheric lifetimes for 25 alkanes were estimated and are listed in Table S4. As can be seen from the table, the atmospheric lifetimes of C₃–C₁₁ alkanes reacting with OH radicals are about 1–11 d. As the carbon chain grows, the atmospheric lifetimes are reduced; especially for long-chain alkanes with carbon atoms of 8–11, the residence time in the atmosphere is only about 1 d. The alkanes are emitted into the air and degrade quickly to generate alkyl radicals, which are immediately converted into alkyl peroxy radicals by reacting with abundant O₂ in the atmosphere. Alkyl peroxyl radicals serve to convert NO to NO₂ directly, leading to the production of tropospheric ozone. Longer atmospheric residence times apply for short-chain alkanes compared to long-chain C₈–C₁₁ alkanes; for example, propane has a lifetime of about 11 d.

4 Conclusions

The use of the multivariate relative-rate method in this study allowed for the simultaneous determination of reaction rate coefficients of C₃–C₁₁ alkanes and OH radicals, which significantly improved the efficiency of determination. A total of 25 relative-rate coefficients at room temperature were obtained, including the determination of a previously unreported room temperature relative-rate coefficient for 3-methylheptane. For the n-alkanes studied, the obtained rate coefficients (k_OH) were found to be consistent with results estimated by the SAR method using parameters provided by various groups, such as Kwok and Atkinson (1995), Neeb (2000), Wilson et al. (2006), Jenkin et al. (2018), and McGillen et al. (2020). However, it is important to note that parameters other than those provided by Wilson group do not appear to reasonably estimate the rate coefficients of 2,3-dimethylbutane. Additionally, SAR estimates for several cyclic alkanes (cyclopentane, methylcyclopentane, cyclohexane) and branched alkanes (2,2,4-trimethylpentane) appear to be overestimated compared to our measurements. This raises reasonable suspicion that these methods may still lack consideration of additional factors, and a more appropriate empirical ring strain factor needs to be derived based on a broader range of experimental data from monocyclic hydrocarbons in the future. Arrhenius expressions for the reaction of 2-methylheptane and 3-methylheptane with OH radicals were obtained for the first time in the temperature range of 273–323 K, expanding the existing database. The rate coefficients do not change significantly in this temperature range, especially for 2-methylheptane, and the value of the pre-exponential factor A increases with the increase in the number of carbon atoms, which is consistent with the law of its reactivity. In addition, correlation equations for the rate coefficients of alkanes reacting with OH radicals and chlorine atoms were obtained, and the rate coefficient of 2,2,3-trimethylpentane with chlorine atoms, which had not previously been reported, was deduced. The atmospheric lifetimes of the alkanes were also obtained for further prediction of their environmental impact.