Enhanced light absorption and reduced snow albedo due to internally mixed mineral dust in grains of snow

Mineral dust is a major light-absorbing aerosol, which can significantly reduce snow albedo and accelerate snow/glacier melting via wet and dry deposition on snow. In this study, three scenarios of internal mixing of dust in ice grains were analyzed theoretically by combining asymptotic radiative transfer theory and (core–shell) Mie theory to evaluate the effects on absorption coefficient and albedo of the semi-infinite snowpack consisting of spherical snow grains. In general, snow albedo was substantially reduced at wavelengths of <1.0 µm by internal dust–snow mixing, with stronger reductions at higher dust concentrations and larger snow grain sizes. Moreover, calculations showed that a nonuniform distribution of dust in snow grains can lead to significant differences in the values of the absorption coefficient and albedo of dust-contaminated snowpack at visible wavelengths relative to a uniform dust distribution in snow grains. Finally, using comprehensive in situ measurements across the Northern Hemisphere, we found that broadband snow albedo was further reduced by 5.2 % and 9.1 % due to the effects of internal dust–snow mixing on the Tibetan Plateau and North American mountains. This was higher than the reduction in snow albedo caused by black carbon in snow over most North American and Arctic regions. Our results suggest that significant dust–snow internal mixing is important for the melting and retreat of Tibetan glaciers and North American mountain snowpack.

1 Introduction

Snow cover is one of the most reflective surfaces in the Earth system and plays a crucial role in the atmospheric solar radiation energy budget via snow–albedo feedbacks (Di Mauro et al., 2020; Flanner et al., 2011; Jacobson, 2004; Usha et al., 2020; Xie et al., 2018). Previous studies have shown that light-absorbing particles (LAPs) effectively reduce snow albedo and enhance the absorption of solar radiation after deposition. These studies were based on in situ observations and model simulations (Casey et al., 2017; Hadley and Kirchstetter, 2012; Shi et al., 2020; Warren and Wiscombe, 1980; Yasunari et al., 2011, 2015). As a result, snow contaminated with LAPs shows significant changes in morphology (Niwano et al., 2012; Rango et al., 1996), chemistry (France et al., 2012; Reay et al., 2012), hydrology (Matt et al., 2018; Qian et al., 2015; Rahimi et al., 2019), snowmelt rate (Kaspari et al., 2015; Warren, 1984), and radiative properties (Grenfell et al., 2002; Hansen and Nazarenko, 2004; Zhao et al., 2014).

Numerous studies have assessed the potential effects of LAPs, such as black carbon (BC) and mineral dust, on snow albedo by assuming that LAPs mixed outside spherical snow grains (i.e., external mixing) (Flanner et al., 2007; Kokhanovsky, 2013; Libois et al., 2013; Wang et al., 2017; Warren and Wiscombe, 1980). For example, Warren and Wiscombe (1980) calculated snow spectral albedo by solving a radiative transfer equation using Mie theory and δ-Eddington approximations, and they found that 10–100 ng g⁻¹ of BC and 1–10 µg g⁻¹ of dust in old snow decreased albedo by 1 %–7 % and 2 %–10 % at the 400 nm wavelength, respectively. Flanner et al. (2007) pointed out that the reduction in snow albedo for 1000 ng g⁻¹ BC in snow was 0.045 (0.17) with a 50 (1000) µm snow grain radius (R_ef) based on the Snow, Ice, and Aerosol Radiation (SNICAR) radiative transfer model. This model utilizes theory from Wiscombe and Warren (1980) and the two-stream radiative transfer solution from Toon et al. (1989). Wang et al. (2017) developed a Spectral Albedo Model for Dirty Snow (SAMDS) based on asymptotic radiative transfer theory, which is a function of the snow grain radius, LAP (e.g., BC, dust) mixing ratios, and mass absorption coefficients (MACs) of LAPs. Their results revealed that broadband snow albedo decreased 0.03 and 0.003 due to 200 ng g⁻¹ of BC and 2 µg g⁻¹ of dust in snow, respectively, with a R_ef of 200 µm. Additionally, recent studies found that snow nonsphericity can interact with LAP–snow mixing, which leads to weaker LAP-induced albedo reductions for nonspherical snow shapes than snow spheres (e.g., Dang et al., 2016; He et al., 2018, 2019).

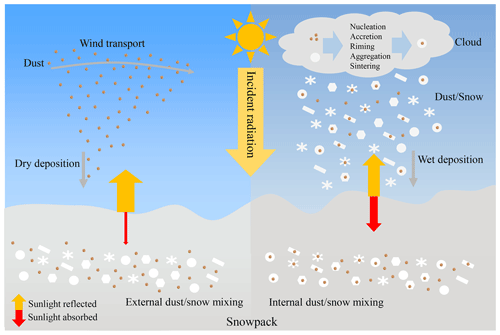
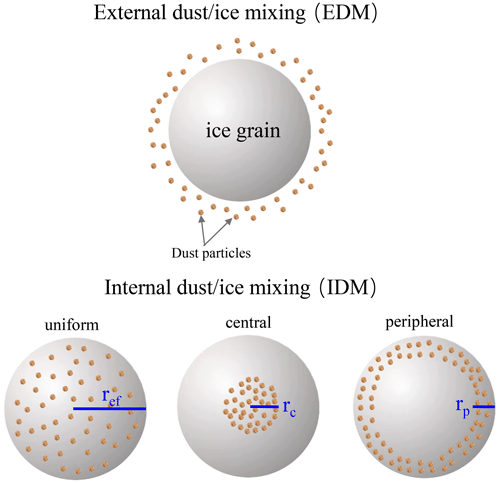
Figure 1 Schematic of dust mixing with snow grains internally. Dust tends to mix externally with snow grains through dry deposition and/or below-cloud scavenging, while dust–snow internal mixtures can be produced by nucleation, accretion, riming, aggregation, and sintering during aerosol–cloud–precipitation processes known as wet deposition. Arrows represent how the absorption (red) and reflection (yellow) of incoming sunlight changes with dust–snow mixing state.

Recently, direct snowpack observations have shown evidence for the existence of LAP–snow internal mixing (e.g., Horhold et al., 2012; Spaulding et al., 2011). LAPs tend to mix externally with snow grains through dry deposition and/or below-cloud scavenging, while internal LAP–snow mixing can be produced by nucleation, accretion, riming, aggregation, and sintering during aerosol–cloud–precipitation processes (i.e., wet deposition; Fig. 1) (Flanner et al., 2012). Furthermore, Flanner et al. (2012) found that internal BC–ice mixing (IBM) increased the absorption of snowpack by a factor of 1.8–2.1 relative to external BC–ice mixing (EBM). He et al. (2018) indicated that IBM enhanced the mean snow albedo reduction over the Tibetan Plateau by 30 %–60 % relative to EBM, based on the updated SNICAR model. Additionally, Dombrovsky and Kokhanovsky (2020) demonstrated that nonuniform BC distribution in ice grains may lead to significantly different absorption coefficients and snowpack albedo in visible (VIS) wavelengths.

Numerous studies have addressed the role of IBM in enhancing the absorption of snowpack due to its strong absorption effect relative to other LAPs (Dombrovsky and Kokhanovsky, 2020; Flanner et al., 2012; He et al., 2018; Liou et al., 2011). In contrast, few studies have considered the effects of internal dust–ice mixing (IDM) in snowpack. Liou et al. (2014) are pioneers in investigating the dust–snow internal mixing effects based on the geometric-optics surface-wave approach. Subsequently, He et al. (2019) used the same method to explicitly quantify the combined effects of dust–snow internal mixing and snow grain nonsphericity on snow optical properties. Thereafter, they developed a set of new dust–snow parameterizations for land–climate modeling applications for the first time. Actually, dust particles are generally larger than BC and act as more efficient ice nuclei, showing a better ability to influence cloud formation and precipitation (Creamean et al., 2013; Huang et al., 2014). Therefore, they are more likely to mix internally with ice grains. Furthermore, dust can also dominate light absorption and effectively decrease snow albedo because of its relatively high mass abundance (ppm) in snowpack, especially in areas with seasonal and patchy snow cover or mountainous regions (Di Mauro et al., 2015; Gabbi et al., 2015; Painter et al., 2012; Reynolds et al., 2020; Xie et al., 2018). Therefore, it is important to account for IDM when estimating the impact of dust deposition on snow albedo.

In this study, we assess the effects of external and internal mixing of dust with ice grains on the snowpack absorption coefficient and albedo using asymptotic radiative transfer theory and Mie theory. In addition, the uniformity and nonuniformity of dust particle distribution inside ice grains are considered for IDM based on the effective medium approximation, and the combined effects of dust content and snow grain radius on snow albedo are quantified. A schematic of various dust spatial distributions from this study is presented in Fig. 2. We further discuss snow albedo sensitivity to complex refractive indices and dust particle size distribution. Based on a comprehensive set of field measurements of dust concentrations, we estimate the reductions in snow albedo by dust external and internal mixing with ice grains across the Northern Hemisphere.

Figure 2 Schematic depicting various mixing scenarios of snow grains and dust particles.

2 Methods

2.1 External mixing model

For fairly pure snow, semi-infinite means about 20 cm in the VIS and 3 cm in the near-infrared (NIR) regions of snow depths (Zhou et al., 2003). For a semi-infinite snow layer under diffuse illumination conditions, albedo can be calculated using an asymptotic analysis of radiative transfer theory, which is valid in small absorptions (Kokhanovsky and Zege, 2004; Zege et al., 1991):

$$\begin{array}{}\text{(1)}& {\mathit{\alpha }}_{\mathit{\lambda }}=\text{exp}\left(-\mathrm{4}{S}_{\mathit{\lambda }}\right),\end{array}$$

where α_λ is the spectral snow albedo, λ is the wavelength, S_λ is the similarity parameter, and

$$\begin{array}{}\text{(2)}& S_{\mathit{\lambda }}=\sqrt{{\displaystyle \frac{{\mathit{\sigma }}_{\mathrm{abs}}}{\mathrm{3}{\mathit{\sigma }}_{\mathrm{ext}}(\mathrm{1}-g)}}}.\end{array}$$

In Eq. (2), σ_abs and σ_ext are the absorption and extinction coefficients, respectively, and g is the asymmetry parameter (the average cosine of the phase function of the medium).

According to Eqs. (18) and (25) in Kokhanovsky and Zege (2004), the extinction coefficients of particles can be expressed as

$$\begin{array}{}\text{(3)}& {\mathit{\sigma }}_{\mathrm{ext}}={\displaystyle \frac{\mathrm{1}}{l_{\mathrm{tr}}(\mathrm{1}-g)}}={\displaystyle \frac{\mathrm{3}{C}_{\mathrm{v}}}{\mathrm{2}{r}_{\mathrm{ef}}}},\end{array}$$

where l_tr is the photon transport path length, $C_{\mathrm{v}}={\mathit{\rho }}_{\mathrm{snow}}/{\mathit{\rho }}_{\mathrm{ice}}$ is the volumetric snow particle concentration, and the values ρ_ice=916.7 kg m⁻³ and ρ_snow=300 kg m⁻³ are used in subsequent calculations. r_ef is the effective snow grain radius, which is equal to the radius of the volume-to-surface equivalent sphere ($r_{\mathrm{ef}}=\frac{\mathrm{3}\stackrel{\mathrm{‾}}{V}}{\mathrm{4}\stackrel{\mathrm{‾}}{A}}$), where $\stackrel{\mathrm{‾}}{V}$ and $\stackrel{\mathrm{‾}}{A}$ are the average volume and cross-sectional (geometric shadow) area of snow grains, respectively.

For external dust–ice mixing (EDM) in a dust-contaminated snowpack, the total absorption coefficient (σ_abs) can be derived from the absorptions by snow (${\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{snow}}$) and dust (${\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{dust}}$):

$$\begin{array}{}\text{(4)}& {\mathit{\sigma }}_{\mathrm{abs}}={\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{snow}}+{\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{dust}}.\end{array}$$

For example, consider a hypothetical case of snow composed of monodispersed, spherical grains of ice. Although nonspherical snow grains lead to a slight increase in snow albedo, Dang et al. (2016) found that the albedo of a snowpack consisting of nonspherical snow grains can be mimicked by using smaller, spherical grains; thus, we do not consider the effect of nonspherical snow grains in this study. Therefore, we used the following equation for the absorption coefficient of snow (Dombrovsky and Baillis, 2010):

$$\begin{array}{}\text{(5)}& {\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{snow}}={\displaystyle \frac{\mathrm{0.75}{C}_{\mathrm{v}}\cdot Q_{\mathrm{abs}}^{\mathrm{ice}}}{r_{\mathrm{ef}}}},\end{array}$$

where $Q_{\mathrm{abs}}^{\mathrm{ice}}$ is the efficiency factor of absorption for a single ice grain, and the value of $Q_{\mathrm{abs}}^{\mathrm{ice}}$ can be calculated for homogeneous spherical ice grains considered in classical Mie theory.

The absorption coefficient of dust (Aoki et al., 2000; Marley et al., 2001; Warren et al., 2006) is expressed as

$$\begin{array}{}\text{(6)}& \begin{array}{rl}{\mathit{\sigma }}_{\mathrm{abs}}^{\mathrm{dust}}& ={\displaystyle \frac{Q_{\mathrm{abs}}^{\mathrm{dust}}\cdot \mathit{\pi }\cdot {\left(r_{\mathrm{ef}}^{\mathrm{dust}}\right)}^{\mathrm{2}}}{\frac{\mathrm{4}}{\mathrm{3}}\mathit{\pi }\cdot {\left(r_{\mathrm{ef}}^{\mathrm{dust}}\right)}^{\mathrm{3}}}}\cdot C_{\mathrm{dust}}={\displaystyle \frac{\mathrm{3}{Q}_{\mathrm{abs}}^{\mathrm{dust}}}{\mathrm{4}{r}_{\mathrm{ef}}^{\mathrm{dust}}}}\cdot C_{\mathrm{dust}}\\ & ={\mathrm{MAC}}_{\mathrm{abs}}^{\mathrm{dust}}\cdot {\mathit{\rho }}_{\mathrm{dust}}\cdot C_{\mathrm{dust}},\end{array}\end{array}$$

where $Q_{\mathrm{abs}}^{\mathrm{dust}}$ and ${\mathrm{MAC}}_{\mathrm{abs}}^{\mathrm{dust}}$ are the absorption efficiency and MAC of dust, respectively, that can be obtained via Mie theory, and ρ_dust and $r_{\mathrm{ef}}^{\mathrm{dust}}$ represent the density and effective dust radius, respectively. In this study, ρ_dust was assumed to be 2500 kg m⁻³ (Zender et al., 2003). We also assumed a log-normal dust size distribution with a geometric mean diameter of 0.65 µm and standard deviation of 2.0 (equivalent to an effective radius of 1.1 µm), which represents dust from large-scale transport (Formenti et al., 2011; Maring et al., 2003) that is likely smaller in size than from local soil (Kok, 2011). The effects of dust size on snow optical properties and albedo were further quantified through sensitivity simulations (see Sect. 3.4). Dust volumetric concentrations (C_dust) are expressed as

$$\begin{array}{}\text{(7)}& C_{\mathrm{dust}}={\displaystyle \frac{{\mathit{\rho }}_{\mathrm{snow}}\cdot C_{\mathrm{dust}}^{\ast }}{{\mathit{\rho }}_{\mathrm{dust}}}},\end{array}$$

where $C_{\mathrm{dust}}^{\ast }$ is the mass concentration of dust in snow (kg kg⁻¹). Thus, the spectral albedo of dust-contaminated snow for EDM can be easily calculated with Eqs. (1) to (7).

2.2 Internal mixing model

For the IDM (Fig. 2), we first determined the effective optical constants of ice containing small dust particles via an effective medium approximation (Maxwell-Garnett and Larmor, 1904). According to this approach, the complex permittivity of a composite medium in ice grains can be calculated in terms of particle polarizability by applying the Lorentz–Lorenz formula (Koledintseva et al., 2009; Markel, 2016). We used the following relationships to calculate effective complex refractive indices (RIs), $m_{\mathrm{ef}}=n_{\mathrm{ef}}-i{k}_{\mathrm{ef}}$ at known values of $m_{\mathrm{ice}}=n_{\mathrm{ice}}-i{k}_{\mathrm{ice}}$ for pure ice and $m_{\mathrm{dust}}=n_{\mathrm{dust}}-i{k}_{\mathrm{dust}}$ for dust:

$$\begin{array}{}\text{(8)}& m_{\mathrm{ef}}^{\mathrm{2}}=m_{\mathrm{ice}}^{\mathrm{2}}{\displaystyle \frac{\mathrm{2}{\mathit{\delta }}_{\mathrm{dust}}\left(m_{\mathrm{dust}}^{\mathrm{2}}-m_{\mathrm{ice}}^{\mathrm{2}}\right)+m_{\mathrm{dust}}^{\mathrm{2}}+\mathrm{2}{m}_{\mathrm{ice}}^{\mathrm{2}}}{\mathrm{2}{m}_{\mathrm{ice}}^{\mathrm{2}}+m_{\mathrm{dust}}^{\mathrm{2}}-{\mathit{\delta }}_{\mathrm{dust}}\left(m_{\mathrm{dust}}^{\mathrm{2}}-m_{\mathrm{ice}}^{\mathrm{2}}\right)}},\end{array}$$

where δ_dust is the local dust fraction volume in an ice grain. We obtained the spectral complex RIs of ice from Warren and Brandt (2008) and the spectral complex RIs of dust from Dang et al. (2015). The imaginary part of the complex RIs of ice (k_ice) and dust (k_dust) associated with absorption is shown in Fig. 3. We also evaluated the effect of dust on the imaginary part of the effective complex RIs (k_ef) assuming dust mass concentrations ($C_{\mathrm{dust}}^{\ast }$) of 1–100 ppm (or µg g⁻¹) in snow.

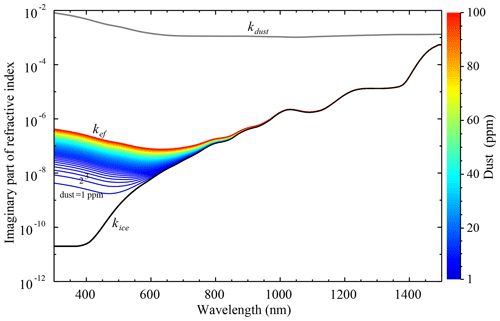
Figure 3 Imaginary part of the spectral complex refractive indices of ice (k_ice) and dust (k_dust) (Warren and Brandt, 2008; Dang et al., 2015), with the imaginary part of the effective complex refractive indices (k_ef) as a function of wavelength, at dust mass concentrations (${\mathrm{C}}_{\mathrm{dust}}^{\ast }$) of 1–100 ppm (or µg g⁻¹) in snow.

In all variations of the spatial distribution of dust particles in snow, the dust mass concentration was assumed to be constant, which means that the local dust fraction volume may differ. In an example of spherical ice grains with uniformly distributed dust, the dust fraction volume in an ice grain was determined as

$$\begin{array}{}\text{(9)}& {\mathit{\delta }}_{\mathrm{dust}}^{\mathrm{0}}={\displaystyle \frac{C_{\mathrm{dust}}^{\ast }}{C_{\mathrm{v}}}}{\displaystyle \frac{{\mathit{\rho }}_{\mathrm{ice}}}{{\mathit{\rho }}_{\mathrm{dust}}}},\end{array}$$

where the ratio of $C_{\mathrm{dust}}^{\ast }/C_{\mathrm{v}}$ is the mass fraction of dust in the ice grain.

We considered two cases of nonuniform dust distributed in a spherical ice grain with radius r_ef. (1) We assumed that the same mass of dust is uniformly distributed in the central part of the ice grain ($r_{\mathrm{ef}}^{\mathrm{dust}}<r_{\mathrm{c}}\le r_{\mathrm{ef}}$). (2) We assumed all of the dust was in the surface layer of the ice grain ($r_{\mathrm{ef}}^{\mathrm{dust}}<r_{\mathrm{p}}\le r_{\mathrm{ef}}$) (Fig. 2). In both cases the local value of δ_dust increases as

$$\begin{array}{}\text{(10)}& \begin{array}{rl}{\mathit{\delta }}_{\mathrm{dust}}& ={\mathit{\delta }}_{\mathrm{dust}}^{\mathrm{0}}/\mathit{\psi }\\ \mathit{\psi }& =\left\{\begin{array}{ll}{\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}}^{\mathrm{3}}& \text{central pollution}\\ \mathrm{1}-{\left(\mathrm{1}-\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}\right)}^{\mathrm{3}}& \text{peripheral pollution},\end{array}\right.\end{array}\end{array}$$

where $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}=r_{\mathrm{c}}/r_{\mathrm{ef}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=r_{\mathrm{p}}/r_{\mathrm{ef}}$. $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}=\mathrm{1}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=\mathrm{1}$ correspond to uniformly distributed dust when ψ=1 and ${\mathit{\delta }}_{\mathrm{dust}}={\mathit{\delta }}_{\mathrm{dust}}^{\mathrm{0}}$ and ψ<1 and ${\mathit{\delta }}_{\mathrm{dust}}>{\mathit{\delta }}_{\mathrm{dust}}^{\mathrm{0}}$ in other cases. Obviously, dust particles increase the imaginary part of the effective complex RIs in polluted ice grains (Fig. 3).

In summary, the m_ef of a spherical ice grain with uniformly and nonuniformly distributed can be calculated, according to Eqs. (8) to (10), then their corresponding absorption efficiencies can be obtained using classical Mie theory and core–shell Mie theory, respectively. The spectral snow albedo for IDM can be easily calculated using Eqs. (1) to (5).

2.3 Broadband snow albedo calculations

The spectral albedo (α_λ) is integrated over the solar spectrum (λ=300–2500 nm) and weighted by incoming solar irradiance (E_λ) to calculate broadband snow albedo (Hadley and Kirchstetter, 2012):

$$\begin{array}{}\text{(11)}& {\mathit{\alpha }}_{\mathrm{integrated}}={\displaystyle \frac{\int {\mathit{\alpha }}_{\mathit{\lambda }}{E}_{\mathit{\lambda }}\mathrm{d}\mathit{\lambda }}{\int E_{\mathit{\lambda }}\mathrm{d}\mathit{\lambda }}}.\end{array}$$

Following the study of Dang et al. (2017), the incoming solar irradiance we used is a typical surface solar spectrum at midlatitudes to high latitudes from January to March under the cloudy sky, calculated by the Santa Barbara DISORT Atmospheric Radiative Transfer (SBDART) model (Pu et al., 2019). The SBDART model is a widely used atmospheric radiation transfer model based on a collection of highly developed physical models, including the Discrete Ordinate Radiative Transfer module (Stamnes et al., 1988), low-resolution atmospheric transmission models, and Mie theory. The SBDART model can be used to compute radiative transfer at different heights and directions under both clear- and cloudy-sky conditions. Details on the SBDART model can be found in Ricchiazzi et al. (1998).

2.4 Dust concentration measurements

To estimate the effect of dust on snow albedo in real snowpack, we collected a comprehensive set of in situ dust concentration measurements during field campaigns to Inner Mongolia, China (IMC) (Wang et al., 2013); the Tibetan Plateau (TP) (Li et al., 2017, 2018, 2016, 2019; Niu et al., 2017; Qu et al., 2014; Zhang et al., 2017, 2018); Sapporo, Japan (SJ) (Kuchiki et al., 2015); the European Alps (EP) (Di Mauro et al., 2015; Lim et al., 2014); and North American mountains (NAM) (Painter et al., 2012; Reynolds et al., 2020). The field campaigns conducted in the TP can be further grouped into three regions, as in previous δ¹⁸O precipitation studies (Yao et al., 2013). These three distinct domains were associated with the Indian monsoon (Southern TP), westerlies (Northern TP), and transition (Central TP) (Yao et al., 2013). It is worth noting that we only considered regions with higher dust concentrations (>1 ppm), such that polar regions are not included in this study. Measurements of dust in the snow samples were generally obtained by weighing the filter before and after filtration using a microbalance.

3 Results

3.1 Impact on the imaginary part of the effective complex RIs

We evaluated the effect of dust on k_ef, including k_ice and k_dust associated with absorption (Fig. 3). The k_dust was in a narrow range (∼0.001–0.01) and gradually decreased with increasing wavelength in the ultraviolet (UV) and VIS regions and then remained stable in the NIR band. The k_ice varied by 8 orders of magnitude from the UV ($\sim {\mathrm{10}}^{-\mathrm{11}}$) to NIR ($\sim {\mathrm{10}}^{-\mathrm{3}}$) bands and increased with increasing wavelength, except at 1.03 µm where k_ice decreased slightly as a result of the presence of ice absorption features (Warren, 2019).

Figure 3 also shows the k_ef with dust mass concentrations ranging from 1 to 100 ppm and wavelengths of 300–1500 nm. The k_ef clearly varied depending on the wavelength and increased with dust mass concentrations. For a given dust mass concentration, k_ef decreased with wavelengths from UV to VIS and then increased from VIS to NIR. For example, the value of k_ef decreased from $\mathrm{4.26}\times {\mathrm{10}}^{-\mathrm{8}}$ at 300 nm to $\mathrm{1.36}\times {\mathrm{10}}^{-\mathrm{8}}$ at 500 nm and then rose to $\mathrm{1.73}\times {\mathrm{10}}^{-\mathrm{6}}$ at 1000 nm at a dust concentration of 10 ppm. Moreover, the wavelength of the minimum of k_ef varied from ∼500 to ∼650 nm depending on the dust mass concentrations (1 to 100 ppm). Additionally, it is worth noting that k_ef was not sensitive to dust mass concentrations in wavelengths >1000 nm, which was generally consistent with k_ice, because the difference between k_dust and k_ice is more than compensated for by the much larger difference in ice and dust concentration at those wavelengths. Conversely, k_ef showed significant differences relative to k_ice in the UV and VIS regions, with higher dust mass concentrations demonstrating larger differences. For example, k_ef was enhanced by a factor of 3, 21, and 205 at 500 nm relative to k_ice for dust mass concentrations of 1, 10, and 100 ppm, respectively.

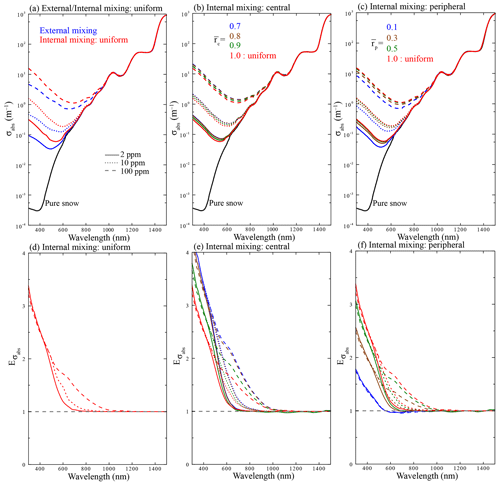
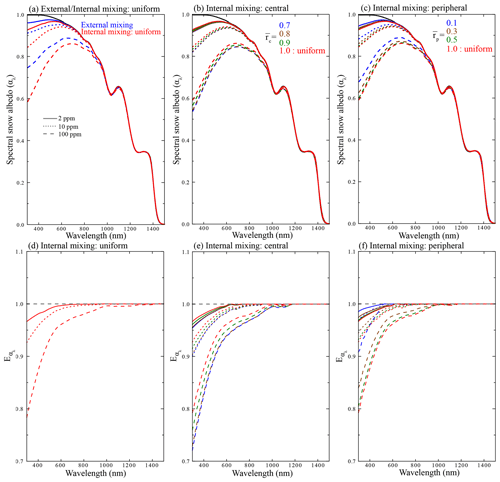
Figure 4 Snow absorption coefficients (σ_abs) for dust–snow (a) external and internal mixing (uniform), (b) internal mixing (central), and (c) internal mixing (peripheral), as a function of wavelength with different dust concentrations and $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$. The corresponding enhancement (${\mathrm{E}}_{{\mathit{\sigma }}_{\mathrm{abs}}}$) caused by (d) internal mixing (uniform), (e) internal mixing (central), and (f) internal mixing (peripheral) relative to external mixing, is shown as a function of wavelength. The snow grain radius was assumed to be 200 µm.

3.2 Impact on spectral snow absorption coefficient and albedo

Dust in snow effectively enhances the snow absorption coefficient, but its effect on the snow asymmetry factor and extinction efficiency is negligible (He et al., 2019). Therefore, we mainly focused on the effects of EDM and three cases of IDM (uniform, central, and peripheral) on the snow absorption coefficient (σ_abs). Figure 4a displays the σ_abs for EDM and IDM (uniform) as a function of wavelength at different dust concentrations. We used a snow grain radius of 200 µm (Fig. 4), which is comparable to previous observations of seasonal snow at midlatitudes to high latitudes in winter (Shi et al., 2020; Wang et al., 2017). The results showed that EDM and IDM have distinct impacts on σ_abs in UV and VIS, but small effects at wavelengths >1000 nm, which is due to the optical properties of snow being affected by LAPs in UV and VIS and primarily affected by snow itself at wavelengths >1000 nm. Additionally, σ_abs increased with increased dust mass concentrations. For instance, σ_abs increased from 0.007 m⁻¹ (pure snow) to 0.03, 0.14, and 1.37 m⁻¹ at 500 nm with 2, 10, and 100 ppm of dust with EDM, respectively. For IDM (uniform), σ_abs increased to 0.06, 0.28, and 2.80 m⁻¹ at 500 nm with 2, 10, and 100 ppm of dust, respectively, where corresponding enhancement factors of σ_abs ($E_{{\mathit{\sigma }}_{\mathrm{abs}}}$, defined as the absorption coefficient of IDM divided by EDM) were 1.84, 2.00, and 2.05. Furthermore, the σ_abs for two cases of nonuniform dust distribution in a spherical ice grain (r_ef=200 µm) depends on the wavelength, dust mass concentrations, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$, and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ (Fig. 4b and c). We note that $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ values of 1 correspond to uniformly distributed dust, and the σ_abs increases and decreases (with the decrease in $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$) for IDM (central) and IDM (peripheral), respectively. For example, σ_abs increased by 29 %, 32 %, and 33 % (500 nm) at dust mass concentrations of 2, 10, and 100 ppm, respectively, when $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ decreased from 1 to 0.7 for IDM (central). However, σ_abs decreased by 41 %, 44 %, and 44 % (500 nm) at dust mass concentrations of 2, 10, and 100 ppm, respectively, when $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$decreased from 1 to 0.1 for IDM (peripheral). This indicates that the IDM (central) further enhanced snowpack light absorption compared with the IDM (uniform), while the IDM (peripheral) reduced snowpack light absorption with a corresponding σ_abs between the value of σ_abs for IDM (uniform) and EDM. Figure 4d–f quantitatively show the spectral snow absorption coefficient enhancement for IDM ($E_{{\mathit{\sigma }}_{\mathrm{abs}}}$). The enhancement decreased sharply with increasing wavelengths and then reduced to 1.0 (i.e., no enhancement) at wavelengths longer than ∼1.0 µm because of strong dust absorption and weak snow absorption at shorter wavelengths. Obviously, $E_{{\mathit{\sigma }}_{\mathrm{abs}}}$ was affected by dust mass concentration, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$, and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$, but $E_{{\mathit{\sigma }}_{\mathrm{abs}}}$ was insensitive to dust mass concentration at wavelengths <450 nm.

Figure 5 Same as Fig. 4, but for spectral snow albedo (α_λ).

Figure 5a–c show the spectral snow albedo (α_λ) for EDM and IDM; α_λ was consistent with σ_abs, with the effects mainly present at wavelengths <1000 nm. Generally, α_λ decreased with increased dust mass concentrations in UV and VIS, and IDM was shown to further trigger the reduction of α_λ. For example, for EDM α_λ was ∼0.97, 0.95, and 0.85 (at 500 nm) for dust concentrations of 2, 10, and 100 ppm, respectively, which was higher than the values for IDM (uniform) (∼0.96, 0.93, and 0.79, respectively). Compared with IDM (uniform), the α_λ for IDM (central) decreased by 0.5 %, 1.1 %, and 3.5 % (at 500 nm) for dust concentrations of 2, 10, and 100 ppm, respectively, when $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ decreased from 1 to 0.7. α_λ for IDM (peripheral) increased by 0.8 %, 1.9 %, and 6.2 % (at 500 nm) for the same dust concentrations, when $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ decreased from 1 to 0.1. Moreover, the wavelength of the maximum α_λ value varied from ∼500 to ∼650 nm depending on the dust mass concentrations, which is consistent with changes of k_ef. Figure 5d–f show the ratio ($E_{{\mathit{\alpha }}_{\mathit{\lambda }}}$) of snow spectral albedo for IDM to EDM where we observed that the $E_{{\mathit{\alpha }}_{\mathit{\lambda }}}$ increased with increasing wavelengths and dust concentrations and then became stable at 1.0. This is because IDM can enhance the light absorption of snowpack more effectively at shorter wavelengths and higher dust concentrations (Fig. 4). Additionally, the values of $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ also have non-negligible effects on $E_{{\mathit{\alpha }}_{\mathit{\lambda }}}$, which can be decreased and increased with decreasing $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$, respectively.

Figure 6 The snow absorption coefficient (σ_abs) at the 500 nm wavelength as a function of $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ for (a) internal mixing (central) and (b) internal mixing (peripheral) with different snow grain radii and dust mass concentrations. Panels (c) and (d) are the same as (a) and (b), but for snow albedo at the 500 nm wavelength.

We found that the optical properties of an ice grain containing uniformly distributed dust in its center, or concentric surface layer, can be affected by $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ or $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$. To better understand this effect, Fig. 6a–b display the σ_abs at 500 nm as a function of $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ with different dust concentrations and r_ef. This demonstrates r_ef has negligible effects on σ_abs due to the geometric optical limits at r_ef≈50 µm, which shows the universal (independent of r_ef) monotonic dependence of σ_abs on $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ for ice grains with r_ef≥50 µm (Velesco et al., 1997). As a result, the spectral absorption coefficient of snow containing polydispersed ice grains can be obtained using our results for a monodispersed model. Interestingly, σ_abs did not depend on $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ when $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$ and decreased almost linearly at higher $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ values (Fig. 6a); this phenomenon can be explained by geometric optical effects (Mackowski et al., 1990). However, σ_abs was significantly affected by the dust mass concentration; for example, σ_abs at 500 nm was decreased by 28 %, 32 %, and 32 % from its maximum value (0.08, 0.38, and 3.71 m⁻¹) for dust concentrations of 2, 10, and 100 ppm, respectively, when $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ increased from <0.75 to 1.0. The monotonic increase in σ_abs with the relative thickness of the polluted ice grain surface layer (i.e., $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$) was also noteworthy (Fig. 6b). The core–shell Mie theory calculations for ice grains with a thin surface layer ($\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=\mathrm{0.01}$) gave almost the same σ_abs as that obtained for the EDM. As a result, the σ_abs increased rapidly with $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}<\mathrm{0.4}$ and then increased more slowly until $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=\mathrm{1}$, which corresponds to IDM (uniform).

The α_λ at 500 nm as a function of $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ and $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ with different dust concentrations and r_ef is illustrated in Fig. 6c and d. In general, α_λ at 500 nm decreased with increasing dust mass concentration and r_ef; the effect of grain radius can be explained by the fact that the snow extinction coefficient is inversely proportional to r_ef, so that for a given amount of dust, the single-scattering albedo of the snow–dust mixture is smaller for large snow grains (Gardner and Sharp, 2010). For a given dust mass concentration and r_ef, the α_λ at 500 nm increased from its minimum value with $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$ to the maximum value with $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}=\mathrm{1}$, corresponding to the findings of IDM (uniform). For example, at dust concentrations of 2, 10, and 100 ppm, and a fixed r_ef of 100 µm, the α_λ at 500 nm increased by 0.1 %, 0.7 %, and 2.6 %, respectively, when $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ increased from <0.75 to 1. When the r_ef was fixed at 500 µm, the α_λ at 500 nm increased by 0.8 %, 1.9 %, and 6.2 % at dust concentrations of 2, 10, and 100 ppm, respectively. Conversely, the α_λ at 500 nm decreased from its maximum value when $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=\mathrm{0.01}$ (similar to EDM) to the minimum value with $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}=\mathrm{1}$, corresponding to the case of IDM (uniform). For example, the α_λ at 500 nm decreased by 0.6 %, 1.4 %, and 4.7 % when $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ increased from 0.01 to 1 for dust concentrations of 2, 10, and 100 ppm, respectively, and a fixed r_ef of 100 µm, whereas for a r_ef of 500 µm, α_λ decreased by 1.4 % (2 ppm dust), 3.3 % (10 ppm), and 10.1 % (100 ppm). These results indicate that dust mass concentrations and r_ef can amplify the influence of $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}$ or $\stackrel{\mathrm{‾}}{r_{\mathrm{p}}}$ on snow albedo. Moreover, the effect of dust mass concentration on snow albedo is similar to r_ef. For example, dust mass concentrations of 10 and 100 ppm and r_ef of 100 and 50 µm gave similar α_λ at 500 nm to dust mass concentrations of 2 and 10 ppm and r_ef of 500 µm. According to this result, spectral albedo measurements at a single wavelength are insufficient to obtain the mass fraction of dust in snow cover because the same effect can also be explained by a combination of different ice grain sizes and a nonuniform distribution of dust inside the grains. It means that additional information is needed to determine accurate dust mass concentrations. This may be a set of measurements at various wavelengths in the VIS and NIR spectral ranges.

Figure 7 Broadband snow albedo (α_integrated) variations affected by different dust mass concentrations and snow grain radii for (a) external mixing, (b) internal mixing (uniform), and (c) internal mixing (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$).

3.3 Effects on broadband snow albedo

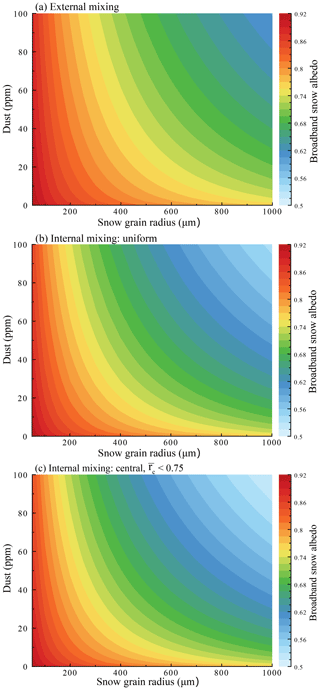
Compared with the spectral optical properties, broadband results can provide more general knowledge for the relevant research community. Figure 7 shows the spectrally weighted α_λ (α_integrated) over 300–2500 nm of a typical surface solar spectrum at midlatitudes to high latitudes, which is comparable with previous studies (Dang et al., 2017; Wang et al., 2017). Because the results of IDM (peripheral) effects on snow albedo fell between results from EDM and IDM (central), we do not consider the case of IDM (peripheral) in the following discussion. Instead, we focus on the effects of dust mass concentration and r_ef on broadband snow albedo for EDM and IDM (uniform, central). Similar to α_λ, α_integrated generally decreased with increasing dust mass concentrations and r_ef such that α_integrated declined more for internal mixing than external mixing. α_integrated showed ranges of 0.60–0.92, 0.54–0.92, and 0.51–0.92 for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively, with dust mass concentrations of 0–100 ppm and r_ef of 50–1000 µm. For a given dust mass concentration and r_ef, α_integrated for IDM (uniform) was smaller than EDM, which is due to higher light absorption in the UV and VIS bands for IDM (uniform) relative to EDM (Fig. 4a). While α_integrated for IDM (uniform) was larger compared with IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), this can be attributed to the fact that radiation is focused near the center of an ice grain with IDM (central) (Ackerman and Toon, 1981; Bohren, 1986), enabling further absorptions from inclusions near the center of a grain due to the lensing effect (Mackowski et al., 1990). For example, α_integrated (dust concentration of 20 ppm, r_ef of 500 µm) was 0.73 for IDM (uniform), less than 0.76 for EDM, but higher than (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) 0.72 for IDM.

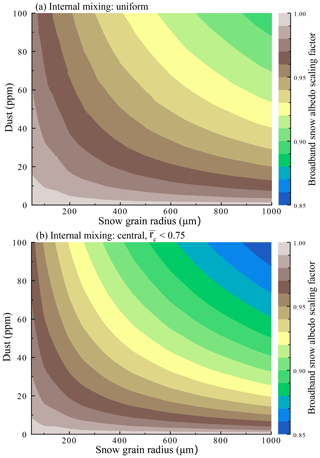
Figure 8 Variations in the broadband snow albedo scaling factor ($E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$, ratio of α_integrated for IDM to EDM) due to different dust mass concentrations and snow grain radii for (a) internal mixing (uniform) and (b) internal mixing (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$).

To quantify the effects of IDM on broadband snow albedo relative to EDM, we defined a broadband snow albedo scaling factor ($E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$), which refers to the ratio of α_integrated of IDM to EDM. Generally, for dust mass concentrations from 0 to 100 ppm and r_ef of 50–1000 µm, $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ varied from 0.89 to ∼1.00 for IDM (uniform) (Fig. 8a) and from 0.85 to ∼1.00 for IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) (Fig. 8b). $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ decreased significantly with increasing dust mass concentration and r_ef. In addition, $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ for IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) was smaller than IDM (uniform). These results have implications for the effects of IDM in real environments. For example, IMC has typical dust concentrations of ∼10 ppm (Wang et al., 2013), so $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ (r_ef of 50–1000 µm) was 0.96–0.99 and 0.95–0.99 for IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively. In contrast, dust concentrations are typically ∼100 ppm in the TP (Ming et al., 2016; Li et al., 2017, 2018), so $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ ranged from 0.89 to 0.98 and 0.85 to 0.96 for IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively. The results show that IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) reduced broadband snow albedo by ∼2.5 % and ∼3.0 %, respectively, in clean snow and ∼6.5 % and ∼9.5 %, respectively, in polluted snow relative to EDM. Moreover, the sensitivity of $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ to mineral dust decreased with increasing dust concentrations. For example, the difference in $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ (r_ef of 500 µm) between dust concentrations of 10 and 20 ppm was 0.011 and 0.015 for IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively, while the corresponding differences between dust concentrations of 90 and 100 ppm were only 0.004 and 0.005. These results provide a convenient method to calculate the albedo of IDM when the albedo of EDM has been obtained for a given dust mass concentration and r_ef.

3.4 Uncertainties

Although we calculated the imaginary RI values of dust using previous studies (Sect. 2.2), there are still large variations which strongly depend on dust composition (e.g., hematite/iron content) (Balkanski et al., 2007; Wagner et al., 2012). To roughly account for this, we estimated the influence of chosen imaginary RI values on spectrally weighted snow albedo ($E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$) by increasing and decreasing the calculated imaginary RI values by 50 %. These changes in imaginary RIs are plausible because they are consistent with other studies (McConnell et al., 2010; Wagner et al., 2012). The results showed that $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ uncertainties attributed to the imaginary RIs of dust were ±3.9 % and ±5.2 % for IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively.

In contrast, observations have displayed large variations in the size distribution of dust in the atmosphere and snow, and this variation is strongly affected by the dust source and transport (Mahowald et al., 2014; Shao et al., 2011). In our standard simulation, we assumed a log-normal dust size distribution with a geometric mean diameter of 0.65 µm and a standard deviation of 2.0 (equivalent to an effective radius of 1.1 µm), which is typical for dust transported over a long range (Formenti et al., 2011; Maring et al., 2003); closer sources of dust tend to be larger (Kok, 2011). Therefore, we investigated the effects of dust particle size on our results by assuming another two log-normal size distributions with effective radii of 2.5 and 5.0 µm, which were within the observed size ranges in the atmosphere and snow and comparable with previously analyzed dust particle sizes (Maring et al., 2003; Shao and Mao, 2016; Zhang et al., 2003). The results showed that the uncertainty of $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ attributed to dust diameter was ±6.1 % for both IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), but it should be emphasized that this uncertainty estimate accounts only for the impact of dust size on snow albedo in the case of external mixing, since the impact on albedo due to dust size cannot be evaluated when using the effective medium approximation. Overall, the total uncertainty of $E_{{\mathit{\alpha }}_{\mathrm{integrated}}}$ from variations in imaginary RIs and dust diameter was ±11.0 % and ±11.2 % for IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively, with dust mass concentrations of 0–100 ppm and r_ef of 50–1000 µm. Specifically, the uncertainty increased with increasing r_ef and dust concentrations.

Figure 9 In situ measurements of dust concentrations in snow (fresh snow, aged snow, and snow pit from field sampling in different regions of the Northern Hemisphere). The solid black circle represents snow samples that were collected days after a significant dust transport event.

3.5 Measurement-based estimate of the effects of dust on snow albedo

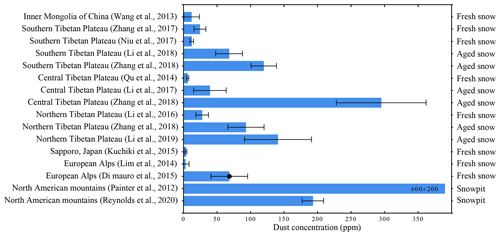
Finally, widespread dust concentrations in snow across the Northern Hemisphere were obtained to assess the effects of dust on snow albedo in real snowpack. Figure 9 shows measured dust concentrations in snow in different regions; dust concentrations spanned a broad range of values because of spatial and temporal variations in emissions, transportation, and deposition among the different regions. Dust concentrations widely varied from ∼3 to ∼600 ppm, with the highest concentration in NAM and lowest in the EP (Di Mauro et al., 2015; Lim et al., 2014; Painter et al., 2012; Reynolds et al., 2020). However, snow samples collected in the days after a significant dust transport event showed that dust concentrations in snow can be up to ∼70 ppm in the EP (Di Mauro et al., 2015). Additionally, the average dust concentrations in fresh snow were 18, 6, and 28 ppm in the southern TP, central TP, and northern TP, respectively, similar to the IMC (12 ppm) (Wang et al., 2013). However, dust concentrations in aged snow (120, 300, and 140 ppm) were 1 to 2 orders of magnitude higher than in fresh snow, indicating the important correlation between snow type and dust concentration (Zhang et al., 2017, 2018).

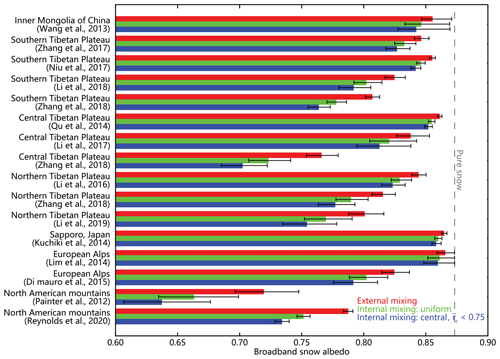
Figure 10 Calculated broadband snow albedo based on dust concentration measurements in different areas for dust–snow external mixing, internal mixing (uniform), and internal mixing (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$). The dashed line represents broadband albedo of pure snow, and the snow grain radius was assumed to be 200 µm.

We calculated the broadband snow albedo for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) based on the measured dust concentrations (Fig. 10). The results showed that broadband snow albedo decreased by 0.8 %, 1.4 %, and 1.6 % in the EP for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively, which was similar to SJ. However, the broadband snow albedo decreased by up to 5.6 %, 8.1 %, and 9.4 % in the EP after a significant dust transport event, indicating strong snow albedo reduction during these events. In addition, broadband snow albedo was reduced by 2.0 %, 3.1 %, and 3.6 % in IMC for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively. Similar results were also found for the southern TP, central TP, and northern TP where the broadband snow albedo for fresh snow was reduced by 2.5 %, 1.4 %, and 3.3 %, respectively, for EDM; 3.9 %, 2.1 %, and 5.1 % for IDM (uniform); and 4.5 %, 2.4 %, and 5.7 % for IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$). However, the broadband snow albedo was more significantly reduced for aged snow: up to 6.0 %, 8.1 %, and 7.5 % for EDM; 9.5 %, 11.6 %, and 10.5 % for IDM (uniform); and 10.9 %, 13.2 %, and 12.3 % for IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) in the southern, central, and northern TP, respectively. This indicates that the effects of dust on snow albedo showed stronger reductions during snowmelt periods. It is worth noting that the effects of dust on snow albedo for aged snow could be underestimated due to the larger snow grain effective radius (r_ef) than fresh snow. Moreover, the largest broadband snow albedo reductions were found in NAM with ranges of 9.8 %–17.6 %, 13.9 %–24.1 %, and 15.9 %–27.0 % for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively. These results suggest that the effects of external or internal dust–snow mixing on snow albedo are particularly significant for the TP and NAM regions, with stronger reductions in albedo. Therefore, these results can have significant impacts on both local hydrological cycles and regional climate change (Oaida et al., 2015; Xie et al., 2018).

Figure 11 Comparisons of snow albedo reduction (Δα) under the cloudy sky caused by dust–snow uniform internal mixing (y axis) and external mixing (x axis) for this study (blue) and He et al. (2019) (red).

4 Discussion

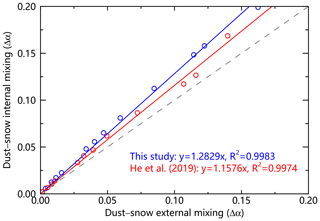
In this study, the application of the effective medium approximation greatly simplifies the complexity of snow radiation transfer calculation for dust–snow internal mixing, and the effect of nonuniform distribution of dust in snow grains on snowpack optical properties are explicitly quantified for the first time. However, it is worth noting that this method has its limitation when applying to large particles (e.g., dust) in snow (Bohren, 1986; Flanner et al., 2012), which can create some errors for the albedo calculation of dust–snow internal mixing. To verify the credibility of our results, we carefully make a comparison with the more rigorous calculations found in He et al. (2019), which used the geometric-optics surface-wave approach (GOS) to consider the impact of dust–snow uniform internal mixing on snow albedo reduction. As shown in Fig. 11, the results show that the enhancement ratio of snow albedo reduction (1.28) due to dust–snow internal mixing (relative to external mixing) is slightly higher than the value (1.16) reported by He et al. (2019), and this deviation is comparable to that caused by snow nonsphericity (He et al., 2019). Therefore, we indicated that the effective medium approximation used in this study is reasonable and reliable.

Over the past few decades, the effects of dust in snow on reductions in albedo have been widely demonstrated (Skiles et al., 2018; Zhang et al., 2018). However, the magnitude of these effects has only been studied in a few regions, and uncertainties still remain. Our study indicates that the albedo of dust-contaminated snowpack can be affected by the dust–ice mixing state. In particular, IDM enhanced light absorption and reduced snow albedo more significantly compared with EDM. For example, in IMC and the TP, IDM reduced snow albedo by ∼5 % relative to EDM at a typical dust mass concentration of 20 ppm and a snow grain radius of 500 µm. This exceeds the contribution of BC to snow light absorption over most areas of North America and the Arctic (Dang et al., 2017). In addition, the effects of IDM on snow albedo were amplified by higher dust mass concentrations and larger snow grain sizes. We therefore strongly suggest that IDM must be considered in future climate models, particularly to more accurately evaluate the climate in areas where snowpack is heavily contaminated with dust and is experiencing melting.

The mixed state between dust and snow gradually progresses from partial external mixing to wholly internally mixed. Therefore, assuming a completely external mixing of dust and snow grains will underestimate the effects of dust on snow albedo and radiative forcing in numerical models (e.g., Dang et al., 2015; Nagorski et al., 2019). Similarly, assuming completely internal mixing of dust and snow grains will overestimate the effects of dust (e.g., He et al., 2019; Liou et al., 2014). Zhao et al. (2014) underestimated the effects of dust by treating wet-deposited dust as externally mixed with snow grains. In future studies, we recommend the actual ratio between external and internal mixing for dust in snow be examined with an environmental scanning electron microscope equipped with a cold stage.

5 Conclusions

In this study, the effects of dust particles on absorption coefficients and snow albedo were theoretically analyzed by combining asymptotic radiative transfer theory and (core–shell) Mie theory. We initially considered external mixing – when dust is present between ice grains – and variations in internal mixing of dust within ice grains. We found that snow spectral absorption coefficients of IDM were larger than EDM across UV to NIR wavelengths but were negligible at wavelengths >1000 nm. The absorption enhancement (relative to EDM) was wavelength-dependent and increased with increased dust concentrations.

Compared with a uniform distribution of dust particles in ice grains, our calculations showed that nonuniformly distributed dust particles may lead to significantly different snow spectral absorption coefficients in the VIS band. Snow spectral absorption coefficients were further increased when all of the dust was positioned in the central part of ice grains, while the maximum absorption coefficient was found when the radius of a dust-polluted core was <75 % of the ice grain radius. In contrast, snow spectral absorption coefficients decreased when all of the dust was positioned in the surface layer of ice grains, and the minimum absorption coefficient was observed in the thin surface layer of dust-polluted ice grains, which was similar for EDM. As a result, broadband snow albedo decreased by up to 21 %, 30 %, and 33 % for EDM, IDM (uniform), and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$), respectively, at dust concentrations of 100 ppm and r_ef of 1000 µm.

Based on comprehensive field measurements across the Northern Hemisphere, the effect of dust on snow albedo in real snowpack was evaluated by assuming external and internal dust–snow mixing. The largest reductions in broadband snow albedo were in NAM because that region had the highest average dust concentrations; IDM (uniform) and IDM (central, $\stackrel{\mathrm{‾}}{r_{\mathrm{c}}}<\mathrm{0.75}$) further decreased snow albedo by 4.6 %–7.8 % and 6.8 %–11.4 %, respectively, compared with EDM. This implies an important influence of internal dust–snow mixing in NAM.