Temperature effects on sulfuric acid aerosol nucleation and growth: initial results from the TANGENT study

New particle formation (NPF) consists of two steps: nucleation and subsequent growth. At present, chemical and physical mechanisms that govern these two processes are not well understood. Here, we report initial results obtained from the TANGENT (Tandem Aerosol Nucleation and Growth Environment Tube) experiments. The TANGENT apparatus enables us to study these two processes independently. The present study focuses on the effects of temperature on sulfuric acid nucleation and further growth. Our results show that lower temperatures enhance both the nucleation and growth rate. However, under temperatures below 268 K the effects of temperature on the nucleation rate become less significant and the nucleation rate becomes less dependent on relative humidity, indicating that particle formation in the conditions of our flow tube takes place via barrierless nucleation at lower temperatures. We also examined the growth of newly formed particles under differing temperature conditions for nucleation and further growth. Our results show that newly nucleated clusters formed at low temperatures can indeed survive evaporation and grow in a warmer environment in the presence of SO₂ and ozone and potentially other contaminant vapors. These results imply that some heterogeneous reactions involving nanoparticles affect nucleation and growth of newly formed particles.

1 Introduction

Atmospheric nanoparticles affect human health and air quality. Newly formed particles can contribute to approximately 30 %–70 % of cloud condensation nuclei (CCN) in the atmosphere (Merikanto et al., 2009; Wang and Penner, 2009; Yu and Luo, 2009; Gordon et al., 2017). New particle formation (NPF) takes place via two steps: initial nucleation (formation of critical clusters) and subsequent growth of nucleated clusters (Kulmala et al., 2013). At present, chemical and physical mechanisms that govern these two processes, as well as the identity of chemical precursors involved in these processes, are still not well understood (Zhang et al., 2012; Yu et al., 2017b; Lee et al., 2019). Current global models fail to represent NPF in the atmosphere for a wide range of temperature and relative humidity (RH) conditions and for different emissions of biogenic and anthropogenic precursors, due to a lack of observations. For example, models predict frequent NPF during the summer in mixed deciduous forests in the United States (Yu et al., 2015), while field observations show an absence of NPF in this region (Kanawade et al., 2011; Lee et al., 2016). Also, current NPF theories are unable to explain the frequent NPF observed in extremely polluted megacities (Yu et al., 2017b; Kulmala et al., 2017).

Temperature and RH are the key thermodynamic properties of aerosol formation and growth (Seinfeld and Pandis, 2016). Nucleation rate (J) is a function of temperature and the Gibbs free energy barrier of cluster formation. At lower temperatures, Gibbs free energy barriers become lower and critical cluster diameters become smaller. Condensational species can affect aerosol growth differently at different temperatures because their saturation vapor pressures are dependent on temperature. For water, RH is the same as saturation ratio and chemical activity. Laboratory experiments of aerosol nucleation and growth as a function of temperature and RH remain limited, although these observations are critical in global models to correctly parameterize NPF under various altitude, latitude and seasonal conditions. Aerosol nucleation experiments are extremely challenging due to various experimental difficulties, including contamination of base compounds (Erupe et al., 2011; Yu et al., 2012). At present there is a lack of consistency between different experiments from different groups and even from the same groups using the same experimental setup (Lee et al., 2019). The lack of reproducibility and consistency of the nucleation experiments greatly hinders our understanding of nucleation mechanisms.

Duplissy et al. (2016) conducted studies of binary homogeneous nucleation of sulfuric acid and water, with and without ions in the CLOUD (Cosmics Leaving OUtdoor Droplets) chamber at different temperatures ranging from 207 to 299 K and RH between 11 % and 58 %. At lower temperatures both ion nucleation and neutral binary nucleation are at the kinetic regime, while at higher temperatures J is strongly dependent on [H₂SO₄], indicating there are high Gibbs free energy barriers at these temperatures. At the nucleation regime, nucleation rates are strongly dependent on RH. Kürten et al. (2016) reported the temperature dependence of ternary nucleation in the CLOUD chamber, at temperatures from 208 to 298 K, [H₂SO₄] between 10⁵ and 10⁹ cm⁻³ and [NH₃] up to ∼1400 pptv. At 208 K, J reached the threshold of 1 cm⁻³ s⁻¹ at [H₂SO₄] of $\sim \mathrm{3}\times {\mathrm{10}}^{\mathrm{6}}$ cm⁻³ for the binary case and at [H₂SO₄] of $\sim \mathrm{5}\times {\mathrm{10}}^{\mathrm{5}}$ cm⁻³ for the ternary case with [NH₃] of 5 pptv. At 298 K, RH has strong effects on the measured J for both charged and neutral ternary nucleation because this increase in RH could lead to a displacement of NH₃ from the stainless steel walls in the CLOUD chamber and lead to an elevated NH₃ background level and consequently to higher J.

Laboratory experiments of growth rates (GRs) of newly nucleated particles are even more sparse. Skrabalova et al. (2014) studied GRs of newly formed particles in a flow tube at temperatures between 283 to 303 K and RH of 1 % and 30 %, designated as “dry” and “wet” conditions, respectively. [H₂SO₄] was varied between 2×10⁸ and 1.4×10¹⁰ cm⁻³. They found different effects of RH on GR: at [H₂SO₄] below 10⁹ cm⁻³, growth is promoted in drier conditions, whereas at [H₂SO₄] higher than 10⁹ cm⁻³, growth favors wetter conditions. Yu et al. (2017a) performed flow tube experiments of sulfuric acid aerosol nucleation at temperatures from 248 to 313 K and RH from 1 % to 79 % under minimal base concentrations ([NH₃] <23 pptv, methylamine <1.5 pptv and dimethylamine <0.52 pptv). Their study provides, for the first time, the temperature and RH dependence of both J and GR. J shows the following dependence within the experimental conditions:

$$\begin{array}{}\text{(1)}& J={\mathrm{10}}^{\mathrm{41.8}}{\left[\mathrm{RA}\right]}^{\mathrm{3}}\left[\mathrm{RH}\right]e^{\frac{-\mathrm{2.4}\times {\mathrm{10}}^{\mathrm{4}}}{T}},\end{array}$$

where RA is relative acidity (or saturation ratio) of sulfuric acid and T is temperature. Their results show that GR is independent of temperature below 290 K but significantly decreases at temperatures above 290 K. RH has a moderate effect on GR.

At present, there is still no clear evidence from atmospheric observations that demonstrates “causal effects” of temperature and RH on NPF (Lee et al., 2019). Recent CLOUD studies have shown that oxygenated organics formed from biogenic volatile organic compounds (BVOCs) can grow newly nucleated particles in a wide range of tropospheric temperatures (Stolzenburg et al., 2018). This is because gas-phase autooxidation reactions involved in the formation of highly oxidized molecules (HOMs) usually have strong temperature dependencies, with higher reaction rates at higher temperatures (Frege et al., 2018), whereas nucleation is favored at lower temperatures.

Another important perspective of temperature effects on NPF is the effects of temperature on evaporation of newly nucleated clusters and nanoparticles that have undergone transport to differing temperature conditions for further growth. Particle concentrations in the free troposphere in marine regions have been shown to be due to sulfuric-acid-driven nucleation of nanoparticles as a result of oxidation of upwardly transported and oceanic dimethylsulfide. These particles then undergo downward transport to become reservoirs of nanoparticles in the boundary layer. This has been observed and modeled by several groups over the previous decades (Russell et al., 1994; Raes, 1995; Clarke, 1993).

The lack of local NPF events in Amazon forests has been a confounding observation for many years (Varanda Rizzo et al., 2018) and yet there are reservoirs of nuclei-mode particles at the surface which do not form typical “banana” plots of aerosol size distributions observed elsewhere (Martin et al., 2016). Understanding the origin of these nuclei-mode particles has been a subject of recent studies. Wang et al. (2016) reported that while NPF does not take place in Amazon forests at the surface level during the dry and wet season, NPF takes place in the colder free troposphere; these newly formed particles can be transported down to the boundary layer to become a reservoir of nanoparticles at the surface. This downward transport process is very similar to the above-mentioned marine boundary layer nanoparticle processes (Russell et al., 1994; Raes, 1995; Clarke, 1993). A subsequent question is thus whether newly nucleated clusters and nanoparticles in the free troposphere can survive evaporation during their transport to the warmer boundary region.

At present, the temperature effects on the growth of newly formed particles crossing different temperature regions have not been examined in a controlled laboratory environment. In order to address this shortcoming in laboratory experiments of this relatively common phenomenon, we have constructed the TANGENT apparatus, consisting of a temperature- and RH-controlled nucleation flow tube that permits study of gas-to-particle conversion over a wide parameter space. This nucleation region is connected to a room temperature growth tube where conditions for further growth of these nanoparticles can differ dramatically from those of their nucleation. With the suite of instruments monitoring precursor gases as well as particle size distributions from sub-3 nm up to CCN sizes at key areas of the flow apparatus, the critical processes that atmospheric nanoparticles undergo can be studied and parameterized with TANGENT.

Here, we present the initial results of the TANGENT experiments conducted during the Intensive Observation Period (IOP) study in June and July 2018. In the present study, we report the temperature effects on J and GR of sub-2 nm particles in the nucleation tube. We also discuss how temperature differences in the nucleation and growth tube affect the potential evaporation of newly nucleated clusters while these clusters are transported from the colder to the warmer temperature region.

2 Methods

2.1 The TANGENT experimental setup

Figure 1 shows the schematic diagram of the TANGENT apparatus. The TANGENT consists of the two flow tubes (FTs) to enable studies of nucleation (FT-1) and subsequent growth (FT-2). The nucleation tube was built by the University of Alabama in Huntsville, and the growth tube was built by University of Delaware. TANGENT allows for nucleation to be observed as a separate process from growth. With a short residence time (45 s), precise temperature control and the ability to produce H₂SO₄ at concentrations spanning 10⁶ to 10⁹ cm⁻³, FT-1 is able to nucleate and monitor particles from precursor gases at a variety of conditions. After particles are nucleated in FT-1 where size distributions are recorded, particles are then transported to the growth tube (FT-2), where the particles undergo further growth at room temperature for a longer residence time (4 min) with the ability to precisely control other trace gases in the growth environment. According to our knowledge, this is the first flow tube experimental setup that allows for aerosol nucleation and growth processes to be investigated independently and simultaneously.

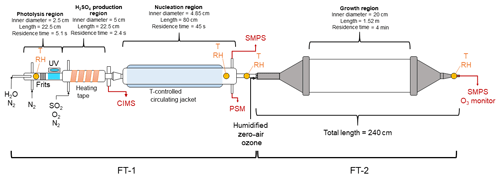
Figure 1 Schematic diagram of the TANGENT experimental setup. This setup consists of two flow tubes (FTs). T indicates temperature. FT-1 is used as the nucleation region and FT-2 as the growth region. Table 1 shows the typical experimental conditions used during the 2018 IOP study.

The experimental setup of the nucleation region (FT-1) was based on several previous studies (Benson et al., 2008, 2009, 2011; Erupe et al., 2011; Young et al., 2008; Yu et al., 2012, 2017a). It consists a photolysis region where H₂SO₄ is generated photochemically and monitored and followed by a temperature-controlled nucleation tube. In the photolysis region, OH radicals were produced via photodissociation of water vapor in a quartz tube using a mercury lamp (Pen-Ray model 11SC-1). The mercury lamp was located in a temperature-controlled enclosure filled with a constant nitrogen flow. UV intensity was adjusted with an aperture over the slit in the enclosure, exposing the quartz tube to the UV lamp. UV intensity was monitored with a cesium iodide (CsI) phototube (Hamamatsu model R5764) and picoammeter (Keithley 6732). Measurements of UV intensity were taken to ensure consistency between experimental trials. SO₂, O₂ and N₂ gases were introduced to the flow tube immediately after the photolysis region. H₂SO₄ forms from the SO₂+OH reaction. Heating tape was applied to the H₂SO₄ production region to suppress nucleation prior to entering the temperature-controlled nucleation zone. The H₂SO₄ production region was monitored with a condensation particle counter (CPC, TSI 3776) and particle size magnifier (PSM, Airmodus A09) to ensure no particles were formed before the flow entered the nucleation region.

H₂SO₄ concentrations at the beginning of the nucleation tube were measured with a nitrate-based chemical ionization mass spectrometer (CIMS), based on Eisele and Tanner (1993), continuously during the experiments. Calibration of [H₂SO₄] with the nitrate-based CIMS was described previously by Young et al. (2008). The CIMS was operated with an inlet flow of 5.0 slpm and an ion–molecule reaction time of 0.05 s. The lower limit of detection was $\sim \mathrm{1}\times {\mathrm{10}}^{\mathrm{5}}$ cm⁻³.

No base compounds were added, but base compounds were still present in the flow tube as impurities likely generated from deionized water used for H₂SO₄ production and RH control (Erupe et al., 2011; Yu et al., 2012). NH₃ and amines were not measured during the 2018 IOP, but they were measured under very similar experimental conditions during the entire 2017 IOP with an ethanol-based CIMS at the beginning of the nucleation tube (You et al., 2014; Yu and Lee, 2012). Detection limits of NH₃ and amines in our CIMS were parts-per-trillion by volume (pptv) or sub-pptv with a 1 min integration, as previously discussed elsewhere (Benson et al., 2010; Erupe et al., 2011; You et al., 2014; Yu and Lee, 2012).

The nucleation tube is an 80 cm long Pyrex glass tube with an inner diameter of 4.85 cm. The temperature of the nucleation tube was controlled with a circulating bath and a water-based potassium formate heat transfer fluid (Dynalene HC-50, Dynalene, Inc.) to adjust the temperature between 258 and 297 K. RH was adjusted by directing some of the dry nitrogen makeup flow through deionized water in a water bubbler. Thus, in our experimental setup, changes in RH in the nucleation tube did not affect the OH radical concentrations in the photolysis region. Temperature and RH probes (CS-215, Campbell Scientific) were used to monitor the conditions at the beginning of the photolysis region, as well as at the end of the nucleation region. An additional temperature and RH probe (Traceable, Fisher Scientific) was applied inside the nucleation tube to confirm the RH. Residence time in the nucleation region (FT-1) was 45 s.

Particle concentrations at the exit of FT-1 were measured with a PSM (Vanhanen et al., 2011). The PSM saturator flow was operated with a 240-step cycle between 0.1 and 0.9 slpm at a rate of 1 s per step, giving saturator-flow-dependent cutoff sizes between 1.26 and 2.85 nm. These cutoffs were resolved to six size bins in an inversion method based on Lehtipalo et al. (2014), producing size distributions with six size bins: 1.26–1.53, 1.53–1.79, 1.79–2.06, 2.06–2.32, 2.32–2.59 and 2.59–2.85 nm. Particle concentrations were also monitored with a scanning mobility particle sizer (SMPS) consisting of a differential mobility analyzer (DMA, TSI 3080) and a CPC (TSI 3776). However, under the typical experimental conditions, particles above 3 nm in diameter did not appear even with the most favorable conditions for nucleation and growth (e.g., high [H₂SO₄], high RH and low temperature).

During the experiments, [H₂SO₄] was varied by adjusting the aperture on the mercury lamp housing (hence varying [OH]) at a fixed [SO₂], allowing for a range of [H₂SO₄] spanning roughly 1 order of magnitude for a given dilution of SO₂. [H₂SO₄] was further varied by adjusting the SO₂ dilution, allowing for measurements spanning [H₂SO₄] of 10⁶ to 10⁹ cm⁻³. The PSM measurements showed that each experimental condition was “stabilized” typically after ∼30 min for a specific set of [H₂SO₄], RH and temperature.

The photolysis and nucleation tubes were cleaned thoroughly with deionized water, citric acid solution and ethanol and allowed to dry for 24 h while heated to 60 ^∘C with pure N₂ flowing through the flow tube. Between experiments, the photolysis and nucleation tubes were continuously flushed with dry vaporized liquid nitrogen. A constant flow of N₂ was passed through the experimental apparatus at all times during the IOP to ensure that the conditions inside the tube would remain constant and there would be no intrusion of room air.

The nucleated clusters (smaller than 2 nm) were transported to the growth tube (FT-2) for further growth with an extended residence time (4 min). FT-1 and FT-2 were coupled with an 0.2 m stainless steel tube with additional inlet ports for injection of ozone, zero air and SO₂. The growth tube was described by Krasnomowitz et al. (2019) and Stangl et al. (2019). The growth tube consists of a 1.52 m long and 0.2 m inner diameter fused quartz tube fitted with stainless steel funnels on each end that reduce the inner diameter down to 0.051 m. The total volume of the tube and entrance and exit funnels is 52.4 L, giving a surface-to-volume ratio of 0.24 cm⁻¹. The 0.2 m straight tube fitting allows carrier and reactant gases to enter the tube via an axial inlet port and continuous flow through the entire length of the reactor during the course of an experiment. The end of the tube was attached to an ozone monitor (Thermo Fisher Scientific 49i), a hygrometer (Traceable, Fisher Scientific) and an SMPS (TSI 3938, 3788).

FT-2 has several ports at the inlet to inject additional gases into the system to observe their effects on further growth of freshly nucleated particles. Ozone was added to FT-2 using the calibration ozone generator on a Thermo Fisher Scientific 49i. Ozone was varied in FT-2 by adjusting the UV intensity of the calibration lamp. The residence time in FT-2 could be changed by varying the flow of zero air into FT-2. During a typical experimental run using both FT-1 and FT-2, conditions would only be changed in FT-2, with temperature, RH and precursor species in FT-1 held constant throughout the entire experiment. The experiments undertaken in this study measured two effects in the system: the effect of changing temperature in the nucleation region as well as the effect of varying ozone (in the copresence of SO₂) in the growth region.

2.2 Calculations of nucleation (J) and growth rate (GR)

Calculations of J were made based on Yu et al. (2017a). Briefly, J was calculated according to the following approximation:

$$\begin{array}{}\text{(2)}& J_{\mathrm{0}}\approx N_{\mathrm{tot}}\times n{k}_{\mathrm{L}},\end{array}$$

where J₀ represents the nucleation rate corresponding to the initial sulfuric acid concentration ([H₂SO₄]₀) measured at the beginning of the nucleation tube, N_tot the total number concentration of particles detected at the end of the nucleation region, n the nucleation theorem power and k_L the diffusion-limited, pseudo-first-order wall loss coefficient (Hanson and Eisele, 2000). The nucleation theorem power n was experimentally determined for each set of experiments by the linear fit of log N_tot vs. log [H₂SO₄]₀, Our k_L was typically 0.01 s⁻¹. Equation (2) allows us to obtain J₀ at different [H₂SO₄]₀ using n and N_tot of the formed clusters (all smaller than 2 nm in the present study).

To calculate GR, the critical cluster size was determined experimentally, with the critical cluster size corresponding to the y intercept of the linear fit between the mean particle diameter, D_p, and the [H₂SO₄]₀ (e.g., Fig. 3). D_p was obtained using the inversion of the PSM size distribution measured at the end of FT-1. GR was calculated by the difference between the critical size and D_p divided by the nucleation time.

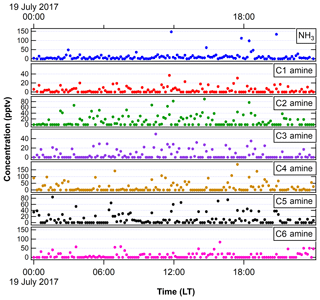
Figure 2 NH₃ and amines measured with the ethanol-based CIMS in FT-1 during the 2017 IOP in a very similar experimental condition as in 2018 IOP. We show 1 d of measurements (19 July 2017) here as an example.

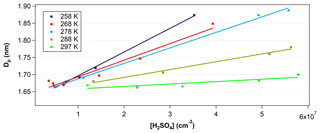
Figure 3 Mean diameter of particles (D_p) inverted from the PSM measurements at the end of the nucleation tube as a function of [H₂SO₄] and temperature. Data points were taken at RH between 20 % and 30 %. Solid lines are linear fittings of the measurement data (colored squares) under different temperatures. Error in [H₂SO₄] is estimated to be ±60 %. Error in D_p at this size range is estimated to be ±0.2 nm or approximately ±12 %.

The growth rate factor k_G is defined as the growth rate enhancement over 1 pptv H₂SO₄, causing 1 nm h⁻¹ of growth. The collision limited condensation of H₂SO₄ of 1 pptv contributes roughly 1 nm h⁻¹ of growth rate at a temperature of 298 K (Nieminen et al., 2011). Thus, the k_G is an indicator of the deviation of the actual growth rate compared to this conventional collision-limited GR. The k_G were determined by the expression derived by Yu et al. (2017a):

$$\begin{array}{}\text{(3)}& k_{\mathrm{G}}={\displaystyle \frac{\mathrm{\Delta }{D}_{\mathrm{p},\mathrm{tr}}\times {\mathrm{10}}^{\mathrm{7}}{\mathrm{cm}}^{-\mathrm{3}}}{[{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}{]}_{\mathrm{0}}}}{\displaystyle \frac{k_{\mathrm{L}}}{\mathrm{1}-e^{-n{k}_{\mathrm{L}}{t}_{\mathrm{r}}}}},\end{array}$$

where ΔD_p,tr represents the change in diameter over the residence time (t_r) in the nucleation tube (FT-1). $\frac{\mathrm{\Delta }{D}_{\mathrm{p},\mathrm{tr}}}{[{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}{]}_{\mathrm{0}}}$ was experimentally determined from the slope of the D_p vs. [H₂SO₄]₀ plot (e.g., Fig. 3). $\frac{k_{\mathrm{L}}\times {\mathrm{10}}^{\mathrm{7}}{\mathrm{cm}}^{-\mathrm{3}}}{\mathrm{1}-e^{-n{k}_{\mathrm{L}}{t}_{\mathrm{r}}}}$ can be calculated from n and k_L values. The k_G is the product of this slope and $\frac{k_{\mathrm{L}}\times {\mathrm{10}}^{\mathrm{7}}{\mathrm{cm}}^{-\mathrm{3}}}{\mathrm{1}-e^{-n{k}_{\mathrm{L}}{t}_{\mathrm{r}}}}$. Extrapolation to the y axis of Fig. 3 gives a value for the critical cluster diameter. $\mathrm{\Delta }{D}_{\mathrm{p},\mathrm{tr}}=\mathrm{0}$ for the critical cluster, and thus D_p,critical will be equal to the y intercept of the linear fit of each data set.

2.3 Uncertainty analysis

The uncertainties in the J₀ calculation arise from uncertainties in CIMS H₂SO₄ measurements, measurements of particle concentrations in the PSM and the size inversion from PSM-measured number concentrations. The uncertainty in the H₂SO₄ CIMS is approximately 60 % (Erupe et al., 2010; Eisele and Tanner, 1993; Benson et al., 2008, 2009; Kürten et al., 2012; Petäjä et al., 2009). The wall loss calculation has ±7 % uncertainty (Hanson and Eisele, 2000). The measurement error in the PSM size distribution, based on the standard error calculated between run-to-run experiments under identical conditions, is ±26 %. The uncertainties in inversion of the particle diameter from the PSM measurement are estimated to be around 12 % (or ±0.2 nm) for inorganic particles in the size range observed in this experiment (Lehtipalo et al., 2014, 2016; Kulmala et al., 2013; Yu et al., 2017a). Propagation of the errors in sulfuric acid measurement, wall loss and the PSM measurements results in an overall uncertainty of ±65.5 % in the calculation of J₀.

3 Results and discussion

3.1 Nucleation and growth in FT-1

Table 1 shows the typical experimental conditions used in FT-1 (nucleation tube) and FT-2 (growth tube) during the 2018 IOP study. In the nucleation tube, temperature was varied from 258 to 297 K and RH from 4 % to 85 %. [H₂SO₄] spanned from 10⁶ to 10⁸ cm⁻³, corresponding to RA of 10⁻⁵ to 10⁻². RA was calculated using the sulfuric acid saturation vapor pressures provided by Vehkamaki et al. (2002). NH₃ and amine measurements were not taken during this study, but they were undertaken during the 2017 IOP campaign using the same experimental apparatus, precursor gases and cleaning schedule as the 2018 campaign that is the concern of this study. The CIMS-measured NH₃ (during the 2017 IOP) was 14.2±6.7 pptv (Fig. 2). Thus, the ratio of [NH₃]∕[H₂SO₄] ranged from 0.6 to 268. According to Schobesberger et al. (2015) and Dunne et al. (2016), these ratios represent ternary nucleation and some nucleation in a transition regime between binary and ternary, when only considering the effects of NH₃ (without amines). Amine concentrations were detected at levels ranging from 4.5±2.60 pptv for C1 amines to 44.8±41.8 pptv for C2 amines (Fig. 2). Overall, the measured nucleation rates ranged from 10 to 10⁵ cm⁻³ at higher temperatures and lower [H₂SO₄] up to 10⁵ cm⁻³ at lower temperatures and higher [H₂SO₄]. The observed GR ranged from 1 to 80 nm h⁻¹. The growth tube (FT-2) was kept at room temperature (297 K) and dry conditions (RH of 10 %). SO₂ was added in the range from 100 ppbv (parts-per-billion by volume) to 5 ppmv (part-per-million by volume) and ozone from 0 to 248 ppbv.

Table 1 Typical experimental conditions used in FT-1 and FT-2. J in FT-1 is the nucleation rate for sub-2 nm particles. J in FT-2 is the formation rate of total particles in the size range from 3 to 60 nm. The particle mean diameter ranged from 2.7 to 3.4 nm depending on the ozone concentration. GR in FT-1 is the growth rate of sub-3 nm particles. GR in FT-2 is the growth rate of total particles from 3 to 60 nm.

Figure 3 shows the measured D_p with the PSM at the end of FT-1 as a function of the initial [H₂SO₄]₀ at temperatures between 258 and 297 K. [H₂SO₄]₀ was varied from 8×10⁶ to 7×10⁷ cm⁻³. The RH was kept in relatively narrow range between 20 % and 30 %. The y intercept in Fig. 3 indicates the critical cluster diameter was estimated to be between 1.6 and 1.7 nm depending on temperature, with lower temperatures resulting in smaller critical cluster diameters. However, since the PSM inversion that determines D_p has an uncertainty of ±0.2 nm, it is difficult to discuss the temperature trends of the critical cluster diameter. This critical size is consistent with the studies of Kulmala et al. (2013) and Almeida et al. (2013), which determined critical cluster diameters of 1.5±0.3 and 1.7 nm, respectively. For a given [H₂SO₄]₀, the mean D_p at the end of the 45 s residence time in FT-1 was larger for lower temperatures. Previously, Glasoe et al. (2015) and Yu et al. (2017a) have also shown increasing GR with increasing [H₂SO₄] from flow tube experiments. The slope of D_p vs. [H₂SO₄]₀ increased with each 10^∘C decrease in temperature over the course of these experiments. Thus, the growth rate factor k_G also increased with subsequent temperature decreases (e.g., from 1.27 at 297 K to 12.6 at 258 K). These results indicate that lower temperatures promote the faster growth of particles due to the reduction in saturation vapor pressures of H₂SO₄ at lower temperatures.

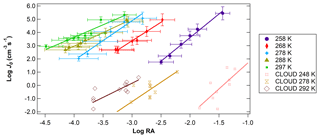
Figure 4 Log J₀ vs. log RA for different temperatures at a relatively constant RH (41 %–45 %). Temperatures ranged from 258 to 297 K. CLOUD data for similar RA and temperature conditions from Dunne et al. (2016) are also shown for comparison.

Figure 4 shows the relationship of log J vs. log RA for different temperatures. Experiments were conducted at 10 K intervals, starting from 297 K down to 258 K for RH between 41 % and 45 %. Across all temperature and RH experiments conducted, in general, J values were shifted 2 to 3 orders of magnitude above previous literature values of flow tube nucleation studies (Brus et al., 2010; Yu et al., 2017a) and 4 to 5 orders of magnitude above CLOUD measurements of H₂SO₄ nucleation as shown in the figure using CLOUD data from Dunne et al. (2016). This upward shift was consistent across trials. Based on our measured NH₃ and amine concentrations (Fig. 2), this upward shift is consistent with the nucleation rate enhancement due to synergistic effects of NH₃ concentrations on the order of 20 to 30 pptv and dimethylamine concentrations on the order of 5 pptv reported by other studies (Glasoe et al., 2015). There was a consistent relationship between log J and log RA. Except for 288 and 297 K, where the slope of log J vs. log RA was approximately 2, and the slope was 3 for all trials, with the best-fit lines shifting towards higher values of RA as temperature decreased. Hanson and colleagues (Glasoe et al., 2015; Zollner et al., 2012) provided comprehensive analysis of log J vs. log RA (or log [H₂SO₄]) obtained from flow tube studies. In general, flow tube studies from various groups have shown slopes between 3 and 6 for the ternary system (Glasoe et al., 2015; Zollner et al., 2012; Brus et al., 2010; Berndt et al., 2014; Erupe et al., 2011; Yu et al., 2012; Hanson et al., 2017). Using the CLOUD experiments (Dunne et al., 2016; Almeida et al., 2013; Kirkby et al., 2011) also showed the slope of 3 for the ternary system. This slope is consistent with the base-stabilization mechanism provided by Chen et al. (2012) and Jen et al. (2014, 2016), who concluded that the bottleneck clusters contain 3–4 H₂SO₄ molecules with at least one base molecule. It was previously believed that the slope of log J vs. log RA dictates the amount of H₂SO₄ molecules present in the critical cluster based on classical nucleation theory (CNT) (Kashchiev, 1982; McGraw and Zhang, 2008), which then would imply here that the critical cluster contains three H₂SO₄ molecules for the ternary system. However, more recent work by Malila et al. (2013) and Vehkamäki et al. (2012) has shown that this conclusion may be an oversimplification of the mechanism of particle formation resulting from an application of CNT with an incomplete understanding of the free energy maxima and minima.

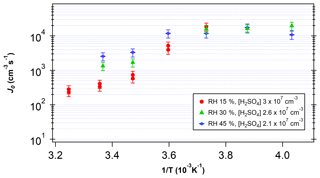
Figure 5 shows the measured J as a function of temperature for [H₂SO₄] between 2×10⁷ and 3×10⁷ cm⁻³ and RH between 15 % and 45 %. J increased with decreasing temperatures in the temperature range above 268 K. The higher J at lower temperatures is consistent with predictions from CNT (Seinfeld and Pandis, 2016). However, a shift in slope is visible around 268 K, indicating that the dependence of J on temperature becomes less significant at low temperatures. The variation seen across RH at higher temperatures also becomes negligible below this temperature. Thus, these results indicate that at temperatures below 268 K, Gibbs free energy barriers are reduced significantly.

Figure 5 Log J₀ vs. 1∕T for [H₂SO₄] between 2×10⁷ and 3×10⁷ cm⁻³. T is the temperature in FT-1. RH ranged from 15 % to 45 %. Vertical bars indicate one standard deviation of the measured nucleation rates.

3.2 TANGENT experiments: further growth of clusters in FT-2

In order to determine whether newly formed particles nucleated at lower temperatures can survive downward transport to warmer temperature conditions, we conducted two tests (Figs. 6 and 7). The first test had a much lower temperature in FT-1 (268 K) than in FT-2 (297 K) and the second test had the same temperature (297 K) in both tubes. Figure 8 shows the GR and particle survival percentage, calculated by dividing the particle concentration at the end of FT-2 by the particle concentration at the end of FT-1. During the first test (Fig. 6), the average particle concentration coming out of FT-1 was 2×10⁵ cm⁻³ with a median diameter of 1.9 nm at [H₂SO₄]₀ of 6×10⁷ cm⁻³ and RH of 10 %. These newly formed particles were further mixed with an additional zero air flow at a 1:6 dilution. The [SO₂] was 83 ppbv and the ozone level was varied from 0 to 248 ppbv in FT-2. No particles were observed coming out of FT-2 when ozone was absent, indicating that SO₂ alone does not cause nucleation and growth of clusters. However, in the presence of ozone and SO₂ together, continuous nucleation and further growth of transported clusters took place in FT-2. The particle concentration measured at the end of FT-2 was closely correlated with the ozone concentration: the particle concentration ranged from 3×10² cm⁻³ (with D_p of 2.8 nm) at the lowest ozone of 28 ppbv up to 5×10⁴ cm⁻³ (D_p of 3.4 nm) at the maximum ozone of 248 ppbv. Thus, the particle concentration in FT-2 at the highest ozone was even greater than that coming out of FT-1 after dilution, indicating that a high ozone load resulted in additional nucleation and the further growth of clusters in FT-2; this is indicated by the greater than 100 % particle survival shown in Fig. 8b. This could be the result of the remaining H₂SO₄ vapor passing through to FT-2, but we excluded this possibility. After considering wall loss in FT-1 and the 1:6 dilution FT-2, [H₂SO₄] in FT-2 was estimated to be 1.15×10⁶ cm⁻³, which can result in J only on the order of 10¹ cm⁻³ s⁻¹ at room temperature and dry conditions, as shown from the results obtained in FT-1 (e.g., Fig. 4). However, the measured formation rate (J) of particles in FT-2 was 190 cm⁻³ s⁻¹, in contrast to this estimation. There may be another possibility that ozone reacted with possible organic impurities in FT-2 to produce OH, which oxidized SO₂ to produce H₂SO₄ that nucleated in FT-2, but no organics were added in our experiments. At present it is not clear what is the cause of nucleation in FT-2 and this requires future study. However, it was clear that the copresence of ozone and SO₂ was an important factor in preventing evaporation of newly formed particles and facilitated their further growth at a higher temperature. The overall GR of particles in FT-2 was from 14.9 to 23.1 nm h⁻¹, depending on the ozone concentration. These results may imply some heterogeneous reactions occurring on acidic sulfuric acid clusters, in a similar way to how sulfate is formed from oxidation reactions of SO₂ on acidic particles, as proposed by Hung and Hoffmann (2015).

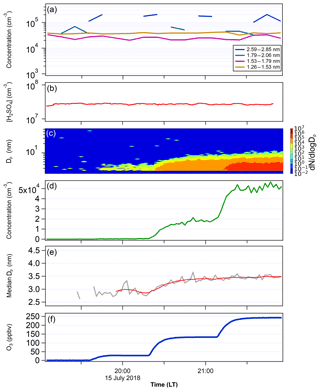
Figure 6 (a) The PSM-inverted size distribution and (b) [H₂SO₄] measured in FT-1 during the temperature gradient TANGENT experiment. FT-1 was at 268 K with a residence time of 45 s. Total concentration at the end of FT-1 was 1.79×10⁵ cm⁻³ with a mean D_p of 1.9 nm. (c) SMPS-measured particle size distribution, (d) total number concentration, (e) the particle median diameter D_p and (f) ozone concentrations in FT-2. FT-2 was kept at 297 K and the residence time was 4 min. The red line in (e) indicates the average values of D_p. SO₂ was 500 and 83 ppbv in FT-1 and FT-2, respectively. H₂SO₄ was not measured in FT-2; however, after considering wall loss in FT-1 and the 1:6 dilution FT-2, [H₂SO₄] in FT-2 was estimated to be 1.15×10⁶ cm⁻³.

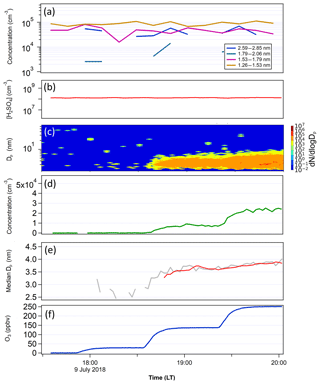
Figure 7 The same as Fig. 6, except that FT-1 and FT-2 had the same temperature in this test. Total particle concentration at the end of FT-1 was 1.7×10⁵ cm⁻³, with a mean D_p of 1.9 nm and [H₂SO₄] in FT-1 was 1.3×10⁸ cm⁻³. In FT-2, the [H₂SO₄] was estimated to be 2.5×10⁶ cm⁻³.

The second test was conducted with FT-1 and FT-2 both at a constant temperature of 297 K (Fig. 7). FT-1 was held at a constant [H₂SO₄]₀ of 1.3×10⁸ cm⁻³. The total particle concentration out of FT-1 for this experiment was 1.7×10⁵ cm⁻³, with a mean diameter of 1.9 nm. Aside from the higher [H₂SO₄]₀, which was necessary to produce the same particle concentration in the higher temperature FT-1, particle count and mean diameter were similar to the experiment with a cold FT-1 (Fig. 6). However, the size distributions in Figs. 6a and 7a indicate that there were more particles in the higher diameter size bins when the temperature in FT-1 was lower. At the end of FT-2, particle concentration and size were again closely correlated with ozone concentration, ranging from 8.6×10¹ cm⁻³ (D_p=2.7 nm) at the lowest ozone concentration up to 2.3×10⁴ cm⁻³ (D_p=3.8 nm) at the highest ozone load. These concentrations and sizes result in growth rates ranging from 12.0 to 28.1 nm h⁻¹, indicating that the growth at the highest ozone concentrations was faster during the constant temperature experiment. This was due to a greater concentration of smaller particles formed at higher [H₂SO₄]₀ in the second test.

Examining the differences between the temperature gradient (Fig. 6) and constant temperature (Fig. 7) experiments, we see notable effects of temperature gradient on the survival of particles to the end of FT-2. While the average size at the end of the nucleation tube was similar in each experiment (D_p=1.9 nm with 268 K FT-1; D_p=1.9 nm with 298 K FT-1), we can see from the size distributions in each experiment that the low-temperature FT-1 resulted in a greater concentration of particles in the larger size bins. These particles (once they are stabilized, e.g., by base compounds) are more thermodynamically stable and therefore have a greater ability to survive evaporation when transported to the warmer environment of FT-2. Particles formed in warmer environments have a greater energy barrier to overcome before they become stable enough to grow spontaneously (Lovejoy et al., 2004). Figure 8 further shows that both the particle growth rate and the tendency for the particles to survive to the end of FT-2 were clearly correlated with ozone concentration, indicating that the concentration of ozone in FT-2 was a critical factor in the persistence, growth and nucleation that occurred in FT-2 during these experiments.

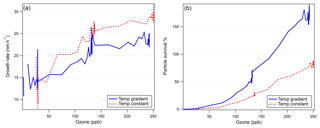
Figure 8 (a) Particle growth rate as a function of ozone concentration in FT-2 during each experiment and (b) the particle survival percentage as a function of ozone concentration in FT-2. Particle survival percentage over 100 % indicates nucleation occurring in FT-2 due to an unknown heterogeneous process.

The additional nucleation occurring in FT-2 in the copresence of SO₂ and ozone cannot be explained by current knowledge. These results suggest some possible heterogeneous reactions involving SO₂ and ozone on acidic clusters. Understanding how SO₂ and ozone interact each other on the acidic nanoparticles to facilitate nucleation and growth require future studies.

We examined the additional effects of organics and base compounds on GR in FT-2. First, to see the effects of possible impurities of organics, we used the growth rate parameterization from Tröstl et al. (2016):

$$\begin{array}{}\text{(4)}& \mathrm{GR}=k{D}_{\mathrm{p}}{\left[\mathrm{HOM}\right]}^p.\end{array}$$

Solving Eq. (4) for [HOM] using our observed growth rates of 12.0 to 28.1 nm h⁻¹, D_p=1.9 nm, $k=\mathrm{5.2}\times {\mathrm{10}}^{-\mathrm{11}}$ and p=1.424 (Tröstl et al., 2016) results in a [HOM] of 2.8 to 3.8 pptv necessary to account for these growth rates. The amount of monoterpene required to produce that amount of HOMs was then calculated using Equation 16 from Trostl et al. (2016):

$$\begin{array}{}\text{(5)}& \left[\mathrm{MT}\right]={\displaystyle \frac{\left[\mathrm{HOM}\right]\cdot \mathrm{CS}}{Y_{\mathrm{1}}{k}_{\mathrm{1}}\left[{\mathrm{O}}_{\mathrm{3}}\right]}}.\end{array}$$

Using the yield of HOMs from ozonolysis of monoterpenes Y₁=2.9 % (Kirkby et al., 2016), temperature-dependent reaction rate of ozone and α-pinene $k_{\mathrm{1}}=\mathrm{8.06}\times {\mathrm{10}}^{-\mathrm{17}}$ (Khamaganov and Hites, 2001), and 10⁻³ for condensation sink (CS, calculated from the measured size distributions in FT-2), it would require between 100 and 1000 ppbv of monoterpenes to account for that concentration of HOMs. These high concentrations were very unlikely to be present in FT-2 considering the rigorous cleaning of the TANGENT apparatus and the lack of added monoterpenes to the system.

Second, to see the possible multicomponent growth due to the presence of ammonia in the system, we utilized the parameterization of multicomponent growth from Eq. (4) of Lehtipalo et al. (2018):

$$\begin{array}{}\text{(6)}& \mathrm{GR}=k_{\mathrm{1}}{\left[{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\right]}^a+k_{\mathrm{2}}{\left[{\mathrm{H}}_{\mathrm{2}}{\mathrm{SO}}_{\mathrm{4}}\right]}^b{\left[{\mathrm{NH}}_{\mathrm{3}}\right]}^c+k_{\mathrm{3}}{\left[\mathrm{Org}\right]}^d,\end{array}$$

where k₁ and k₂ are $\mathrm{2.05}\times {\mathrm{10}}^{-\mathrm{7}}$ and $\mathrm{6.69}\times {\mathrm{10}}^{-\mathrm{11}}$, respectively (Lehtipalo et al., 2018), and $a=b=c=d=\mathrm{1}$. Assuming [Org] =0 for simplicity and [H₂SO₄] and [NH₃] values from the temperature gradient experiment (1.15×10⁶ cm⁻³ H₂SO₄ and 14 pptv NH₃ estimated from 2017 IOP measurements), we should expect a GR of 0.24 nm h⁻¹. However, this calculation only considers the presence of NH₃ in the system, when it is very likely that there were amines present at pptv levels (e.g., Fig. 2). Glasoe et al. (2015) and Yu et al. (2012) found synergistic effects on GR when both NH₃ and methylamine and dimethylamine are present, which can enhance GR by 1 to 2 orders of magnitude and would put these calculated GR values in range of the observed 23.1 nm h⁻¹ GR seen in FT-2.

Furthermore, cluster–cluster collision in the presence of stabilizing base compounds such as trace amines that were present in TANGENT may enhance growth by an order of magnitude (Lehtipalo et al., 2016). Thus, it seems that the observed high GRs in FT-2 were in some part due to multicomponent effects of base compounds and cluster–cluster collisional growth effects on GR, in addition to potential heterogeneous processes speculated above.

4 Conclusions and implications

We have conducted experiments to study the temperature dependence of aerosol nucleation and growth using the TANGENT setup. This setup consists of two flow tubes that enables us to study nucleation and subsequent growth independently. In the nucleation tube, temperature was varied from 258 to 297 K and RH from 4 % to 85 %. [H₂SO₄] spanned 10⁶ to 10⁸ cm⁻³, which corresponds to RA of 10⁻⁵ to 10⁻². Based on the measured [NH₃] to [H₂SO₄] ratios, it was most likely that nucleation took place via the ternary process. The growth tube was kept at room temperature and the dry condition (RH of 10 %). SO₂ was present at 100 ppbv to 5 ppmv and ozone was present at 0 to 248 ppbv.

Our results indicate that lower temperatures enhance both nucleation and growth rates as predicted by CNT. However, the temperature effects on nucleation rates become less important at lower temperatures (below 268 K), consistent with CLOUD studies (Duplissy et al., 2016), which found that sulfuric acid nucleation takes place at the kinetic limit without a Gibbs free energy barrier when temperature was low. These results emphasize the importance of NH₃ and other ternary species at warmer temperatures, for example, especially in the conditions present in the boundary layer.

Our results demonstrate that clusters formed at lower temperatures, while being transported to warmer temperatures, can indeed survive evaporation and even grow further in the presence of SO₂ and ozone. We are providing the first experimental evidence that supports the mechanism of downward transport of newly formed particles from the free troposphere to the marine boundary layer as proposed by several field and modeling studies (Russell et al., 1994; Raes, 1995; Clarke, 1993). Similarly, based on our results, it is reasonable to conclude that the new particles formed in the free troposphere over the Amazon forest are transferred downward to the warmer surface to act as a reservoir of nuclei-mode particles (Wang et al., 2016), though the mechanism of growth is different from the one studied here (SO₂ + ozone).

Our results also show that the further growth is strongly dependent on the ozone level, implying that some unknown heterogeneous reaction processes involving SO₂ and ozone on sulfuric clusters may play important roles in NPF. These results can open a new research avenue for future studies to create a better understanding of the roles of heterogeneous reactions involving nanoparticles and the effects of SO₂ on the nanoparticle growth (Stangl et al., 2019). At present, it is not known why NPF takes place with high frequency and strong magnitude in extremely polluted megacities in China under the conditions with exceedingly high loadings of preexisting aerosol particles (Guo et al., 2014; Yao et al., 2018; Yu et al., 2017b; Lee et al., 2019). The heterogeneous reactions involving SO₂ in nanoparticles proposed here, if they can be repeated and further understood in future studies, may provide some key insights into understanding the frequent nucleation and fast growth observed in these regions, where there are also very high concentrations of SO₂ and ozone.